Nachtermin Teil B - lernen mit Serlo!

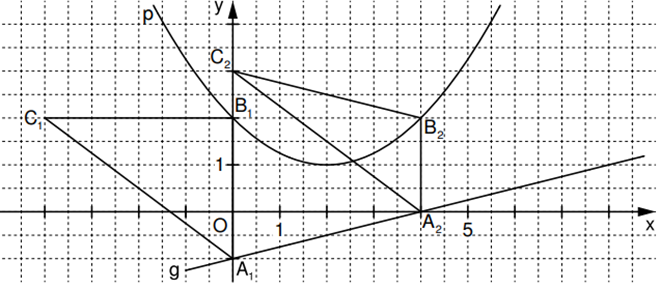
Aufgabe B2

Gegeben sind die Parabel $p$ mit der Gleichung $y = 0,25 x^{2} - x + 2 (x, y \in ℝ)$ und die

Gerade $g$ mit der Gleichung $y = 0,25 x - 1 (x, y \in ℝ)$ .

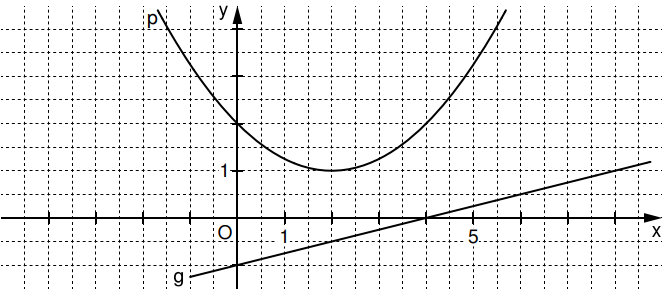
Punkte $A_{n} (x | 0,25 x - 1)$ auf der Geraden $g$ und Punkte $B_{n} (x | 0,25 x^{2} - x + 2)$ auf der
Parabel $p$ haben dieselbe Abszisse $x$ . Sie sind zusammen mit Punkten $C_{n}$ Eckpunkte von Dreiecken $A_{n} B_{n} C_{n}$ . Es gilt: $\vec{A_{n} C_{n}} = (\begin{array}{c} - 4 \\ 3 \end{array})$ .
Zeichnen Sie die Dreiecke $A_{1} B_{1} C_{1}$ für $x = 0$ und $A_{2} B_{2} C_{2}$ für $x = 4$ in das
Koordinatensystem der Aufgabenstellung ein. (2 P)

geg.: Parabel $p : y = 0,25 \cdot x^{2} - x + 2$ ; Gerade $g : y = 0,25 \cdot x - 1$
Punkte $A_{n} (x | 0,25 \cdot x - 1)$ auf g, Punkte $B_{n} (x | 0,25 \cdot x^{2} - x + 2)$ auf p mit derselben Abszisse $x$ ,
Punkte $C_{n}$ sind Eckpunkte von $△ A_{n} B_{n} C_{n}$ mit $\vec{A_{n} C_{n}} = (\begin{array}{c} - 4 \\ 3 \end{array})$
ges.: Einzeichnen der Dreiecke $A_{n} B_{n} C_{n}$ für $x = 0$ und $x = 4$ ins KOSY
Ansatz und Rechnung:
Setze $x = 0$ in $A$ und in $B$ ein:
$A_{1} (0 | - 1)$
$B_{1} (0 | 2)$
Berechne $C_{1}$ :
$\vec{A_{1} C_{1}} = (\begin{array}{c} - 4 \\ 3 \end{array}) = {\vec{C}}_{1} - {\vec{A}}_{1} \Rightarrow {\vec{C}}_{1} = {\vec{A}}_{1} + (\begin{array}{c} - 4 \\ 3 \end{array}) = (\begin{array}{c} 0 \\ - 1 \end{array}) + (\begin{array}{c} - 4 \\ 3 \end{array}) = (\begin{array}{c} - 4 \\ 2 \end{array})$
$\Rightarrow C_{1} (- 4 | 2)$
Setze $x = 4$ in $A$ und in $B$ ein:
$A_{2} (4 | 0)$
$B_{2} (4 | 2)$
Berechne $C_{2}$ :
${\vec{C}}_{2} = {\vec{A}}_{2} + (\begin{array}{c} - 4 \\ 3 \end{array}) = (\begin{array}{c} 4 \\ 0 \end{array}) + (\begin{array}{c} - 4 \\ 3 \end{array}) = (\begin{array}{c} 0 \\ 3 \end{array})$
Parabel $p$ mit Gerade $g$ und den Dreiecken $A_{1} B_{1} C_{1}$ für $x = 0$ und $A_{2} B_{2} C_{2}$ für $x = 4$
Hast du eine Frage oder Feedback?
Kommentiere hier 👇
Einzeichnen von Dreiecken mit Eckpunkten auf Parabel und Gerade
Zeigen Sie rechnerisch, dass für die Länge der Strecken $A_{n} B_{n}$ in Abhängigkeit von der Abszisse $x$ der Punkte $A_{n}$ gilt: $| A_{n} B_{n} | (x) = (0,25 x^{2} - 1,25 x + 3) LE$ . (1 P)

geg.: $A_{n} (x | 0,25 \cdot x - 1)$ und $B_{n} (x | 0,25 \cdot x^{2} - x + 2)$
ges.: $| \vec{A_{n} B_{n}} (x) |$
Ansatz und Rechnung:
1. Bestimmung des Vektors $\vec{A_{n} B_{n}} (x)$ aus den Punkten $A_{n} (x | 0,25 \cdot x - 1)$ und $B_{n} (x | 0,25 \cdot x^{2} - x + 2)$
$\vec{A_{n} B_{n}} (x) = (\begin{array}{c} x \\ 0,25 \cdot x^{2} - x + 2 \end{array}) - (\begin{array}{c} x \\ 0,25 \cdot x - 1 \end{array})$
$\vec{A_{n} B_{n}} (x) = (\begin{array}{c} x - x \\ 0,25 \cdot x^{2} - x + 2 - 0,25 \cdot x + 1 \end{array}) = (\begin{array}{c} 0 \\ 0,25 \cdot x^{2} - 1,25 \cdot x + 3 \end{array})$
Anmerkung:
Die $x -$ Komponenten von $A_{n} (x | 0,25 x - 1)$ und $B_{n} (x | 0,25 x^{2} - x + 2)$ sind identisch.
Somit kann auf die obige Vektordarstellung verzichtet werden und die Vektorlängenberechnung allein aus der Addition der $y -$ Komponenten erfolgen!
2. Berechnung der Vektorlänge $\vec{A_{n} B_{n}} (x)$ nach Pythagoras:
$| \vec{A_{n} B_{n}} | (x) = \sqrt{0^{2} + (0,25 \cdot x^{2} - 1,25 \cdot x + 3)^{2}}$
$| \vec{A_{n} B_{n}} | (x) = \sqrt{{(0,25 \cdot x^{2} - 1,25 \cdot x + 3)}^{2}}$ | Wurzel und Quadrat heben sich auf
$\Rightarrow | \vec{𝐀_{𝐧} 𝐁_{𝐧}} | (𝐱) = [𝟎,𝟐𝟓 \cdot 𝐱^{𝟐} - 𝟏,𝟐𝟓 \cdot 𝐱 + 𝟑] 𝐋 𝐄$
Hast du eine Frage oder Feedback?
Kommentiere hier 👇
Bestimmung von Vektor $\vec{A_{n} B_{n}} (x)$ und seiner Länge
Die Dreiecke $A_{3} B_{3} C_{3}$ und $A_{4} B_{4} C_{4}$ sind gleichschenklig mit der Basis $B_{3} C_{3}$ bzw. $B_{4} C_{4}$ . Berechnen Sie die zugehörigen Werte von $x$ . (3 P)
Runden Sie auf zwei Stellen nach dem Komma.

geg.: $\vec{A_{n} C_{n}} = (\begin{array}{c} - 4 \\ 3 \end{array})$ und $| \vec{A_{n} B_{n}} | (x) = [0,25 \cdot x^{2} - 1,25 \cdot x + 3] L E$
ges.: $| \vec{A_{n} C_{n}} |$ und $x$ -Werte der gleichschenkligen Dreiecke $A_{3} B_{3} C_{3}$ und $A_{4} B_{4} C_{4}$
Ansatz und Rechnung:
1. Berechnung der Länge von $\vec{A_{n} C_{n}} = (\begin{array}{c} - 4 \\ 3 \end{array})$ :
$\Rightarrow | \vec{A_{n} C_{n}} | = \sqrt{(- 4)^{2} + 3^{2}} = \sqrt{16 + 9} = \sqrt{25} = 5 L E$
2. Berechnung der $x -$ Werte der gleichschenkligen Dreiecke $A_{3} B_{3} C_{3}$ und $A_{4} B_{4} C_{4}$ :
In den gleichschenkligen Dreiecken mit der Basis $B_{3} C_{3}$ bzw. $B_{4} C_{4}$ sind die Beträge der Schenkel gleich, also gilt: $| \vec{A_{n} C_{n}} | = | \vec{A_{n} B_{n}} | (x)$
$\Rightarrow [0,25 \cdot x^{2} - 1,25 \cdot x + 3] L E = 5 L E$
Äquivalenzumformung und Erstellung der quadratischen Gleichung:
$[0,25 \cdot x^{2} - 1,25 \cdot x + 3] - 5 = 0$
$0,25 \cdot x^{2} - 1,25 \cdot x - 2 = 0$
$a$ - $b$ - $c$ -Formel anwenden: $x_{1,2} = \frac{- b \pm \sqrt{b^{2} - 4 a c}}{2 a}$
Diskriminante $D$ berechnen mit $a = 0,25$ ; $b = - 1,25$ ; $c = - 2$ ;
$D = b^{2} - 4 \cdot a \cdot c = (- 1,25)^{2} - 4 \cdot 0,25 \cdot (- 2) = 1,5625 + 2 = 3,5625$
$\Rightarrow D > 0$ . Also gibt es 2 Lösungen.
$D$ in $a$ - $b$ - $c$ -Formel einsetzen:
$x_{1,2} = \frac{- b \pm \sqrt{D}}{2 a} = \frac{- (- 1,25) \pm \sqrt{3,5625}}{2 \cdot 0,25} = \frac{1,25 \pm \sqrt{3,5625}}{0,5}$
$\Rightarrow x_{1} = \frac{1,25 + 1,887}{0,5} = 6,274$
$\Rightarrow x_{2} = \frac{1,25 - 1,887}{0,5} = - 1,274$
Die $x$ -Koordinaten sind somit $𝐱_{𝟏} = 𝟔,𝟐𝟕; 𝐱_{𝟐} = - 𝟏,𝟐𝟕$
Hast du eine Frage oder Feedback?
Kommentiere hier 👇
Berechnung der x-Werte für die gleichschenkligen Dreiecke mithilfe $| \vec{A_{n} C_{n}} |$ und $| \vec{A_{n} B_{n}} | (x)$

Dieses Werk wurde vom Bayerischen Staatsministerium für Unterricht und Kultus zur Verfügung gestellt. → Was bedeutet das?

Aufgabe B2

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?