Aufgaben zur linearen Unabhängigkeit
Hier findest du Aufgaben zur linearen Unabhängigkeit. Lerne, Vektoren auf ihre lineare Unabhängigkeit zu überprüfen und dessen Bedeutung!
- 1
Bestimme die Skalare, sodass der Vektor eine Linearkombination der Vektoren ist.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Linearkombination
Zu bestimmen sind die Skalare , sodass gilt
.
Setze die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen und .
.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Linearkombination
Zu bestimmen sind die Skalare , sodass gilt
Setze die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen und .
.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Linearkombination
Zu bestimmen sind die Skalare , sodass gilt
Setze die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen und .
.
Hast du eine Frage oder Feedback?
- 2
Prüfe, ob die Vektoren linear unabhängig sind.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare Unabhängigkeit
Die Vektoren und sind linear unabhängig, wenn du zwei Zahlen finden kannst, sodass und und nicht beide gleichzeitig sind.
Setz die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen und .
. Das bedeutet, das Gleichungssystem ist nur gelöst für und somit sind und linear unabhängig.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare Unabhängigkeit
Die Vektoren und sind linear unabhängig, wenn du zwei Zahlen finden kannst, sodass und und nicht beide gleichzeitig sind.
Setz die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen und .
Setze nun in ein.
↓ Setze ein.
↓ Vereinfache
Die Gleichung ist immer erfüllt. Also müssen und nur die Gleichung erfüllen. Für beispielsweise und ist die Gleichung also erfüllt und somit sind und linear abhängig.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare Unabhängigkeit
Die Vektoren , und sind linear unabhängig, wenn du drei Zahlen finden kannst, sodass
und , und gleichzeitig sind.
Setze die gegebenen Vektoren ein.
Es handelt sich um ein lineares Gleichungssystem mit den Variablen , und .
Löse das lineare Gleichungssystem zum Beispiel mit dem Additionsverfahren.
Rechne:
Rechne:
Setze in ein
Setze und in ein
Es ist also . Das bedeutet, das Gleichungssystem wird nur gelöst für und somit sind , und linear unabhängig.
Hast du eine Frage oder Feedback?
- 3
Prüfe rechnerisch, ob die drei Vektoren komplanar sind.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare (Un)abhängigkeit
Sind die Vektoren linear unabhängig, so kann man zum Beispiel den Vektor eindeutig durch und darstellen:
Daraus entsteht ein Gleichungssystem mit drei Gleichungen und zwei Unbekannten:
Die erste und die dritte Gleichung liefern unterschiedliche Lösungen für . Dadurch hat das Gleichungssystem keine eindeutige Lösung.
Die Vektoren sind also linear unabhängig und nicht komplanar.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare (Un)abhängigkeit
Sind die Vektoren linear unabhängig, so kann man zum Beispiel den Vektor eindeutig durch und darstellen:
Daraus entsteht ein Gleichungssystem mit drei Gleichungen und zwei Unbekannten:
Forme die erste Gleichung um:
Setze in II ein:
Und die erste Gleichung liefert
Da es sich um ein überbestimmtes System handelt, musst du zur Probe noch in die III. Gleichung einsetzen:
Die Gleichung III liefert eine wahre Aussage. Somit hat das System eine eindeutige Lösung und die drei Vektoren sind linear abhängig und somit komplanar.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Lineare (Un)abhängigkeit
Da die Vektoren und parallel sind, kann man nicht wie bei den letzten Aufgaben untersuchen.
Alternativ prüfst du
Daraus entsteht erneut ein Gleichungssystem mit drei Gleichungen und zwei Unbekannten:
Forme z.B. die erste Gleichung um:
Setze in die II. Gleichung ein:
Setze das Ergebnis in Gleichung I ein:
Setze in Gleichung III ein:
Das System hat also eine eindeutige Lösung und somit sind die drei Vektoren komplanar.
Hast du eine Frage oder Feedback?
- 4
Zeige, dass sich der Vektor auf unendlich viele Arten als Linearkombination der Vektoren und darstellen lässt und deute das Ergebnis geometrisch.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Linearkombination
Als Linearkombination darstellen bedeutet, dass du Zahlen finden musst, so dass:
Daraus entsteht ein Gleichungssystem mit zwei Gleichungen und zwei Unbekannten:
Wenn du die zweite Gleichung halbierst, erhältst du erneut die erste Gleichung:
Somit handelt es sich um ein unterbestimmtes System mit unendlich vielen Lösungen.
Forme die erste Gleichung um, um die Lösungsmenge in Abhängigkeit von anzugeben:
Für ergibt sich zum Beispiel .
Für ergibt sich folglich
geometrische Interpretation
Bei genauerem Hinsehen solltest du erkennen, dass alle drei Vektoren kollinear sind, also paarweise parallel. Sie liegen also auf einer gemeinsamen Geraden.
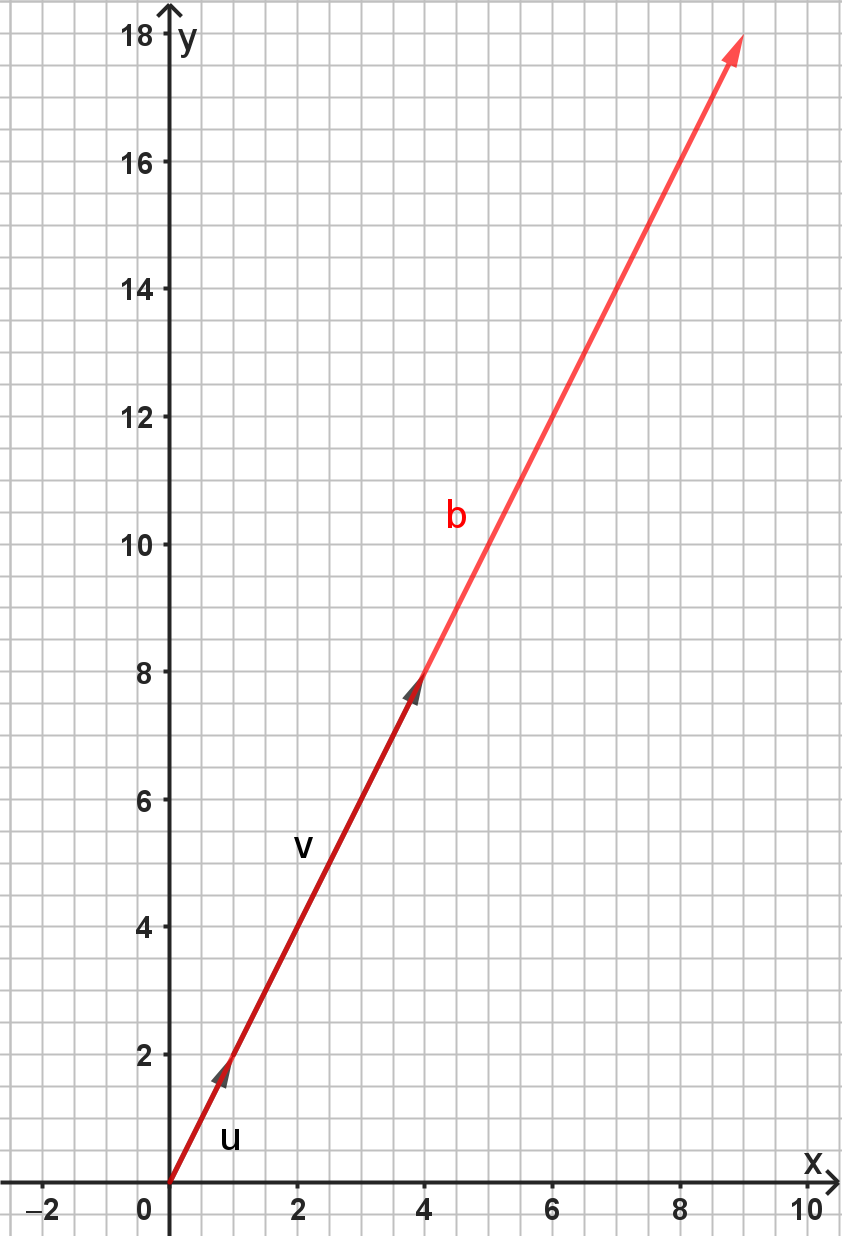
Einen Vektor als Linearkombination von und darzustellen, bedeutet, Zahlen zu finden, mit denen und multipliziert werden können, so dass der Vektor entsteht.
Zeige, dass es unendlich viele solche Zahlen gibt.
Dieses Werk steht unter der freien Lizenz
CC BY-SA 4.0 → Was bedeutet das?
