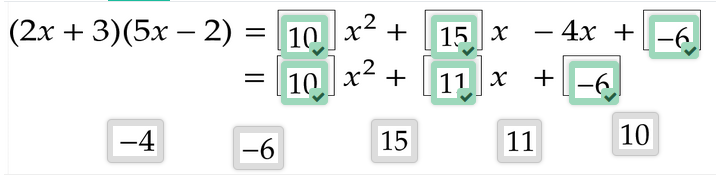Aufgaben zum Ausmultiplizieren

Hier findest du Übungsaufgaben zum Thema Ausmultiplizieren. Übe das Auflösen von Klammern, sowie Zusammenfassen der Terme!

1
Multipliziere aus

Für diese Aufgabe benötigst Du folgendes Grundwissen: Ausmultiplizieren
Richtige Zuordnung
Richtiges Ergebnis
Wähle den ersten Summanden in der ersten Klammer: $(2 x + 3)$
Multipliziere $2 x$ mit den Summanden der anderen Klammer.
Wähle den zweiten Summanden in der ersten Klammer: $(2 x + 3)$
Multipliziere $3$ mit den Summanden der anderen Klammer.
2
Löse auf
1. $a (c + d)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Distributivgesetz anwenden
  ↓
  $a (c + d)$ $=$ $a c + a d$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2. $4 (5 a + 3 b)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Distributivgesetz anwenden
  ↓
  $4 (5 a + 3 b)$ $=$ $4 \cdot 5 a + 4 \cdot 3 b$
  ↓
  Multiplikation
  $=$ $20 a + 12 b$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
3. $6 (3 x + 8 a)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Distributivgesetz anwenden
  ↓
  $6 (3 x + 8 a)$ $=$ $6 \cdot 3 x + 6 \cdot 8 a$
  ↓
  Multiplikation
  $=$ $18 x + 48 a$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
4. $10 (5 a + 10 c + 3 x)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Distributivgesetz anwenden
  ↓
  $10 (5 a + 10 c + 3 x)$ $=$ $10 \cdot 5 a + 10 \cdot 10 c + 10 \cdot 3 x$
  ↓
  Multiplikation
  $=$ $50 a + 100 c + 30 x$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
5. $4 (a + 2 b)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $4 (a + 2 b)$ $=$ $4 \cdot a + 4 \cdot 2 b$
  ↓
  Multipliziere.
  $=$ $4 a + 8 b$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
6. $6 (3 b + 17 a)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $6 (3 b + 17 a)$ $=$ $6 \cdot 3 b + 6 \cdot 17 a$
  ↓
  Multipliziere.
  $=$ $18 b + 102 a$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
7. $33 (5 a + 8 b)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $33 (5 a + 8 b)$ $=$ $33 \cdot 5 a + 33 \cdot 8 b$
  ↓
  Multipliziere
  $=$ $165 a + 264 b$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
8. $14 (4 a + 5 b)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $14 (4 a + 5 b)$ $=$ $14 \cdot 4 a + 14 \cdot 5 b$
  ↓
  Multipliziere
  $=$ $56 a + 70 b$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
9. $4 ac (ab + 3 cd)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $4 a c (a b + 3 c d)$ $=$ $4 a c \cdot a b + 4 a c \cdot 3 c d$
  ↓
  Multipliziere
  $=$ $4 a^{2} b c + 12 a c^{2} d$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
10. $(14 x - 15 a) (30 b + 18 c)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $(14 x - 15 a) (30 b + 18 c)$ $=$ $14 x \cdot 30 b + 14 x \cdot 18 c - 15 a \cdot 30 b - 15 a \cdot 18 c$
  ↓
  Multipliziere.
  $=$ $420 b x + 252 c x - 450 a b - 270 a c$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
11. $(7 a + 3 c) (4 b + 2 c)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $(7 a + 3 c) (4 b + 2 c)$ $=$ $7 a \cdot 4 b + 7 a \cdot 2 c + 3 c \cdot 4 b + 3 c \cdot 2 c$
  ↓
  Multipliziere.
  $=$ $28 a b + 14 a c + 12 b c + 6 c^{2}$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
12. $(3 x - 5 y) (- 3 xy + 2 y)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $(3 x - 5 y) (- 3 x y + 2 y)$ $=$ $3 x \cdot (- 3 x y) + 3 x \cdot 2 y + (- 5 y) \cdot (- 3 x y) + (- 5 y) \cdot 2 y$
  ↓
  Multipliziere
  $=$ $- 9 x^{2} y + 6 x y + 15 x y^{2} - 10 y^{2}$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
13. $7 ac (4 bc + 4 ac)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $7 a c (4 b c + 4 a c)$ $=$ $7 a c \cdot 4 b c + 7 a c \cdot 4 a c$
  ↓
  Multipliziere
  $=$ $28 a b c^{2} + 28 a^{2} c^{2}$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
14. $4 ab (7 bc + 7 c)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $4 a b (7 b c + 7 c)$ $=$ $4 a b \cdot 7 b c + 4 a b \cdot 7 c$
  ↓
  Multipliziere.
  $=$ $28 a b^{2} c + 28 a b c$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
15. $4 c (7 a + 7 b)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $4 c (7 a + 7 b)$ $=$ $4 c \cdot 7 a + 4 c \cdot 7 b$
  ↓
  Multipliziere
  $=$ $28 a c + 28 b c$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
16. $7 bc (4 c + 4 bc)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
  Wende das Distributivgesetz an.
  ↓
  $7 b c (4 c + 4 b c)$ $=$ $7 b c \cdot 4 c + 7 b c \cdot 4 b c$
  ↓
  Multipliziere
  $=$ $28 b c^{2} + 28 b^{2} c^{2}$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉

Multipliziere die Summen aus.

123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$x \cdot (m + n)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$x \cdot (m + n)$ $=$
↓
Multipliziere jeden Summanden mit x.
$=$ $m x + n x$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

$- 20 \cdot (- 5 u + 3 v + 3 v - 1,5 w)$

123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$2,5 \cdot (4 x + 2 y)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$2,5 \cdot (4 x + 2 y)$ $=$
↓
Multipliziere jeden Summanden mit 2,5.
$=$ $2,5 \cdot 4 x + 2,5 \cdot 2 y$
↓
Berechne die Produkte bilden unter Beachtung der Vorzeichen.
$=$ $10 x + 5 y$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$6 m \cdot (3 m - 1,5 n - 4 mn)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$6 m \cdot (3 m - 1,5 n - 4 mn)$ $=$
↓
Multipliziere jeden Summanden mit 6m.
$=$ $6 m \cdot 3 m - 6 m \cdot 1,5 n - 6 m \cdot 4 m n$
↓
Berechne die Produkte. Beachte die Vorzeichen!
$=$ $18 m^{2} - 9 mn - 24 m^{2} n$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$- 3 m \cdot (- m - n)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$- 3 m \cdot (- m - n)$ $=$
↓
Multipliziere jeden Summanden mit -3m.
$=$ $(- 3 m) \cdot (- m) + (- 3 m) \cdot (- n)$
↓
Berechne die Produkte. Beachte die Vorzeichen!
$=$ $3 m^{2} + 3 m n$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$\frac{3}{4} \cdot (\frac{9}{8} a - \frac{5}{6} b - \frac{1}{12} c)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$\frac{3}{4} \cdot (\frac{9}{8} a - \frac{5}{6} b - \frac{1}{12} c)$ $=$
↓
Multipliziere jeden Summanden mit $\frac{3}{4}$
$=$ $\frac{3}{4} \cdot \frac{9}{8} a - \frac{3}{4} \cdot \frac{5}{6} b - \frac{3}{4} \cdot \frac{1}{12} c$
↓
Berechne die Produkte.
$=$ $\frac{27}{32} a - \frac{15}{24} b - \frac{3}{48} c$
↓
Kürze den Bruch bei $b$ und $c$ mit 3.
$=$ $\frac{27}{32} a - \frac{5}{8} b - \frac{1}{16} c$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(x - 5) \cdot (x + \frac{3}{2})$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(x - 5) \cdot (x + \frac{3}{2})$ $=$
↓
Multipliziere die Klammern aus.
$=$ $x^{2} + \frac{3}{2} x - 5 x - 5 \cdot \frac{3}{2}$
↓
Fasse gleiche Variablen zusammen.
$=$ $x^{2} - \frac{7}{2} x - \frac{15}{2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(\frac{2}{3} x - 2) \cdot (x + 3)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(\frac{2}{3} x - 2) \cdot (x + 3)$ $=$
↓
Multipliziere die Klammern aus.
$=$ $\frac{2}{3} x^{2} + 3 \cdot \frac{2}{3} x - 2 x - 6$
↓
Berechne die Produkte.
$=$ $\frac{2}{3} x^{2} + 2 x - 2 x - 6$
↓
Fasse die gleichen Variablen zusammen.
$=$ $\frac{2}{3} x^{2} - 6$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(\frac{1}{2} x - \frac{5}{2}) \cdot (x + 5)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(\frac{1}{2} x - \frac{5}{2}) \cdot (x + 5)$ $=$
↓
Multipliziere die Klammern aus.
$=$ $\frac{1}{2} x^{2} + 5 \cdot \frac{1}{2} x - \frac{5}{2} x - 5 \cdot \frac{5}{2}$
↓
Berechne die Produkte.
$=$ $\frac{1}{2} x^{2} + \frac{5}{2} x - \frac{5}{2} x - \frac{25}{2}$
↓
Fasse die gleichen Variablen zusammen.
$=$ $\frac{1}{2} x^{2} - \frac{25}{2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$\frac{3}{2} \cdot (x + 4) \cdot (x + 4)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$\frac{3}{2} \cdot (x + 4) \cdot (x + 4)$ $=$
↓
Wende die erste binomische Formel an.
$=$ $\frac{3}{2} \cdot (x^{2} + 8 x + 16)$
↓
Löse die Klammer auf.
$=$ $\frac{3}{2} x^{2} + \frac{3}{2} \cdot 8 x + \frac{3}{2} \cdot 16$
↓
Berechne die Produkte.
$=$ $\frac{3}{2} x^{2} + 12 x + 24$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(3 - 2 x) \cdot (- 2 x + 3)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(3 - 2 x) \cdot (- 2 x + 3)$ $=$
↓
Multipliziere die Klammern aus.
$=$ $- 6 x + 9 + 4 x^{2} - 6 x$
↓
Fasse die gleichen Variablen zusammen.
$=$ $4 x^{2} - 12 x + 9$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

$\frac{x - 5}{1} \cdot (2 x + 8)$

123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(x + 8) \cdot (\frac{1}{4} x + 1)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(x + 8) \cdot (\frac{1}{4} x + 1)$ $=$
↓
Multipliziere die Klammern aus.
$=$ $\frac{1}{4} x^{2} + x + \frac{8}{4} x + 8$
↓
Kürze $\frac{8}{4}$ mit 4.
$=$ $\frac{1}{4} x^{2} + x + 2 x + 8$
↓
Fasse die gleichen Variablen zusammen.
$=$ $\frac{1}{4} x^{2} + 3 x + 8$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$(1 - \frac{1}{5} x) \cdot (\frac{2}{5} x + 2)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(1 - \frac{1}{5} x) \cdot (\frac{2}{5} x + 2)$ $=$
↓
Multipliziere die Klammern aus.
$=$ $\frac{2}{5} x + 2 - \frac{2}{25} x^{2} - \frac{2}{5} x$
↓
Fasse die gleichen Variablen zusammen.
$=$ $- \frac{2}{25} x^{2} + 2$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$\frac{x}{2} \cdot {(2 x - k)}^{2}$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$\frac{x}{2} \cdot {(2 x - k)}^{2}$ $=$
↓
Wende die zweite binomische Formel an.
$=$ $\frac{x}{2} \cdot (4 x^{2} - 4 kx + k^{2})$
↓
Multipliziere die Klammern aus.
$=$ $2 x^{3} - 2 {kx}^{2} + \frac{k^{2}}{2} x$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
123mathe.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Rudolf Brinkmann
$- \frac{1}{8} \cdot {(4 - 2 x)}^{2}$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$- \frac{1}{8} \cdot {(4 - 2 x)}^{2}$ $=$
↓
Wende die zweite binomische Formel an.
$=$ $- \frac{1}{8} \cdot (16 - 16 x + 4 x^{2})$
↓
Multipliziere die Klammer aus.
$=$ $- 2 + 2 x - \frac{1}{2} x^{2}$
↓
Sortiere nach Variablen.
$=$ $- \frac{1}{2} x^{2} + 2 x - 2$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

$x \cdot (x + 3) \cdot (2 x - 5)$

${(x - 1)}^{3}$

Multipliziere aus und fasse zusammen.

$(- 2 {ab}^{3}) \cdot (1,5 a^{2} b)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Terme ausmultiplizieren
Multipliziere die Klammern aus.
$(- 2 a b^{3}) \cdot (1,5 a^{2} b)$ $=$ $- 3 a^{3} b^{4}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$3 x [5 xy - (6 yx - 9 y^{2})] - 2 y (- 2 x^{2})$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammer ausmultiplizieren
$3 x [5 xy - (6 yx - 9 y^{2})] - 2 y (- 2 x^{2})$ $=$ $3 x (5 x y - 6 y x + 9 y^{2}) + 4 y x^{2}$
↓
Multipliziere die Klammern aus.
$=$ $15 x^{2} y - 18 y x^{2} + 27 x y^{2} + 4 y x^{2}$
↓
Fasse die Terme zusammen.
$=$ $1 x^{2} y + 27 x y^{2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

$10,5 a^{3} b^{4} c : (- 3 bc) - {(- 2)}^{2} {(2 ab)}^{3}$

$(1,5 u + v) (- 2 u + 5 v)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
Multipliziere die Klammern aus.
$(1,5 u + v) (- 2 u + 5 v)$ $=$ $- 3 u^{2} + 7,5 u v - 2 v u + 5 v^{2}$
↓
Subtrahiere. $u v = v u$
$=$ $- 3 u^{2} + 5,5 u v + 5 v^{2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$(x - 7) (x + 4) - x (- 2 x - 3)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
Multipliziere die Klammern aus
$(x - 7) (x + 4) - x (- 2 x - 3)$ $=$ $x^{2} + 4 x - 7 x - 28 + 2 x^{2} + 3 x$
↓
Addiere und Subtrahiere.
$=$ $3 x^{2} - 28$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$(- \frac{1}{2} a + 3 b) (1,25 + \frac{3}{4}) \cdot 3 a - 2 a (b - 1 \frac{1}{2} b)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(- \frac{1}{2} a + 3 b) (1,25 + \frac{3}{4}) \cdot 3 a - 2 a (b - 1 \frac{1}{2} b)$
Wir schreiben $- \frac{1}{2} a = - 0,5 a$ und fassen $1,25 + \frac{3}{4} = 1,25 + 0,75 = 2$ mit $3 a$ zu $6 a$ zusammen.
Außerdem rechnen wir $b - 1 \frac{1}{2} b = b - 1,5 b = - 0,5 b$ .
$= (- 0,5 a + 3 b) \cdot 6 a - 2 a \cdot (- 0,5 b)$
Jetzt multiplizieren wir die erste Klammer aus:
$- 0,5 a \cdot 6 a = (- 0,5 \cdot 6) \cdot a \cdot a = - 3 a^{2}$ und $3 b \cdot 6 a = 18 a b .$
Außerdem ist wegen $- 2 \cdot (- 0,5) = 1$ der Vorfaktor im letzten Summanden 1 und darf weggelassen werden.
$= - 3 a^{2} + 18 a b + a b$
$= - 3 a^{2} + 19 a b$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$(0,3 x - x + \frac{7}{10} x) (x + 4) (x^{2} - 3 x + 1) - 2 (x^{2} - 1)$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(0,3 x - x + \frac{7}{10} x) (x + 4) (x^{2} - 3 x + 1) - 2 (x^{2} - 1)$
$= (0,3 x^{2} - x^{2} + \frac{7}{10} x^{2} + 1,2 x - 4 x + \frac{14}{5} x) (x^{2} - 3 x + 1) - 2 x^{2} + 2$
$= (0,3 x^{2} - x^{2} + \frac{7}{10} x^{2} + 1,2 x - 4 x + \frac{14}{5} x) (x^{2} - 3 x + 1) - 2 x^{2} + 2$
$= 0 (x^{2} - 3 x + 1) - 2 x^{2} + 2$
$= - 2 x^{2} + 2$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$(0,5 x - 1) (- y + \frac{2}{3} x) - \frac{1}{3} (x - 2) x$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$(0,5 x - 1) (- y + \frac{2}{3} x) - \frac{1}{3} (x - 2) x$
$= - 0,5 x y + \frac{1}{3} x^{2} + y - \frac{2}{3} x - \frac{1}{3} x^{2} + \frac{2}{3} x$
$= - 0,5 x y + y$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$2 (\frac{1}{2} a \cdot 3 b) + 3 b [0,25 b - (- 2 a)]$

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern ausmultiplizieren
$2 (\frac{1}{2} a \cdot 3 b) + 3 b [0,25 b - (- 2 a)]$
$= 3 a b + 3 b (0,25 b + 2 a)$
$= 3 a b + 0,75 b^{2} + 6 a b$
$= 9 a b + 0,75 b^{2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

5
Überlege, aus wie vielen Summanden die Summe besteht, die man nach dem Ausmultiplizieren des Terms $(a^{2} + a + 1) (b^{2} - b^{5} + b^{11} - 1) (c^{3} - 1)$ erhält.
Summanden

Für diese Aufgabe benötigst Du folgendes Grundwissen: Klammern auflösen
Man muss die Anzahl der Elemente in den einzelnen Klammern zählen.
$(3) (4) (2)$
Da man beim Auflösen von Klammern immer jedes Element einer Klammer jeweils mit den Elementen der anderen Klammer(n) multipliziert, muss man um die Anzahl der Summanden zu erhalten, die Anzahl der Elemente in den einzelnen Klammern miteinander multiplizieren.
$3 \cdot 4 \cdot 2 = 24$
$\Rightarrow$ Die Summe besteht aus 24 Elementen.
6
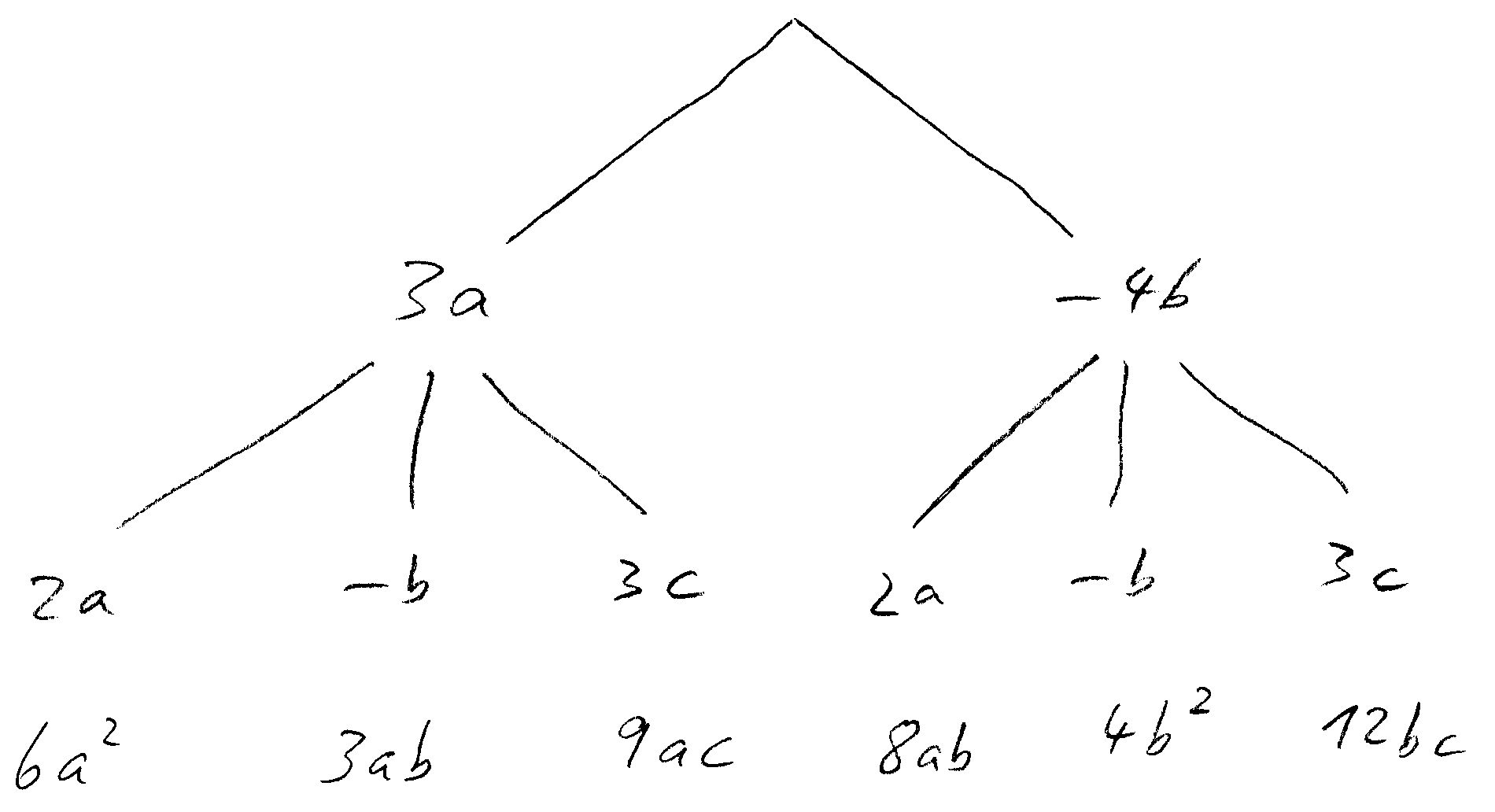
Benutze das Hilfsmittel des Baum-Schemas, um die folgenden Klammern auszumultiplizieren:
$(3 a - 4 b) \cdot (2 a - b + 3 c)$

Und nun liest du von oben nach unten die Produkte ab, die du beim Ausmultiplizieren erhältst und schreibst sie unter den Baum. Die Vorzeichen berücksichtigst du erst einmal nicht!
Erst wenn du die Summe der Produkte bildest, fügst du jeweils vor ein Produkt ein Minus- oder ein Pluszeichen ein, je nachdem, ob du auf dem Weg zu diesem Produkt einer ungeraden oder einer geraden Anzahl von Minuszeichen begegnest.
Auf dem Weg zu $4 b^{2}$ begegnest du beispielsweise 2 Minuszeichen, also kommt vor $4 b^{2}$ ein Pluszeichen (Minus mal Minus gibt Plus).
Damit erhältst du das Ergebnis
$6 a^{2} - 3 a b + 9 a c - 8 a b + 4 b^{2} - 12 b c$
Dies ist das Ergebnis des Ausmultiplizierens der beiden Klammern!
Wenn du noch gleiche Terme zusammenfassen willst, erhältst du
$6 a^{2} - 11 a b + 9 a c + 4 b^{2} - 12 b c$
Du zeichnest einen Baum mit zwei Ebenen. Die erste Ebene beschriftest du mit den Summanden der ersten Klammer, die zweite Ebene mit den Summanden der zweiten Klammer.

Dieses Werk steht unter der freien Lizenz
CC BY-SA 4.0 → Was bedeutet das?

Distributivgesetz anwenden
	↓
$4 (5 a + 3 b)$	$=$	$4 \cdot 5 a + 4 \cdot 3 b$
	↓	Multiplikation
	$=$	$20 a + 12 b$

Distributivgesetz anwenden
	↓
$6 (3 x + 8 a)$	$=$	$6 \cdot 3 x + 6 \cdot 8 a$
	↓	Multiplikation
	$=$	$18 x + 48 a$

Distributivgesetz anwenden
	↓
$10 (5 a + 10 c + 3 x)$	$=$	$10 \cdot 5 a + 10 \cdot 10 c + 10 \cdot 3 x$
	↓	Multiplikation
	$=$	$50 a + 100 c + 30 x$

Wende das Distributivgesetz an.
	↓
$4 (a + 2 b)$	$=$	$4 \cdot a + 4 \cdot 2 b$
	↓	Multipliziere.
	$=$	$4 a + 8 b$

Wende das Distributivgesetz an.
	↓
$6 (3 b + 17 a)$	$=$	$6 \cdot 3 b + 6 \cdot 17 a$
	↓	Multipliziere.
	$=$	$18 b + 102 a$

Wende das Distributivgesetz an.
	↓
$33 (5 a + 8 b)$	$=$	$33 \cdot 5 a + 33 \cdot 8 b$
	↓	Multipliziere
	$=$	$165 a + 264 b$

Wende das Distributivgesetz an.
	↓
$14 (4 a + 5 b)$	$=$	$14 \cdot 4 a + 14 \cdot 5 b$
	↓	Multipliziere
	$=$	$56 a + 70 b$

Wende das Distributivgesetz an.
	↓
$4 a c (a b + 3 c d)$	$=$	$4 a c \cdot a b + 4 a c \cdot 3 c d$
	↓	Multipliziere
	$=$	$4 a^{2} b c + 12 a c^{2} d$

Wende das Distributivgesetz an.
	↓
$(14 x - 15 a) (30 b + 18 c)$	$=$	$14 x \cdot 30 b + 14 x \cdot 18 c - 15 a \cdot 30 b - 15 a \cdot 18 c$
	↓	Multipliziere.
	$=$	$420 b x + 252 c x - 450 a b - 270 a c$

Wende das Distributivgesetz an.
	↓
$(7 a + 3 c) (4 b + 2 c)$	$=$	$7 a \cdot 4 b + 7 a \cdot 2 c + 3 c \cdot 4 b + 3 c \cdot 2 c$
	↓	Multipliziere.
	$=$	$28 a b + 14 a c + 12 b c + 6 c^{2}$

$2,5 \cdot (4 x + 2 y)$	$=$
	↓	Multipliziere jeden Summanden mit 2,5.
	$=$	$2,5 \cdot 4 x + 2,5 \cdot 2 y$
	↓	Berechne die Produkte bilden unter Beachtung der Vorzeichen.
	$=$	$10 x + 5 y$

$- 3 m \cdot (- m - n)$	$=$
	↓	Multipliziere jeden Summanden mit -3m.
	$=$	$(- 3 m) \cdot (- m) + (- 3 m) \cdot (- n)$
	↓	Berechne die Produkte. Beachte die Vorzeichen!
	$=$	$3 m^{2} + 3 m n$

$\frac{3}{4} \cdot (\frac{9}{8} a - \frac{5}{6} b - \frac{1}{12} c)$	$=$
	↓	Multipliziere jeden Summanden mit $\frac{3}{4}$
	$=$	$\frac{3}{4} \cdot \frac{9}{8} a - \frac{3}{4} \cdot \frac{5}{6} b - \frac{3}{4} \cdot \frac{1}{12} c$
	↓	Berechne die Produkte.
	$=$	$\frac{27}{32} a - \frac{15}{24} b - \frac{3}{48} c$
	↓	Kürze den Bruch bei $b$ und $c$ mit 3.
	$=$	$\frac{27}{32} a - \frac{5}{8} b - \frac{1}{16} c$

$(x - 5) \cdot (x + \frac{3}{2})$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$x^{2} + \frac{3}{2} x - 5 x - 5 \cdot \frac{3}{2}$
	↓	Fasse gleiche Variablen zusammen.
	$=$	$x^{2} - \frac{7}{2} x - \frac{15}{2}$

Wende das Distributivgesetz an.
	↓
$(3 x - 5 y) (- 3 x y + 2 y)$	$=$	$3 x \cdot (- 3 x y) + 3 x \cdot 2 y + (- 5 y) \cdot (- 3 x y) + (- 5 y) \cdot 2 y$
	↓	Multipliziere
	$=$	$- 9 x^{2} y + 6 x y + 15 x y^{2} - 10 y^{2}$

Wende das Distributivgesetz an.
	↓
$7 a c (4 b c + 4 a c)$	$=$	$7 a c \cdot 4 b c + 7 a c \cdot 4 a c$
	↓	Multipliziere
	$=$	$28 a b c^{2} + 28 a^{2} c^{2}$

Wende das Distributivgesetz an.
	↓
$4 a b (7 b c + 7 c)$	$=$	$4 a b \cdot 7 b c + 4 a b \cdot 7 c$
	↓	Multipliziere.
	$=$	$28 a b^{2} c + 28 a b c$

Wende das Distributivgesetz an.
	↓
$4 c (7 a + 7 b)$	$=$	$4 c \cdot 7 a + 4 c \cdot 7 b$
	↓	Multipliziere
	$=$	$28 a c + 28 b c$

Wende das Distributivgesetz an.
	↓
$7 b c (4 c + 4 b c)$	$=$	$7 b c \cdot 4 c + 7 b c \cdot 4 b c$
	↓	Multipliziere
	$=$	$28 b c^{2} + 28 b^{2} c^{2}$

$x \cdot (m + n)$	$=$
	↓	Multipliziere jeden Summanden mit x.
	$=$	$m x + n x$

$- 20 \cdot (- 5 u + 3 v + 3 v - 1,5 w)$	$=$
	↓	Fasse zusammen.
	$=$	$- 20 \cdot (- 5 u + 6 v - 1,5 w)$
	↓	Multipliziere jeden Summanden mit -20.
	$=$	$(- 20) \cdot (- 5 u) + (- 20) \cdot 6 v + (- 20) \cdot (- 1,5 w)$
	↓	Berechne die Produkte unter Beachtung der Vorzeichen.
	$=$	$100 u - 120 v + 30 w$

$6 m \cdot (3 m - 1,5 n - 4 mn)$	$=$
	↓	Multipliziere jeden Summanden mit 6m.
	$=$	$6 m \cdot 3 m - 6 m \cdot 1,5 n - 6 m \cdot 4 m n$
	↓	Berechne die Produkte. Beachte die Vorzeichen!
	$=$	$18 m^{2} - 9 mn - 24 m^{2} n$

$(\frac{2}{3} x - 2) \cdot (x + 3)$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$\frac{2}{3} x^{2} + 3 \cdot \frac{2}{3} x - 2 x - 6$
	↓	Berechne die Produkte.
	$=$	$\frac{2}{3} x^{2} + 2 x - 2 x - 6$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$\frac{2}{3} x^{2} - 6$

$(\frac{1}{2} x - \frac{5}{2}) \cdot (x + 5)$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$\frac{1}{2} x^{2} + 5 \cdot \frac{1}{2} x - \frac{5}{2} x - 5 \cdot \frac{5}{2}$
	↓	Berechne die Produkte.
	$=$	$\frac{1}{2} x^{2} + \frac{5}{2} x - \frac{5}{2} x - \frac{25}{2}$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$\frac{1}{2} x^{2} - \frac{25}{2}$

Aufgaben zum Ausmultiplizieren

Multipliziere aus

Richtige Zuordnung

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

$\frac{3}{2} \cdot (x + 4) \cdot (x + 4)$	$=$
	↓	Wende die erste binomische Formel an.
	$=$	$\frac{3}{2} \cdot (x^{2} + 8 x + 16)$
	↓	Löse die Klammer auf.
	$=$	$\frac{3}{2} x^{2} + \frac{3}{2} \cdot 8 x + \frac{3}{2} \cdot 16$
	↓	Berechne die Produkte.
	$=$	$\frac{3}{2} x^{2} + 12 x + 24$

$(3 - 2 x) \cdot (- 2 x + 3)$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$- 6 x + 9 + 4 x^{2} - 6 x$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$4 x^{2} - 12 x + 9$

$\frac{x - 5}{1} \cdot (2 x + 8)$	$=$
	↓	Multipliziere.
	$=$	$\frac{(x - 5) \cdot (2 x + 8)}{1}$
	↓	Multipliziere die Klammer im Zähler aus. Den Bruchstrich kannst du jetzt weglassen, da durch 1 geteilt wird.
	$=$	$2 x^{2} + 8 x - 10 x - 40$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$2 x^{2} - 2 x - 40$

$(x + 8) \cdot (\frac{1}{4} x + 1)$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$\frac{1}{4} x^{2} + x + \frac{8}{4} x + 8$
	↓	Kürze $\frac{8}{4}$ mit 4.
	$=$	$\frac{1}{4} x^{2} + x + 2 x + 8$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$\frac{1}{4} x^{2} + 3 x + 8$

$(1 - \frac{1}{5} x) \cdot (\frac{2}{5} x + 2)$	$=$
	↓	Multipliziere die Klammern aus.
	$=$	$\frac{2}{5} x + 2 - \frac{2}{25} x^{2} - \frac{2}{5} x$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$- \frac{2}{25} x^{2} + 2$

$\frac{x}{2} \cdot {(2 x - k)}^{2}$	$=$
	↓	Wende die zweite binomische Formel an.
	$=$	$\frac{x}{2} \cdot (4 x^{2} - 4 kx + k^{2})$
	↓	Multipliziere die Klammern aus.
	$=$	$2 x^{3} - 2 {kx}^{2} + \frac{k^{2}}{2} x$

$- \frac{1}{8} \cdot {(4 - 2 x)}^{2}$	$=$
	↓	Wende die zweite binomische Formel an.
	$=$	$- \frac{1}{8} \cdot (16 - 16 x + 4 x^{2})$
	↓	Multipliziere die Klammer aus.
	$=$	$- 2 + 2 x - \frac{1}{2} x^{2}$
	↓	Sortiere nach Variablen.
	$=$	$- \frac{1}{2} x^{2} + 2 x - 2$

$x \cdot (x + 3) \cdot (2 x - 5)$	$=$
	↓	Fasse die beiden Klammern zusammen.
	$=$	$x \cdot (2 x^{2} - 5 x + 6 x - 15)$
	↓	Fasse in der Klammer die gleichen Variablen zusammen.
	$=$	$x \cdot (2 x^{2} + x - 15)$
	↓	Multipliziere die Klammern aus.
	$=$	$2 x^{3} + x^{2} - 15 x$

${(x - 1)}^{3}$	$=$
	↓	Zerlege in zwei Produkte.
	$=$	$(x - 1) \cdot {(x - 1)}^{2}$
	↓	Wende im zweiten Faktor die zweite binomische Formel an.
	$=$	$(x - 1) \cdot (x^{2} - 2 x + 1)$
	↓	Löse die Klammern auf.
	$=$	$x^{3} - 2 x^{2} + x - x^{2} + 2 x - 1$
	↓	Fasse die gleichen Variablen zusammen.
	$=$	$x^{3} - 3 x^{2} + 3 x - 1$

$3 x [5 xy - (6 yx - 9 y^{2})] - 2 y (- 2 x^{2})$	$=$	$3 x (5 x y - 6 y x + 9 y^{2}) + 4 y x^{2}$
	↓	Multipliziere die Klammern aus.
	$=$	$15 x^{2} y - 18 y x^{2} + 27 x y^{2} + 4 y x^{2}$
	↓	Fasse die Terme zusammen.
	$=$	$1 x^{2} y + 27 x y^{2}$