Aufgaben zum Aufstellen von Ebenengleichungen

1
raschweb.de (Gilt für: Aufgabenstellung)Dieses Werk steht unter der freien Lizenz CC BY-SA 4.0 mit Namensnennung von Herrn Günther Rasch
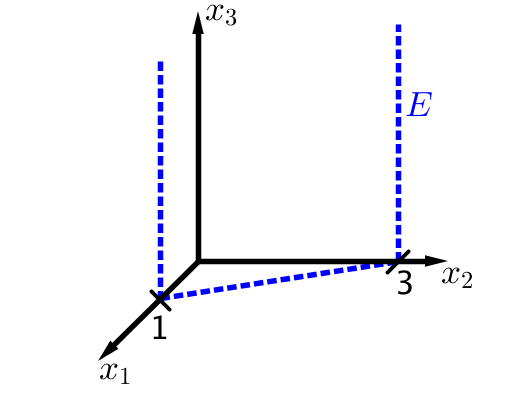
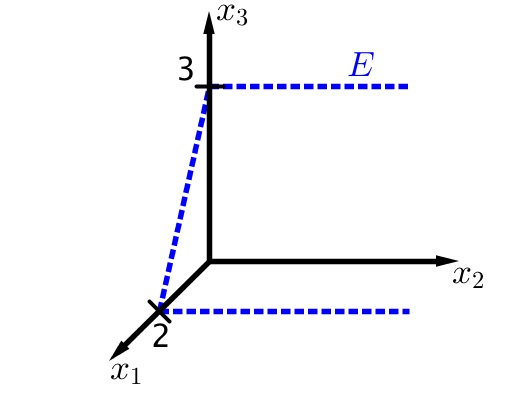
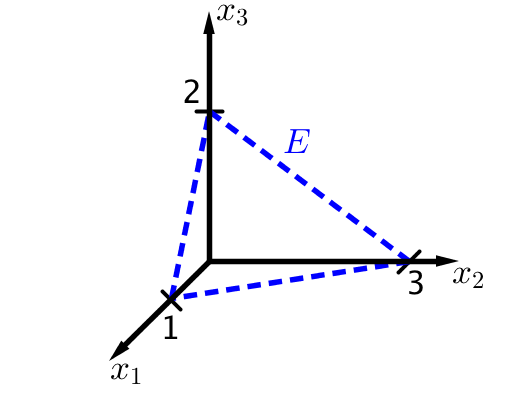
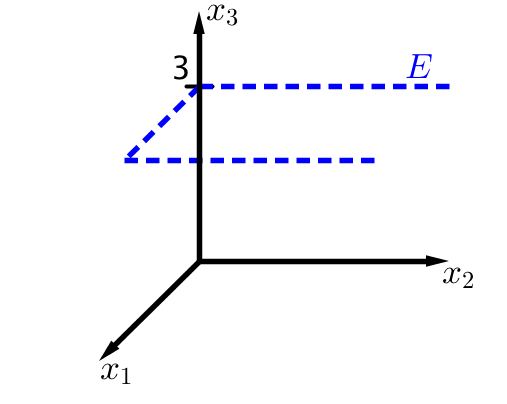
In den folgenden Bildern ist je eine Ebene E dargestellt. Stelle die dargestellte Ebene in Parameterform auf.
1. Aus der Zeichnung kannst du zwei Punkt ablesen, die in der Ebene liegen:
  $A (1; 0; 0)$
  $B (0; 3; 0)$
  Der Vektor $\vec{AB}$ ist einer der Richtungsvektoren der Ebene $E$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 0 \\ 3 \\ 0 \end{array}) - (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} - 1 \\ 3 \\ 0 \end{array})$
  Der zweite Richtungsvektor ist parallel zur $x_{3} - Achse$ . Wir können also als zweiten Richtungsvektor $(\begin{array}{c} 0 \\ 0 \\ 1 \end{array})$ nehmen.
  Wähle den Punkt $A$ oder $B$ als Aufpunkt und stelle die Gleichung der Ebene $E$ in Parameter auf.
  $E : \vec{x} = (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 3 \\ 0 \end{array}) + μ \cdot (\begin{array}{c} 0 \\ 0 \\ 1 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2. Ebene in Parameterform aufstellen
  Lese die gegebenen Punkte ab:
  $A (2; 0; 0)$ , $B (0; 0; 3)$
  Der Vektor $\vec{AB}$ ist einer der Richtungsvektoren der Ebene $E$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 0 \\ 0 \\ 3 \end{array}) - (\begin{array}{c} 2 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} - 2 \\ 0 \\ 3 \end{array})$
  Der zweite Richtungsvektor $\vec{v}$ ist parallel zur $x_{2} - Achse$ .
  $\Rightarrow \vec{v} = (\begin{array}{c} 0 \\ 1 \\ 0 \end{array})$
  Wähle den Punkt $A$ oder $B$ als Aufpunkt und Stelle die Gleichung der Ebene $E$ auf.
  $E : \vec{x} = (\begin{array}{c} 2 \\ 0 \\ 0 \end{array}) + λ \cdot (\begin{array}{c} - 2 \\ 0 \\ 3 \end{array}) + μ \cdot (\begin{array}{c} 0 \\ 1 \\ 0 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
3. Ebene in Parameterform aufstellen
  Lese die gegebenen Punkte ab:
  $A (1; 0; 0)$ , $B (0; 3; 0)$ , $C (0; 0; 2)$
  Der Vektor $\vec{AB}$ und $\vec{AC}$ sind die Richtungsvektoren der Ebene $E$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 0 \\ 3 \\ 0 \end{array}) - (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} - 1 \\ 3 \\ 0 \end{array})$
  $\vec{A C} = \vec{C} - \vec{A} = (\begin{array}{c} 0 \\ 0 \\ 2 \end{array}) - (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} - 1 \\ 0 \\ 2 \end{array})$
  Wähle einen der Punkte $A$ , $B$ oder $C$ als Aufpunkt und Stelle die Gleichung der Ebene $E$ auf.
  $E : \vec{x} = (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 3 \\ 0 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ 0 \\ 2 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
4. Ebene in Parameterform aufstellen
  Lese die gegebenen Punkte ab:
  $A (0; 0; 3)$
  Einer der Richtungsvektoren der Ebene $E$ ist parallel zur $x_{1} - Achse$ .
  $\Rightarrow \vec{v} = (\begin{array}{c} 1 \\ 0 \\ 0 \end{array})$
  Der andere Richtungsvektor ist parallel zur $x_{2} - Achse$ .
  $\Rightarrow \vec{u} = (\begin{array}{c} 0 \\ 2 \\ 0 \end{array})$
  Wähle den Punkt $A$ als Aufpunkt und Stelle die Gleichung Ebene $E$ auf.
  $E : \vec{x} = (\begin{array}{c} 0 \\ 0 \\ 3 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ 0 \\ 0 \end{array}) + μ \cdot (\begin{array}{c} 0 \\ 1 \\ 0 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2
Gegeben sind die parallelen Geraden
$g : \vec{x} = (\begin{array}{c} 2 \\ - 1 \\ 1 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ - 2 \\ 1 \end{array})$
und
$h : \vec{x} = (\begin{array}{c} 1 \\ 3 \\ - 1 \end{array}) + μ \cdot (\begin{array}{c} - 2 \\ 4 \\ - 2 \end{array})$
Bestimme die Gleichung der Ebene $E$ in Parameterform, in der die beiden Geraden $g$ und $h$ liegen.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene aus zwei Geraden bestimmen
Wähle den Vektor zwischen den Aufpunkten $A_{g}$ und $A_{h}$ der
beiden Geraden als ersten Richtungsvektor der Ebene.
Berechne $\vec{A_{g} A_{h}}$ .
$\begin{array}{l} \vec{A_{g} A_{h}} \\ = \vec{A_{h}} - \vec{A_{g}} \\ = (\begin{array}{c} 1 \\ 3 \\ - 1 \end{array}) - (\begin{array}{c} 2 \\ - 1 \\ 1 \end{array}) \\ = (\begin{array}{c} - 1 \\ 4 \\ - 2 \end{array}) \end{array}$
Wähle einen der Aufpunkte der beiden Geraden, z.b. $A_{g}$ ,
als Aufpunkt der Ebene.
Wähle einen der Richtungsvektoren der beiden Geraden
z.B. $\vec{v_{g}}$ , als zweiten Richtungsvektor der Ebene.
Stelle nun die Ebenengleichung auf.
$E : \vec{x} = (\begin{array}{c} 2 \\ - 1 \\ 1 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 4 \\ - 2 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ - 2 \\ 1 \end{array})$
3
Gegeben ist die Gerade $g : \vec{x} = (\begin{array}{c} 2 \\ 1 \\ - 3 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 3 \\ 1 \end{array})$ und der Punkt $P (1; - 3; - 3)$ , der nicht auf der Geraden liegt.
Bestimme die Gleichung der Ebene $E$ in Parameterform, in der der Punkt $P$ und die Gerade $g$ liegen.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene aus einem Punkt und einer Geraden aufstellen
Wähle den Richtungsvektor der Geraden $\vec{u} = (\begin{array}{c} - 1 \\ 3 \\ 1 \end{array})$ als ersten Richtungsvektor und den Vektor zwischen dem Punkt $P$ und dem Aufpunkt $A$ der Geraden als zweiten Richtungsvektor der Ebene $E$ .
Berechne $\vec{PA}$ .
$\vec{PA} = \vec{A} - \vec{P} = (\begin{array}{c} 2 \\ 1 \\ - 3 \end{array}) - (\begin{array}{c} 1 \\ - 3 \\ - 3 \end{array}) = (\begin{array}{c} 1 \\ 4 \\ 0 \end{array})$
Wähle $P$ als Aufpunkt der Ebene $E$ .
$\Rightarrow E : \vec{x} = (\begin{array}{c} 1 \\ - 3 \\ - 3 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 3 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ 4 \\ 0 \end{array})$
4
Gegeben sind die zueinander windschiefen Geraden $g : \vec{x} = (\begin{array}{c} 1 \\ - 3 \\ 2 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ 2 \\ - 3 \end{array})$ und $h : \vec{x} = (\begin{array}{c} 14 \\ 4 \\ 3 \end{array}) + μ \cdot (\begin{array}{c} 2 \\ - 3 \\ 0 \end{array})$ . Bestimme die Gleichung der Ebene $E$ in Parameterform, in der die Gerade $g$ liegt und zu der die Gerade $h$ parallel ist.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene
Ebene in Parameterform aufstellen
Wähle den Aufpunkt der Geraden $g$ als Aufpunkt und die beiden Richtungsvektoren $\vec{u} = (\begin{array}{c} 1 \\ 2 \\ - 3 \end{array})$ und $\vec{v} = (\begin{array}{c} 2 \\ - 3 \\ 0 \end{array})$ der Geraden als Richtungsvektor der Ebene $E$ .
Die Gleichung der Ebene lautet dann : $E : \vec{x} = (\begin{array}{c} 1 \\ - 3 \\ 2 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ 2 \\ - 3 \end{array}) + μ \cdot (\begin{array}{c} 2 \\ - 3 \\ 0 \end{array})$
5
Stelle aus den folgenden drei Punkten eine Ebenengleichung in Parameterform auf.
1. $A (1; 0; 3)$ , $B (0; 2; 1)$ , $C (2; 2; 4)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 0 \\ 2 \\ 1 \end{array}) - (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) = (\begin{array}{c} - 1 \\ 2 \\ - 2 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} 2 \\ 2 \\ 4 \end{array}) - (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) = (\begin{array}{c} 1 \\ 2 \\ 1 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 2 \\ - 2 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ 2 \\ 1 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2. $A (0; 1; 2)$ , $B (3, 3; 3)$ , $C (- 1; 1; 4)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 3 \\ 3 \\ 3 \end{array}) - (\begin{array}{c} 0 \\ 1 \\ 2 \end{array}) = (\begin{array}{c} 3 \\ 2 \\ 1 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} - 1 \\ 1 \\ 4 \end{array}) - (\begin{array}{c} 0 \\ 1 \\ 2 \end{array}) = (\begin{array}{c} - 1 \\ 0 \\ 2 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 0 \\ 1 \\ 2 \end{array}) + λ \cdot (\begin{array}{c} 3 \\ 2 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ 0 \\ 2 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
3. $A (0; 0; 0)$ , $B (1; 0; 3)$ , $C (- 1; 2; 0)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) - (\begin{array}{c} 0 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} 1 \\ 0 \\ 3 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} - 1 \\ 2 \\ 0 \end{array}) - (\begin{array}{c} 0 \\ 0 \\ 0 \end{array}) = (\begin{array}{c} - 1 \\ 2 \\ 0 \end{array})$
  Wähle $\vec{OA} = \vec{O}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{O} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 0 \\ 0 \\ 0 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ 2 \\ 0 \end{array})$
  Den Ortsvektor $\vec{O} = (\begin{array}{c} 0 \\ 0 \\ 0 \end{array})$ kann man auch weglassen.
  $E : \vec{x} = λ \cdot (\begin{array}{c} 1 \\ 0 \\ 3 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ 2 \\ 0 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
4. $A (1; 1; - 1)$ , $B (1; 2; 1)$ , $C (0; 3; 1)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 1 \\ 2 \\ 1 \end{array}) - (\begin{array}{c} 1 \\ 1 \\ - 1 \end{array}) = (\begin{array}{c} 0 \\ 1 \\ 2 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} 0 \\ 3 \\ 1 \end{array}) - (\begin{array}{c} 1 \\ 1 \\ - 1 \end{array}) = (\begin{array}{c} - 1 \\ 2 \\ 2 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 1 \\ 1 \\ - 1 \end{array}) + λ \cdot (\begin{array}{c} 0 \\ 1 \\ 2 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ 2 \\ 2 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
5. $A (3; 1; 2)$ , $B (2; 3; 1)$ , $C (4; 3; 3)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 2 \\ 3 \\ 1 \end{array}) - (\begin{array}{c} 3 \\ 1 \\ 2 \end{array}) = (\begin{array}{c} - 1 \\ 2 \\ - 1 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} 4 \\ 3 \\ 3 \end{array}) - (\begin{array}{c} 3 \\ 1 \\ 2 \end{array}) = (\begin{array}{c} 1 \\ 2 \\ 1 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 3 \\ 1 \\ 2 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 2 \\ - 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ 2 \\ 1 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
6. $A (2; 2; 2)$ , $B (5; 2; 1)$ , $C (3; 2; 4)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 5 \\ 2 \\ 1 \end{array}) - (\begin{array}{c} 2 \\ 2 \\ 2 \end{array}) = (\begin{array}{c} 3 \\ 0 \\ - 1 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} 3 \\ 2 \\ 4 \end{array}) - (\begin{array}{c} 2 \\ 2 \\ 2 \end{array}) = (\begin{array}{c} 1 \\ 0 \\ 2 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 2 \\ 2 \\ 2 \end{array}) + λ \cdot (\begin{array}{c} 3 \\ 0 \\ - 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ 0 \\ 2 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
7. $A (40; 80; 0)$ , $B (20; 60; 10)$ , $C (55; 90; 20)$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Berechne zuerst die Richtungsvektoren $\vec{AB}$ und $\vec{AC}$ :
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 20 \\ 60 \\ 10 \end{array}) - (\begin{array}{c} 40 \\ 80 \\ 0 \end{array}) = (\begin{array}{c} - 20 \\ - 20 \\ 10 \end{array})$
  Da sich die Richtung eines Vektors durch Multiplikation mit einer reellen Zahl nicht ändert, kann man $10$ ausklammern, um einen möglichst einfachen Vektor zu erhalten.
  $\Rightarrow \vec{AB} = (\begin{array}{c} - 2 \\ - 2 \\ 1 \end{array})$
  $\vec{AC} = \vec{C} - \vec{A} = (\begin{array}{c} 55 \\ 90 \\ 20 \end{array}) - (\begin{array}{c} 40 \\ 80 \\ 0 \end{array}) = (\begin{array}{c} 15 \\ 10 \\ 20 \end{array})$
  Auch hier kann man $5$ ausklammern, um einen möglichst einfachen Vektor zu erhalten.
  $\Rightarrow \vec{AC} = (\begin{array}{c} 3 \\ 2 \\ 4 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{AB} + μ \cdot \vec{AC}$ ein.
  $E : \vec{x} = (\begin{array}{c} 40 \\ 80 \\ 0 \end{array}) + λ \cdot (\begin{array}{c} - 2 \\ - 2 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} 3 \\ 2 \\ 4 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
6
Stelle aus zwei Punkten und einem Richtungsvektor eine Ebenengleichung in Parameterform auf.
1. $A (1; 3; - 2)$ , $B (3; 7; 5)$ , $\vec{v} = (\begin{array}{c} - 2 \\ 10 \\ - 7 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene
  Wähle $\vec{v}$ als ersten Richtungsvektor und $\vec{AB}$ als zweiten Richtungsvektor der Ebene.
  Berechne $\vec{AB}$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 3 \\ 7 \\ 5 \end{array}) - (\begin{array}{c} 1 \\ 3 \\ - 2 \end{array}) = (\begin{array}{c} 2 \\ 4 \\ 7 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{v} + μ \cdot \vec{AB}$ ein.
  $E : \vec{x} = (\begin{array}{c} 1 \\ 3 \\ - 2 \end{array}) + λ \cdot (\begin{array}{c} - 2 \\ 10 \\ - 7 \end{array}) + μ \cdot (\begin{array}{c} 2 \\ 4 \\ 7 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2. $A (2; 1; - 3)$ , $B (1; - 3; - 3)$ , $\vec{v} = (\begin{array}{c} - 1 \\ 3 \\ 1 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene
  Wähle $\vec{v}$ als ersten Richtungsvektor und $\vec{AB}$ als zweiten Richtungsvektor der Ebene.
  Berechne $\vec{AB}$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 1 \\ - 3 \\ - 3 \end{array}) - (\begin{array}{c} 2 \\ 1 \\ - 3 \end{array}) = (\begin{array}{c} - 1 \\ - 4 \\ 0 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{v} + μ \cdot \vec{AB}$ ein.
  $E : \vec{x} = (\begin{array}{c} 2 \\ 1 \\ - 3 \end{array}) + λ \cdot (\begin{array}{c} - 1 \\ 3 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} - 1 \\ - 4 \\ 0 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
3. $A (8; 13; 9)$ , $B (4; - 3; - 1)$ , $\vec{v} = (\begin{array}{c} - 6 \\ 1 \\ 1 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene
  Wähle $\vec{v}$ als ersten Richtungsvektor und $\vec{AB}$ als
  zweiten Richtungsvektor der Ebene.
  Berechne $\vec{AB}$ .
  $\vec{AB} = \vec{B} - \vec{A} = (\begin{array}{c} 4 \\ - 3 \\ - 1 \end{array}) - (\begin{array}{c} 8 \\ 13 \\ 9 \end{array}) = (\begin{array}{c} - 4 \\ - 16 \\ - 10 \end{array})$
  Um einen einfacheren Vektor zu erhalten, kann man gegebenenfalls noch $- 2$ ausklammern.
  $\Rightarrow \vec{AB} = (\begin{array}{c} 2 \\ 8 \\ 5 \end{array})$
  Wähle $\vec{OA}$ als Aufpunkt der Ebene und setze in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{v} + μ \cdot \vec{AB}$ ein.
  $E : \vec{x} = (\begin{array}{c} 8 \\ 13 \\ 9 \end{array}) + λ \cdot (\begin{array}{c} - 6 \\ 1 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} 2 \\ 8 \\ 5 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
7
Stelle aus einem Punkt und zwei Richtungsvektoren eine Ebenengleichung in Parameterform auf.
1. $A (1; 3; - 2)$ , $\vec{u} = (\begin{array}{c} - 2 \\ 10 \\ - 7 \end{array})$ , $\vec{v} = (\begin{array}{c} 1 \\ - 2 \\ 6 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Wähle $\vec{OA}$ als Aufpunkt und $\vec{u}$ und $\vec{v}$ als Richtungsvektoren der Ebene und setze sie in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{u} + μ \cdot \vec{v}$ ein:
  $E : \vec{x} = (\begin{array}{c} 1 \\ 3 \\ - 2 \end{array}) + λ \cdot (\begin{array}{c} - 2 \\ 10 \\ - 7 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ - 2 \\ 6 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
2. $A (1; 1; 1)$ , $\vec{u} = (\begin{array}{c} 1 \\ 1 \\ 1 \end{array})$ , $\vec{v} = (\begin{array}{c} 1 \\ 1 \\ - 1 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Parameterform aufstellen
  Wähle $\vec{OA}$ als Aufpunkt und $\vec{u}$ und $\vec{v}$ als Richtungsvektoren der Ebene und setze sie in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{u} + μ \cdot \vec{v}$ ein:
  $E : \vec{x} = (\begin{array}{c} 1 \\ 1 \\ 1 \end{array}) + λ \cdot (\begin{array}{c} 1 \\ 1 \\ 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ 1 \\ - 1 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
3. $A (5; 5; - 23)$ , $\vec{u} = (\begin{array}{c} 6 \\ 1 \\ - 17 \end{array})$ , $\vec{v} = (\begin{array}{c} 1,5 \\ - 2 \\ 8 \end{array})$
  
  Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene in Paramterform aufstellen
  Wähle $\vec{OA}$ als Aufpunkt und $\vec{u}$ und $\vec{v}$ als Richtungsvektoren der Ebene und setze sie in die Ebenengleichung $E : \vec{x} = \vec{OA} + λ \cdot \vec{u} + μ \cdot \vec{v}$ ein:
  $E : \vec{x} = (\begin{array}{c} 5 \\ 5 \\ - 23 \end{array}) + λ \cdot (\begin{array}{c} 6 \\ 1 \\ - 17 \end{array}) + μ \cdot (\begin{array}{c} 1,5 \\ - 2 \\ 8 \end{array})$
  Hast du eine Frage oder Feedback?
  Kommentiere hier 👉
8
Gegeben sind die Geraden $g : \vec{x} = (\begin{array}{c} 2 \\ 2 \\ - 3 \end{array}) + λ \cdot (\begin{array}{c} 2 \\ 1 \\ - 1 \end{array})$ und $h : \vec{x} = (\begin{array}{c} 3 \\ 0 \\ - 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ - 2 \\ 2 \end{array})$ , die sich im Punkt $S (2; 2; - 3)$ schneiden. Bestimme die Ebene $E$ in Parameterform, in der beide Geraden liegen.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Ebene von Koordinatenform in Parameterform umwandeln
Ebene in Parameterform aufstellen
Wähle den gemeinsamen Schnittpunkt $S$ der Geraden als Aufpunkt und die beiden Richtungsvektoren $\vec{u} = (\begin{array}{c} 2 \\ 1 \\ - 1 \end{array})$ und $\vec{v} = (\begin{array}{c} 1 \\ - 2 \\ 2 \end{array})$ der Geraden als Richtungsvektoren der Ebene $E$ .
Die Gleichung der Ebene $E$ lautet dann: $E : \vec{x} = (\begin{array}{c} 2 \\ 2 \\ - 3 \end{array}) + λ \cdot (\begin{array}{c} 2 \\ 1 \\ - 1 \end{array}) + μ \cdot (\begin{array}{c} 1 \\ - 2 \\ 2 \end{array})$
Veranschaulichung
Ziehe die Grafik mit der rechten Maustaste, um sie zu drehen.

Dieses Werk steht unter der freien Lizenz
CC BY-SA 4.0 → Was bedeutet das?

Aufgaben zum Aufstellen von Ebenengleichungen

Hast du eine Frage oder Feedback?

Ebene in Parameterform aufstellen

Hast du eine Frage oder Feedback?

Ebene in Parameterform aufstellen

Hast du eine Frage oder Feedback?

Ebene in Parameterform aufstellen

Hast du eine Frage oder Feedback?

Ebene in Parameterform aufstellen

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Ebene in Parameterform aufstellen

Veranschaulichung