Aufgaben zur Flächenberechnung

1

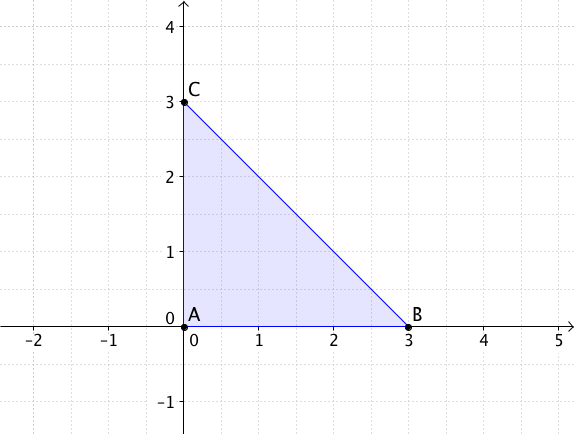
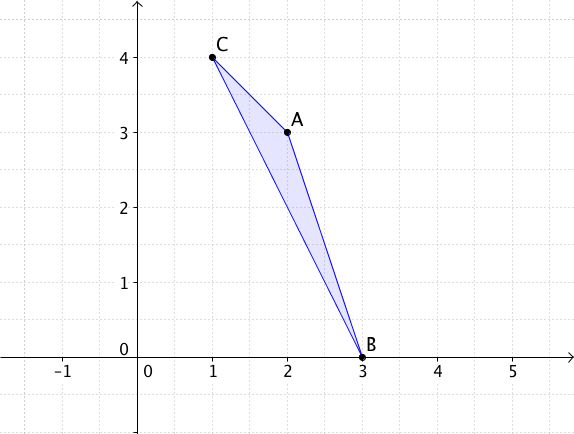
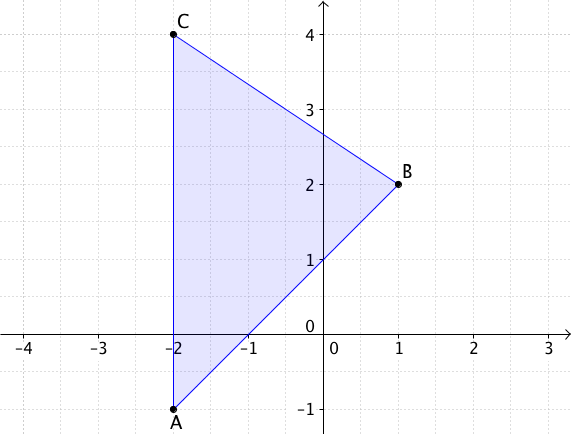
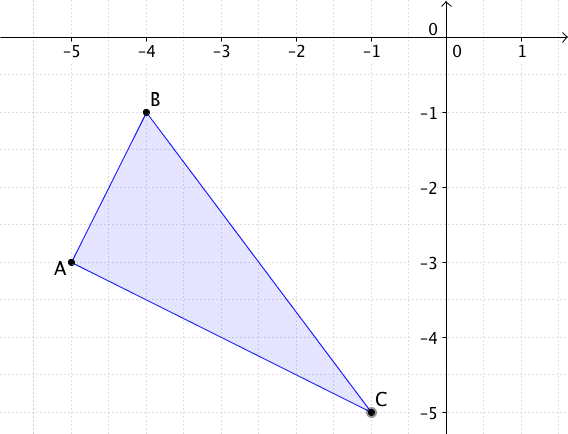
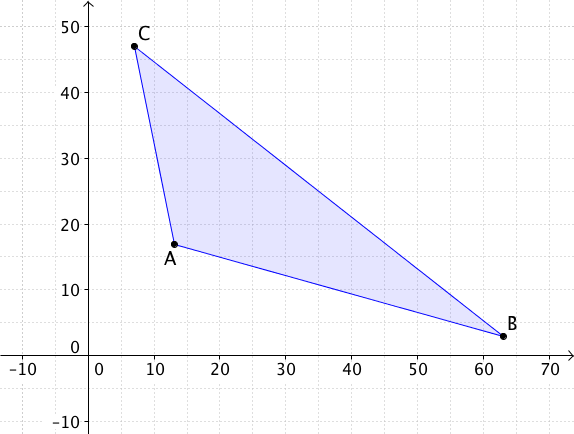
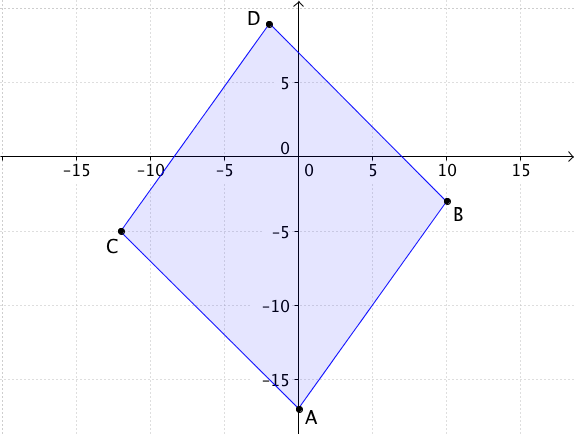
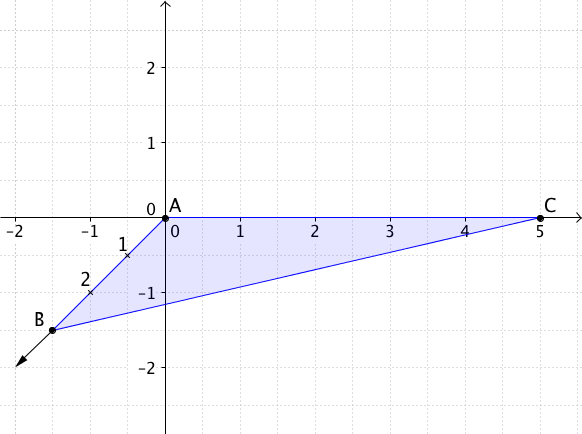
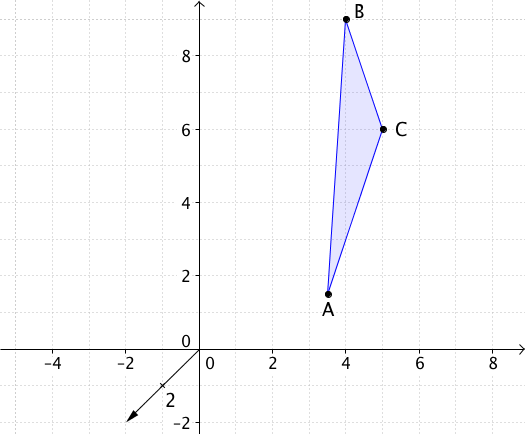
Berechne den Flächeninhalt des Dreiecks, das

durch die Punkte $\mathrm A\left(0\;\left|\;0\right.\right)$ , $\mathrm B\left(3\;\left|\;0\right.\right)$ , $\mathrm C\left(0\;\left|\;3\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}3\\0\end{pmatrix}-\begin{pmatrix}0\\0\end{pmatrix}=\begin{pmatrix}3\\0\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}0\\3\end{pmatrix}-\begin{pmatrix}0\\0\end{pmatrix}=\begin{pmatrix}0\\3\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Zweidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
Benutze die Eigenschaften der Determinante einer Diagonalmatrix .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\begin{pmatrix}3&0\\0&3\end{pmatrix}\right|$
$=\frac12\cdot\left|9\right|=4{,}5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(2\;\left|\;3\right.\right)$ , $\mathrm B\left(3\;\left|\;0\right.\right)$ , $\mathrm C\left(1\;\left|\;4\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}3\\0\end{pmatrix}-\begin{pmatrix}2\\3\end{pmatrix}=\begin{pmatrix}1\\-3\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}1\\4\end{pmatrix}-\begin{pmatrix}2\\3\end{pmatrix}=\begin{pmatrix}-1\\1\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Zweidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\begin{pmatrix}1&-1\\-3&1\end{pmatrix}\right|$
$=\frac12\cdot\left|\left(1-3\right)\right|=\frac12\cdot\left|-2\right|=1$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-2\;\left|\;-1\right.\right)$ , $\mathrm B\left(1\;\left|\;2\right.\right)$ , $\mathrm C\left(-2\;\left|\;4\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}1\\2\end{pmatrix}-\begin{pmatrix}-2\\-1\end{pmatrix}=\begin{pmatrix}3\\3\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-2\\4\end{pmatrix}-\begin{pmatrix}-2\\-1\end{pmatrix}=\begin{pmatrix}0\\5\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Zweidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
Benutze die Eigenschaften der Determinante einer unteren Dreiecksmatrix .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\begin{pmatrix}3&0\\3&5\end{pmatrix}\right|$
$=\frac12\cdot\left|15\right|=7{,}5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-5\;\left|\;-3\right.\right)$ , $\mathrm B\left(-4\;\left|\;-1\right.\right)$ , $\mathrm C\left(-1\;\left|\;-5\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}-4\\-1\end{pmatrix}-\begin{pmatrix}-5\\-3\end{pmatrix}=\begin{pmatrix}1\\2\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-1\\-5\end{pmatrix}-\begin{pmatrix}-5\\-3\end{pmatrix}=\begin{pmatrix}4\\-2\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Zweidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\begin{pmatrix}1&4\\2&-2\end{pmatrix}\right|$
$=\frac12\cdot\left|-2-8\right|=\frac12\cdot\left|-10\right|=5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(13\;\left|\;17\right.\right)$ , $\mathrm B\left(63\;\left|\;3\right.\right)$ , $\mathrm C\left(7\;\left|\;47\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}63\\3\end{pmatrix}-\begin{pmatrix}13\\17\end{pmatrix}=\begin{pmatrix}50\\-14\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}7\\47\end{pmatrix}-\begin{pmatrix}13\\17\end{pmatrix}=\begin{pmatrix}-6\\30\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Zweidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\det\begin{pmatrix}50&-6\\-14&30\end{pmatrix}\right|$
$=\frac12\cdot\left|\left(1500-84\right)\right|=\frac12\cdot\left|1416\right|=708$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

2

Bestimme den Flächeninhalt des Dreiecks $\,\triangle ABC$ mithilfe der Determinante

$A (3 ∣ 1)$ , $B (0 ∣ 2)$ und $C (4 ∣ 5)$
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Determinante
Um den Flächeninhalt $F$ bestimmen zu können, brauchen wir erst die Vektoren $\overrightarrow{AB}$ und $\overrightarrow{AC}$ .
$\overrightarrow{AB} = B - A = \begin{pmatrix}0 - 3\\2-1\end{pmatrix} = \begin{pmatrix}-3\\1\end{pmatrix}$
$\overrightarrow{AC} = C - A = \begin{pmatrix}4 - 3\\5-1\end{pmatrix} = \begin{pmatrix}1\\4\end{pmatrix}$
Nun setzt du die Vektoren in die Determinante ein, so dass für den Flächeninhalt $F$ gilt:
Beachte dabei, dass die Reihenfolge der Vektoren, die man in die Determinante einsetzt, gegen dem Uhrzeigersinn ist!
$F = \frac 1 2 \cdot \left|\overrightarrow{AC} \, \overrightarrow{AB} \right|$
$\phantom{F}=\frac 1 2 \cdot \left|\begin{pmatrix}1\\4\end{pmatrix} \begin{pmatrix}-3\\1\end{pmatrix} \right|$
$\phantom{F}=\frac 1 2 \begin{vmatrix} 1& -3\\ 4& 1\end{vmatrix} =\frac 1 2 ( 1 \cdot 1 - (-3) \cdot 4) = 6{,}5$
Für den Flächeninhalt des Dreiecks $\,\triangle ABC$ gilt also $F = 6,5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$A (- 4 ∣ - 5)$ , $B (- 1 ∣ 1)$ und $C (3 ∣ - 2)$
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Determinante
Um den Flächeninhalt $F$ bestimmen zu können, brauchen wir erst die Vektoren $\overrightarrow{AB}$ und $\overrightarrow{AC}$ .
$\overrightarrow{AB} = B - A = \begin{pmatrix}-1 - (-4)\\1-(-5)\end{pmatrix} = \begin{pmatrix}3\\6\end{pmatrix}$
$\overrightarrow{AC} = C - A = \begin{pmatrix}3 - (-4)\\-2-(-5)\end{pmatrix} = \begin{pmatrix}7\\3\end{pmatrix}$
Nun setzt du die Vektoren in die Determinante ein, so dass für den Flächeninhalt $F$ gilt:
Beachte dabei, dass die Reihenfolge der Vektoren, die man in die Determinante einsetzt, gegen dem Uhrzeigersinn ist!
$F = \frac 1 2 \cdot \left|\overrightarrow{AC} \, \overrightarrow{AB} \right|$
$\phantom{F}=\frac 1 2 \cdot \left|\begin{pmatrix}7\\3\end{pmatrix} \begin{pmatrix}3\\6\end{pmatrix} \right|$
$\phantom{F}=\frac 1 2 \begin{vmatrix} 7& 3\\ 3& 6\end{vmatrix} =\frac 1 2 ( 7 \cdot 6 - 3 \cdot 3) = 16{,}5$
Für den Flächeninhalt des Dreiecks $\,\triangle ABC$ gilt also $F = 16,5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$A (4 ∣ 4,5)$ , $B(2{,}5|-\frac{2}{3})$ und $C (- 3,2 ∣ - 2)$
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Determinante
Um den Flächeninhalt $F$ bestimmen zu können, brauchen wir erst die Vektoren $\overrightarrow{AB}$ und $\overrightarrow{AC}$ .
$\overrightarrow{AB} = B - A = \begin{pmatrix}2{,}5 - 4\\ -\frac{2}{3} -4{,}5\end{pmatrix} = \begin{pmatrix}-1{,}5\\-\frac{31}{6}\end{pmatrix}$
$\overrightarrow{AC} = C - A = \begin{pmatrix}-3{,}2 - 4\\-2-4{,}5\end{pmatrix} = \begin{pmatrix}-7{,}2\\-6{,}5\end{pmatrix}$
Nun setzt du die Vektoren in die Determinante ein, so dass für den Flächeninhalt $F$ gilt:
Beachte dabei, dass die Reihenfolge der Vektoren, die man in die Determinante einsetzt, gegen dem Uhrzeigersinn ist!
$F = \frac 1 2 \cdot \left|\overrightarrow{AC} \, \overrightarrow{AB} \right|$
$\phantom{F}=\frac 1 2 \cdot \left| \begin{pmatrix}-7{,}2\\-6{,}5\end{pmatrix} \begin{pmatrix}-1{,}5\\-\frac{31}{6}\end{pmatrix} \right|$
$\phantom{F}=\frac 1 2 \begin{vmatrix} -7{,}2& -1{,}5 \\ -6{,}5& -\frac{31}{6} \end{vmatrix}$
$\phantom{F}=\frac 1 2 \left[ (-7{,}2) \cdot \left(-\frac{31}{6}\right) - ( -1{,}5) \cdot (-6{,}5)\right]$
$\phantom{F}=13{,}725$
Für den Flächeninhalt des Dreiecks $\,\triangle ABC$ gilt also $F = 13,725$ .
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

3

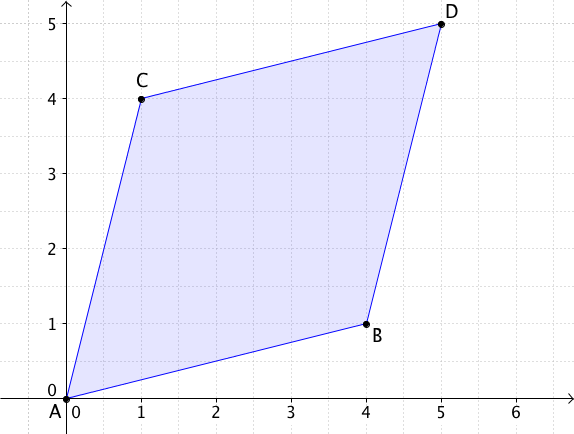
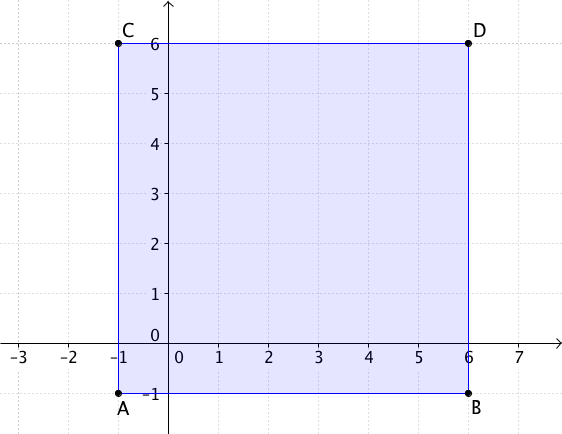
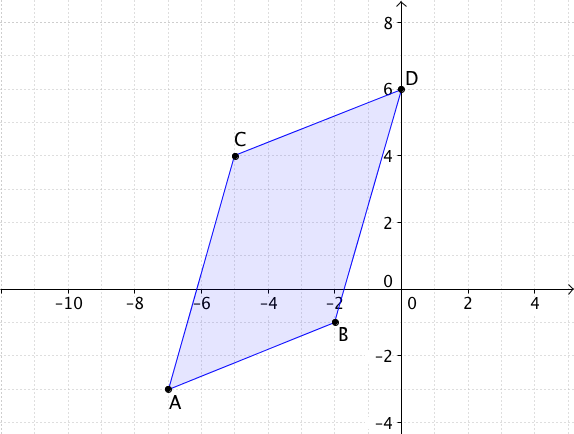
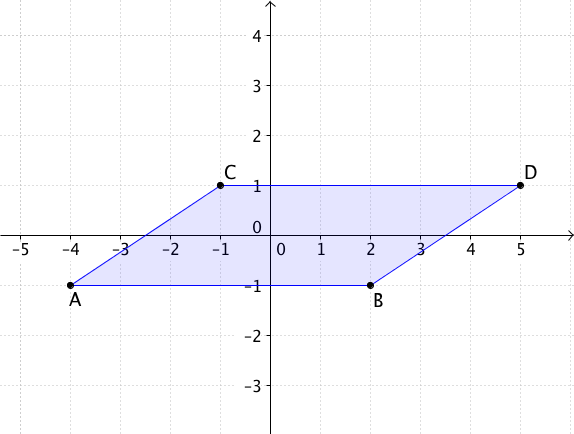
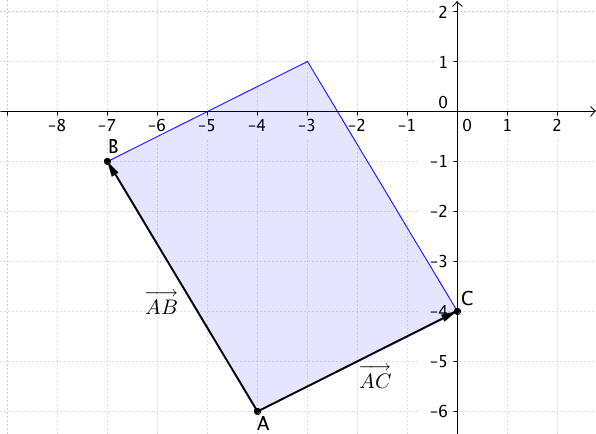
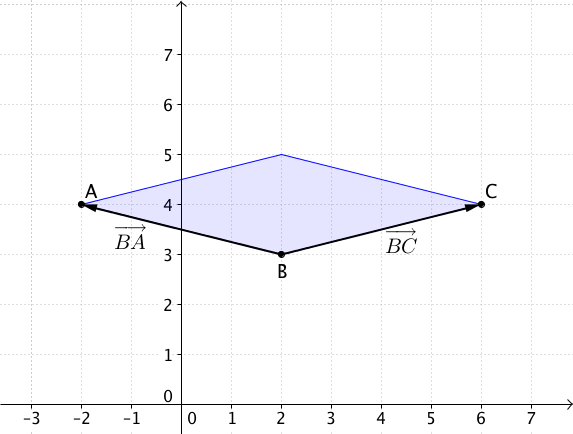
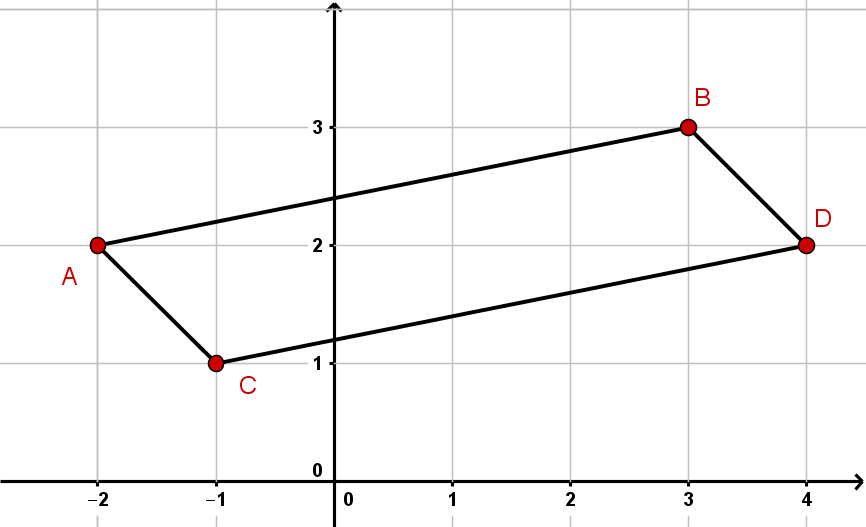
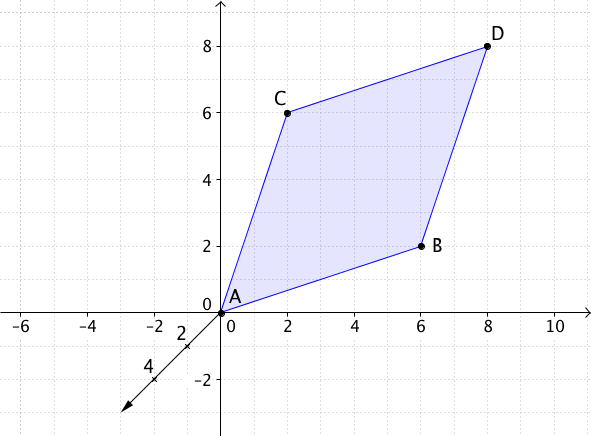
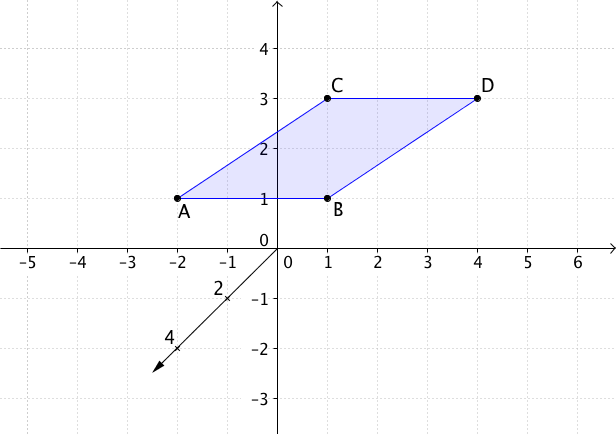
Berechne den Flächeninhalt des Parallelogramms, das

durch die Punkte $\mathrm A\left(0\;\left|\;0\right.\right)$ , $\mathrm B\left(4\;\left|\;1\right.\right)$ , $\mathrm C\left(1\;\left|\;4\right.\right)$ , $\mathrm D\left(5\;\left|\;5\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}4\\1\end{pmatrix}-\begin{pmatrix}0\\0\end{pmatrix}=\begin{pmatrix}4\\1\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}1\\4\end{pmatrix}-\begin{pmatrix}0\\0\end{pmatrix}=\begin{pmatrix}1\\4\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}4&1\\1&4\end{pmatrix}\right|$
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
$=\left|16-1\right|=\left|15\right|=15$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-1\;\left|\;-1\right.\right)$ , $\mathrm B\left(6\;\left|\;-1\right.\right)$ , $\mathrm C\left(-1\;\left|\;6\right.\right)$ , $\mathrm D\left(6\;\left|\;6\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}6\\-1\end{pmatrix}-\begin{pmatrix}-1\\-1\end{pmatrix}=\begin{pmatrix}7\\0\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-1\\6\end{pmatrix}-\begin{pmatrix}-1\\-1\end{pmatrix}=\begin{pmatrix}0\\7\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}7&0\\0&7\end{pmatrix}\right|$
Benutze die Eigenschaften der Determinante einer Diagonalmatrix .
$=\left|7\cdot7\right|=\left|49\right|=49$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-7\;\left|\;-3\right.\right)$ , $\mathrm B\left(-2\;\left|\;1\right.\right)$ , $\mathrm C\left(-5\;\left|\;4\right.\right)$ , $\mathrm D\left(0\;\left|\;6\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}-2\\1\end{pmatrix}-\begin{pmatrix}-7\\-3\end{pmatrix}=\begin{pmatrix}5\\2\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-5\\4\end{pmatrix}-\begin{pmatrix}-7\\-3\end{pmatrix}=\begin{pmatrix}2\\7\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}5&2\\2&7\end{pmatrix}\right|$
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
$=\left|35-4\right|=\left|31\right|=31$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-4\;\left|\;-1\right.\right)$ , $\mathrm B\left(2\;\left|\;-1\right.\right)$ , $\mathrm C\left(-1\;\left|\;1\right.\right)$ , $\mathrm D\left(5\;\left|\;1\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\def\arraystretch{1.25} \begin{array}{l}\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}2\\-1\end{pmatrix}-\begin{pmatrix}-4\\-1\end{pmatrix}=\begin{pmatrix}6\\0\end{pmatrix}\end{array}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-1\\1\end{pmatrix}-\begin{pmatrix}-4\\-1\end{pmatrix}=\begin{pmatrix}3\\2\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}6&3\\0&2\end{pmatrix}\right|$
Benutze die Eigenschaften der Determinante einer unteren Dreiecksmatrix .
$=\left|12\right|=12$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(0\;\left|\;-17\right.\right)$ , $\mathrm B\left(10\;\left|\;-3\right.\right)$ , $\mathrm C\left(-12\;\left|\;-5\right.\right)$ , $\mathrm D\left(-2\;\left|\;9\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}10\\-3\end{pmatrix}-\begin{pmatrix}0\\-17\end{pmatrix}=\begin{pmatrix}10\\14\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-12\\-5\end{pmatrix}-\begin{pmatrix}0\\-17\end{pmatrix}=\begin{pmatrix}-12\\12\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}10&-12\\14&12\end{pmatrix}\right|$
Berechne die $2\times2-\mathrm{Matrizen}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
$=\left|120+168\right|=\left|288\right|=288$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
den Punkt $\mathrm A\left(-4\;\left|\;-6\right.\right)$ und die Vektoren $\overrightarrow{\mathrm{AB}}=\begin{pmatrix}-3\\5\end{pmatrix}$ und $\overrightarrow{\mathrm{AC}}=\begin{pmatrix}4\\2\end{pmatrix}$ aufgespannt wird.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{AB}},\overrightarrow{\mathrm{AC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}-3&4\\5&2\end{pmatrix}\right|$
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
$=\left|-6-20\right|=\left|-26\right|=26$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
den Punkt $\mathrm B\left(2\;\left|\;3\right.\right)$ und die Vektoren $\overrightarrow{\mathrm{BA}}=\begin{pmatrix}-4\\1\end{pmatrix}$ und $\overrightarrow{\mathrm{BC}}=\begin{pmatrix}4\\1\end{pmatrix}$ aufgespannt wird.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Parallelogramms (im Zweidimensionalen)
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\det\left(\overrightarrow{\mathrm{BA}},\overrightarrow{\mathrm{BC}}\right)\right|$ .
$\mathrm A=\left|\det\begin{pmatrix}-4&4\\1&1\end{pmatrix}\right|$
Berechne die $2\times2-\mathrm{Matrix}$ mit Hilfe der Formel $\det\begin{pmatrix}\mathrm a&\mathrm b\\\mathrm c&\mathrm d\end{pmatrix}=\mathrm{ad}-\mathrm{bc}$ .
$=\left|-4-4\right|=\left|-8\right|=8$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

4

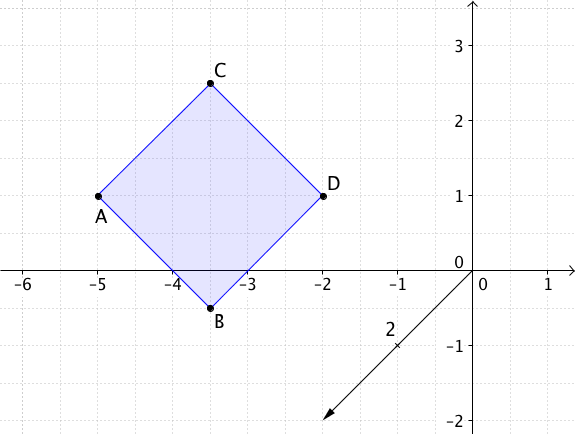
Bestimme den Flächeninhalt des Parallelogramms $A B C D$

$A (- 1 ∣ - 1), B (1 ∣ 2)$ und $D (5 ∣ 2)$
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Determinante
Der Flächeninhalt $F$ ist gegeben durch $F = \left|\overrightarrow{AB} \,\overrightarrow{AC}\right|$ , du brauchst also erst die Vektoren $\overrightarrow{AB}$ und $\overrightarrow{AC}$ zu bestimmen.
$\displaystyle \overrightarrow{AB} = B - A$
$\displaystyle \,\,\,\,\,\,\,\,\,\,\,= \begin{pmatrix}1\\2\end{pmatrix} - \begin{pmatrix}-1\\-1\end{pmatrix} = \begin{pmatrix}2\\3\end{pmatrix}$
$\displaystyle \overrightarrow{AC} = C - A$
$\displaystyle \,\,\,\,\,\,\,\,\,\,\,= \begin{pmatrix}3\\-1\end{pmatrix} - \begin{pmatrix}-1\\-1\end{pmatrix} = \begin{pmatrix}4\\0\end{pmatrix}$
Nun setzst du ein! Beachte dabei, dass die Reihenfolge der Vektoren in der Determinante gegen dem Uhrzeigersinn ist.
$F=\left| \overrightarrow{AC} \overrightarrow{AB}\right|= \begin{vmatrix}4&2\\0&3\end{vmatrix}=4 \cdot 3-2 \cdot 0 = 12$
Also beträgt der Flächeninhalt des Parallelogramms $A B C D$ genau $F = 12$ .
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
$A (- 2 ∣ 2), B (3 ∣ 3)$ und $C (- 1 ∣ 1)$
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Determinante
Der Flächeninhalt $F$ ist gegeben durch $F = \left|\overrightarrow{AB}\,\overrightarrow{AC}\right|$ , du bestimmst also zuerst die Vektoren $\overrightarrow{AB}$ und $\overrightarrow{AC}$ .
$\displaystyle \overrightarrow{AB} = B - A$
$\displaystyle \,\,\,\,\,\,\,\,\,\,\,= \begin{pmatrix}3\\3\end{pmatrix} - \begin{pmatrix}-2\\2\end{pmatrix} = \begin{pmatrix}5\\1\end{pmatrix}$
$\displaystyle \overrightarrow{AC} = C - A$
$\displaystyle \,\,\,\,\,\,\,\,\,\,\,= \begin{pmatrix}-1\\1\end{pmatrix} - \begin{pmatrix}-2\\2\end{pmatrix} = \begin{pmatrix}1\\-1\end{pmatrix}$
Nun setzen wir ein:
$F=\left|\overrightarrow{AC}\,\overrightarrow{AB}\right|=\begin{vmatrix}1&5\\-1&1\end{vmatrix}=1 \cdot 1-5 \cdot (-1) = 6$
Also beträgt der Flächeninhalt des Parallelogramms $A B C D$ genau $F = 6$ .
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

5

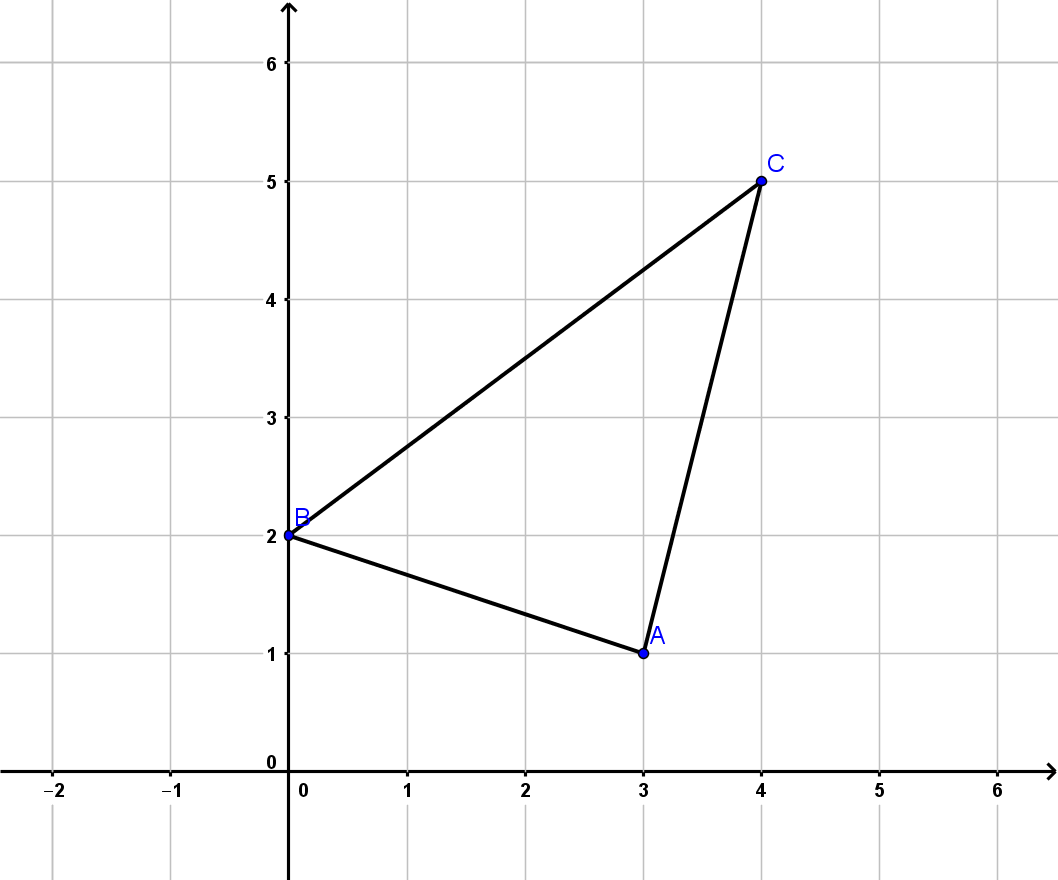
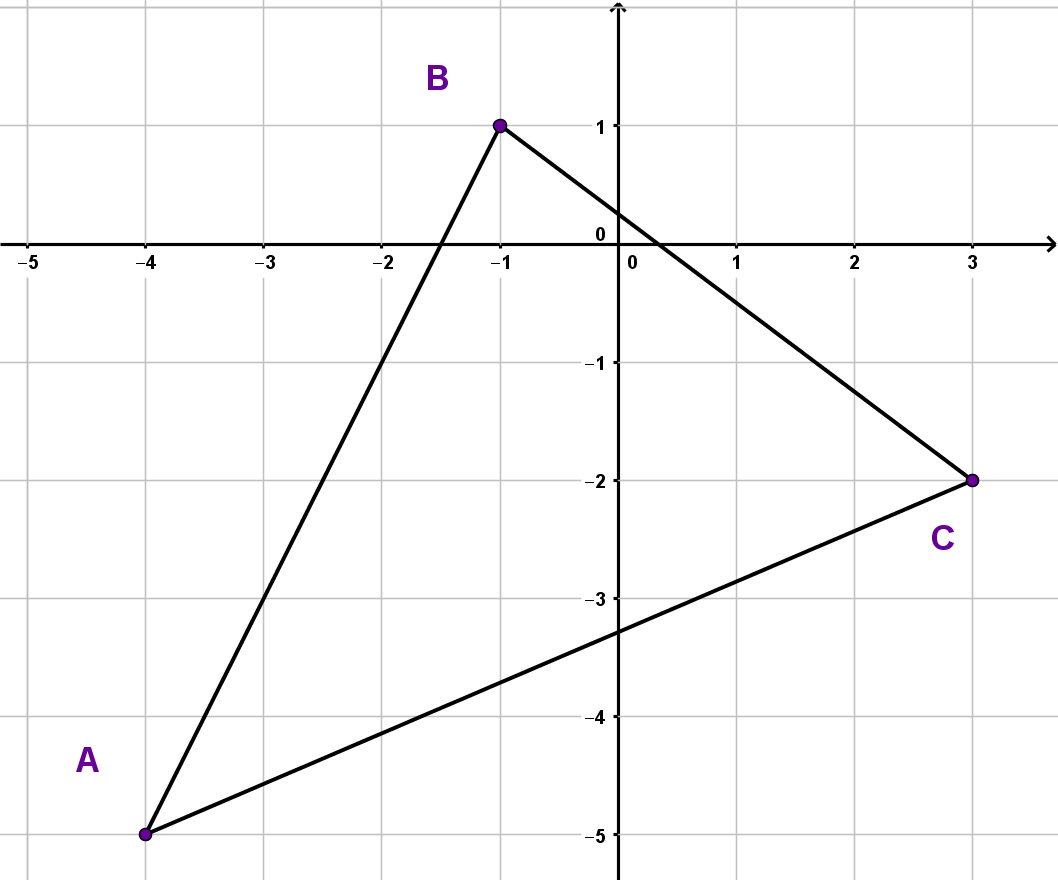
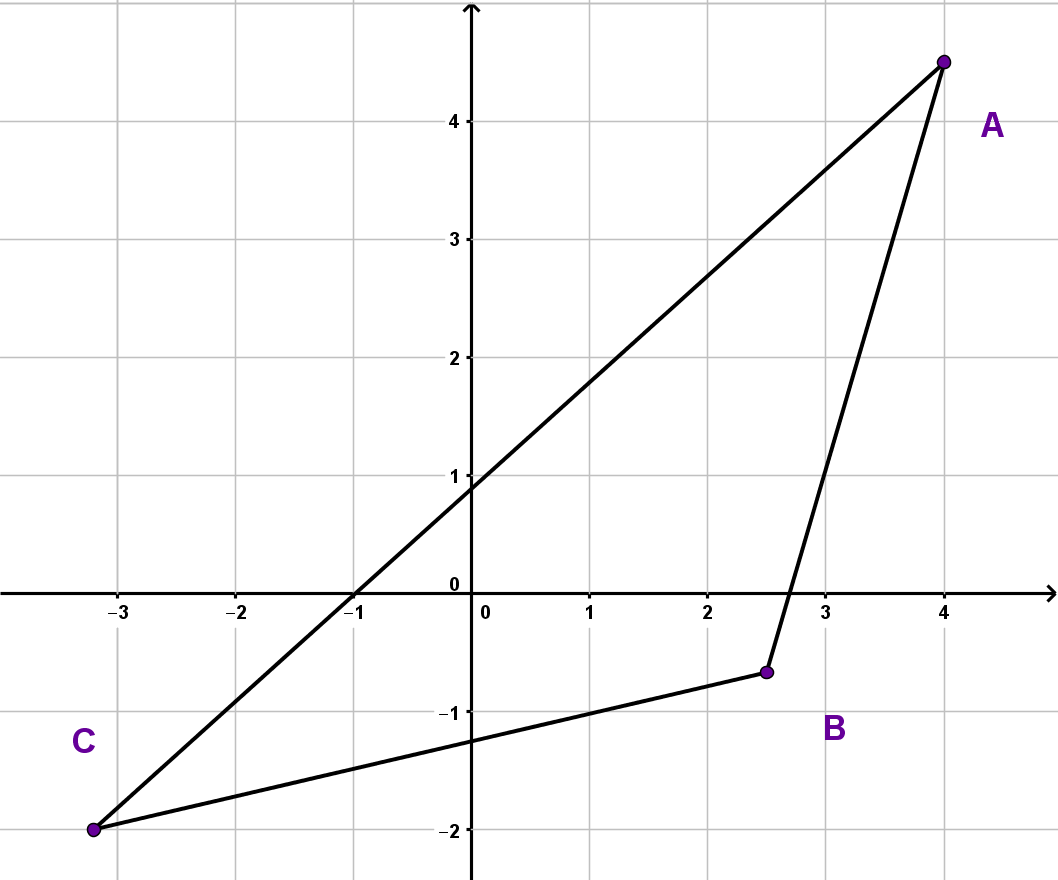
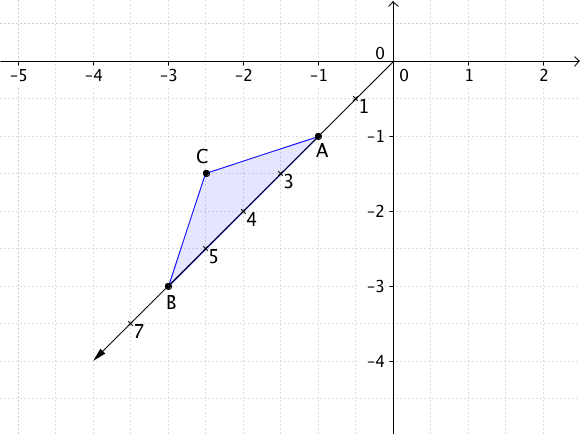
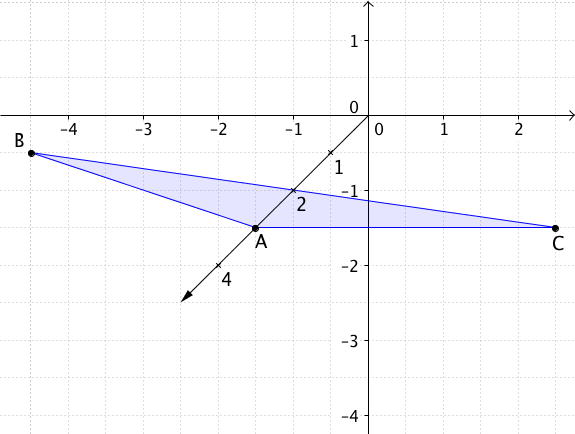
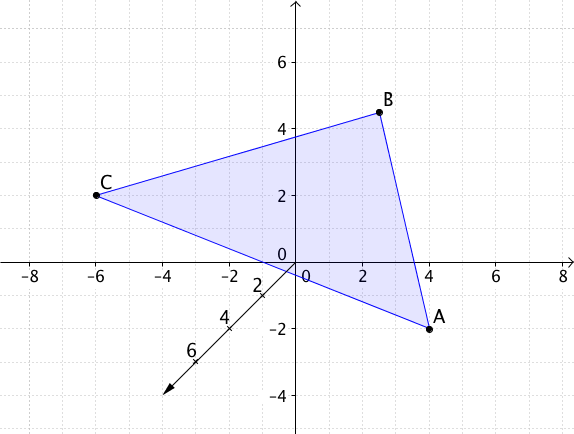
Berechne den Flächeninhalt des Dreiecks, das

durch die Punkte $\mathrm A\left(0\;\left|\;0\;\left|\;0\right.\right.\right)$ , $\mathrm B\left(3\;\left|\;0\;\left|\;0\right.\right.\right)$ , $\mathrm C\left(0\;\left|\;5\;\left|\;0\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}3\\0\\0\end{pmatrix}-\begin{pmatrix}0\\0\\0\end{pmatrix}=\begin{pmatrix}3\\0\\0\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}0\\5\\0\end{pmatrix}-\begin{pmatrix}0\\0\\0\end{pmatrix}=\begin{pmatrix}0\\5\\0\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Dreidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
Berechne das Kreuzprodukt und dann den Betrag des Vektors .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\begin{pmatrix}3\\0\\0\end{pmatrix}\times\begin{pmatrix}0\\5\\0\end{pmatrix}\right|$
$=\frac12\cdot\left|\begin{pmatrix}0\\0\\15\end{pmatrix}\right|$
$=\frac12\cdot\sqrt{0^2+0^2+15^2}$
$=\frac12\cdot15=7{,}5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(3\;\left|\;1\;\left|\;1\right.\right.\right)$ , $\mathrm B\left(4\;\left|\;-1\;\left|\;-1\right.\right.\right)$ , $\mathrm C\left(3\;\left|\;-1\;\left|\;0\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}4\\-1\\-1\end{pmatrix}-\begin{pmatrix}3\\1\\1\end{pmatrix}=\begin{pmatrix}1\\-2\\-2\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}3\\-1\\0\end{pmatrix}-\begin{pmatrix}3\\1\\1\end{pmatrix}=\begin{pmatrix}0\\-2\\-1\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Dreidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
Berechne das Kreuzprodukt und dann den Betrag des Vektors .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\begin{pmatrix}1\\-2\\-2\end{pmatrix}\times\begin{pmatrix}0\\-2\\-1\end{pmatrix}\right|$
$=\frac12\cdot\left|\begin{pmatrix}-2\\1\\-2\end{pmatrix}\right|$
$=\frac12\cdot\sqrt{\left(-2\right)^2+1^2+\left(-2\right)^2}$
$=\frac12\cdot\sqrt{4+1+4}$
$=\frac12\cdot\sqrt9=\frac32=1{,}5$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(5\;\left|\;1\;\left|\;1\right.\right.\right)$ , $\mathrm B\left(3\;\left|\;-3\;\left|\;1\right.\right.\right)$ , $\mathrm C\left(5\;\left|\;5\;\left|\;-1\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche des Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}3\\-3\\1\end{pmatrix}-\begin{pmatrix}5\\1\\1\end{pmatrix}=\begin{pmatrix}-2\\-4\\0\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}5\\5\\-1\end{pmatrix}-\begin{pmatrix}5\\1\\1\end{pmatrix}=\begin{pmatrix}0\\4\\-2\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Dreidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
Berechne das Kreuzprodukt und dann den Betrag des Vektors.
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\begin{pmatrix}-2\\-4\\0\end{pmatrix}\times\begin{pmatrix}0\\4\\-2\end{pmatrix}\right|$
$=\frac12\cdot\left|\begin{pmatrix}8\\-4\\-8\end{pmatrix}\right|$
$=\frac12\cdot\sqrt{8^2+\left(-4\right)^2+\left(-8\right)^2}$
$=\frac12\cdot\sqrt{64+16+64}$
$=\frac12\cdot\sqrt{144}=\frac{12}2=6$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(11\;\left|\;9\;\left|\;7\right.\right.\right)$ , $\mathrm B\left(4\;\left|\;6\;\left|\;11\right.\right.\right)$ , $\mathrm C\left(8\;\left|\;9\;\left|\;10\right.\right.\right)$ gegeben ist.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}4\\6\\11\end{pmatrix}-\begin{pmatrix}11\\9\\7\end{pmatrix}=\begin{pmatrix}-7\\-3\\4\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}8\\9\\10\end{pmatrix}-\begin{pmatrix}11\\9\\7\end{pmatrix}=\begin{pmatrix}-3\\0\\3\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Dreidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
Berechne das Kreuzprodukt und dann den Betrag des Vektors .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\begin{pmatrix}-7\\-3\\4\end{pmatrix}\times\begin{pmatrix}-3\\0\\3\end{pmatrix}\right|$
$=\frac12\cdot\left|\begin{pmatrix}-9\\9\\-9\end{pmatrix}\right|$
$=\frac12\cdot\sqrt{\left(-9\right)^2+9^2+\left(-9\right)^2}$
$=\frac12\cdot\sqrt{3\cdot9^2}$
$=\frac92\sqrt3$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(2\;\left|\;5\;\left|\;-1\right.\right.\right)$ , $\mathrm B\left(-3\;\left|\;1\;\left|\;3\right.\right.\right)$ , $\mathrm C\left(4\;\left|\;-4\;\left|\;4\right.\right.\right)$ gegeben ist.

Für diese Aufgabe benötigst Du folgendes Grundwissen: Fläche eines Dreiecks
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}-3\\1\\3\end{pmatrix}-\begin{pmatrix}2\\5\\-1\end{pmatrix}=\begin{pmatrix}-5\\-4\\4\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}4\\-4\\4\end{pmatrix}-\begin{pmatrix}2\\5\\-1\end{pmatrix}=\begin{pmatrix}2\\-9\\5\end{pmatrix}$
Setze in die Formel für die Fläche eines Dreiecks im Dreidimensionalen ein: ${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
Berechne das Kreuzprodukt und dann den Betrag des Vektors .
${\mathrm A}_\mathrm\Delta=\frac12\cdot\left|\begin{pmatrix}-5\\-4\\4\end{pmatrix}\times\begin{pmatrix}2\\-9\\5\end{pmatrix}\right|$
$=\frac12\cdot\left|\begin{pmatrix}16\\33\\53\end{pmatrix}\right|$
$=\frac12\cdot\sqrt{16^2+33^2+53^2}$
$=\frac12\cdot\sqrt{256+1089+2809}$
$=\frac12\cdot\sqrt{4154}=\sqrt{\frac{2077}2}$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

6

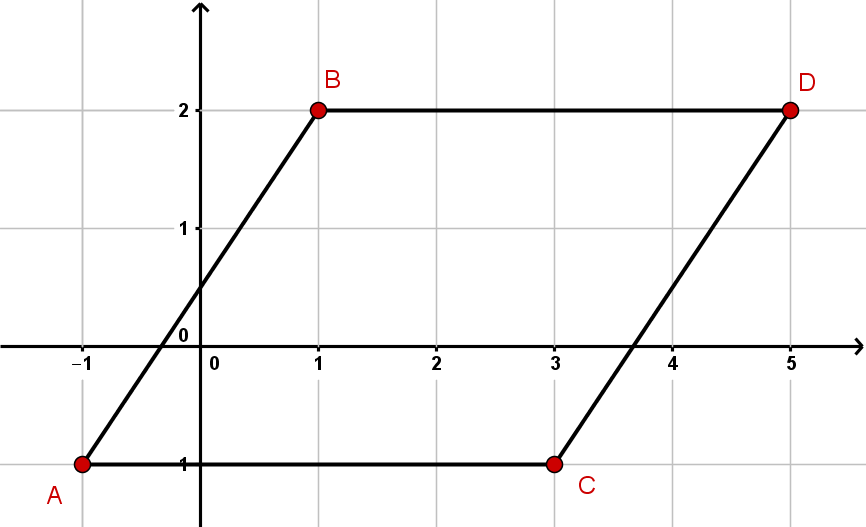
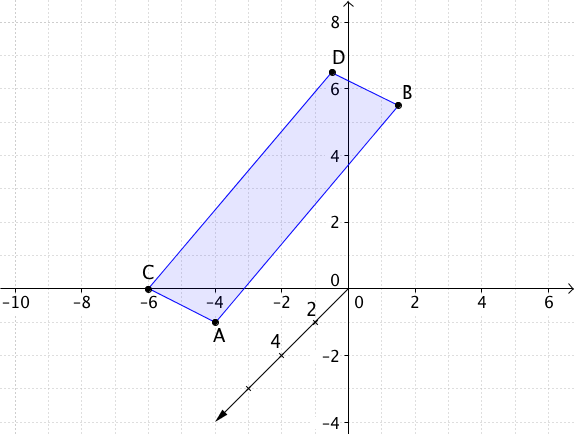
Berechne den Flächeninhalt des Parallelogramms, das durch folgende Punkte gegeben ist. Runde das Ergebnis wenn nötig bis auf zwei Nachkommastellen.

durch die Punkte $\mathrm A\left(0\;\left|\;0\;\left|\;0\right.\right.\right)$ , $\mathrm B\left(0\;\left|\;6\;\left|\;2\right.\right.\right)$ , $\mathrm C\left(0\;\left|\;2\;\left|\;6\right.\right.\right)$ , $\mathrm D\left(0\;\left|\;8\;\left|\;8\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Flächenberechnung in der analytischen Geometrie
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}0\\6\\2\end{pmatrix}-\begin{pmatrix}0\\0\\0\end{pmatrix}=\begin{pmatrix}0\\6\\2\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}0\\2\\6\end{pmatrix}-\begin{pmatrix}0\\0\\0\end{pmatrix}=\begin{pmatrix}0\\2\\6\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
$\mathrm A=\left|\begin{pmatrix}0\\6\\2\end{pmatrix}\times\begin{pmatrix}0\\2\\6\end{pmatrix}\right|$
Berechne nun das Kreuzprodukt und danach den Betrag des Vektors.
$\mathrm A=\left|\begin{pmatrix}0\\6\\2\end{pmatrix}\times\begin{pmatrix}0\\2\\6\end{pmatrix}\right|\\\phantom{A}=\left|\begin{pmatrix}32\\0\\0\end{pmatrix}\right|\\\\\phantom{A}=\sqrt{32^2+0^2+0^2}\\\phantom{A}=32$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(4\;\left|\;-3\;\left|\;3\right.\right.\right)$ , $\mathrm B\left(1\;\left|\;-3\;\left|\;0\right.\right.\right)$ , $\mathrm C\left(5\;\left|\;-1\;\left|\;5\right.\right.\right)$ , $\mathrm D\left(2\;\left|\;-1\;\left|\;2\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Flächenberechnung in der analytischen Geometrie
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}1\\-3\\0\end{pmatrix}-\begin{pmatrix}4\\-3\\3\end{pmatrix}=\begin{pmatrix}-3\\0\\-3\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}5\\-1\\5\end{pmatrix}-\begin{pmatrix}4\\-3\\3\end{pmatrix}=\begin{pmatrix}1\\2\\2\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
$\mathrm A=\left|\begin{pmatrix}-3\\0\\-3\end{pmatrix}\times\begin{pmatrix}1\\2\\2\end{pmatrix}\right|$
Berechne nun das Kreuzprodukt und danach den Betrag des Vektors.
$\mathrm A=\left|\begin{pmatrix}-3\\0\\-3\end{pmatrix}\times\begin{pmatrix}1\\2\\2\end{pmatrix}\right|\\\phantom{A}=\left|\begin{pmatrix}-6\\-3\\6\end{pmatrix}\right|\\\phantom{A}=\sqrt{\left(-6\right)^2+\left(-3\right)^2+6^2}\\\phantom{A}=\sqrt{36+9+36}\\\phantom{A}=\sqrt{81}\\\phantom{A}=9$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(2\;\left|\;-1\;\left|\;2\right.\right.\right)$ , $\mathrm B\left(0\;\left|\;1\;\left|\;1\right.\right.\right)$ , $\mathrm C\left(2\;\left|\;2\;\left|\;4\right.\right.\right)$ , $\mathrm D\left(0\;\left|\;4\;\left|\;3\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Flächenberechnung in der analytischen Geometrie
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}0\\1\\1\end{pmatrix}-\begin{pmatrix}2\\-1\\2\end{pmatrix}=\begin{pmatrix}-2\\2\\-1\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}2\\2\\4\end{pmatrix}-\begin{pmatrix}2\\-1\\2\end{pmatrix}=\begin{pmatrix}0\\3\\2\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
$\mathrm A=\left|\begin{pmatrix}-2\\2\\-1\end{pmatrix}\times\begin{pmatrix}0\\3\\2\end{pmatrix}\right|$
Berechne nun das Kreuzprodukt und danach den Betrag des Vektors.
$\mathrm A=\left|\begin{pmatrix}-2\\2\\-1\end{pmatrix}\times\begin{pmatrix}0\\3\\2\end{pmatrix}\right|\\\phantom{A}=\left|\begin{pmatrix}7\\4\\-6\end{pmatrix}\right|\\\phantom{A}=\sqrt{7^2+4^2+\left(-6\right)^2}\\\phantom{A}=\sqrt{49+16+36}\\\phantom{A}=\sqrt{101}\\\phantom{A}\approx 10{,}05$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉
durch die Punkte $\mathrm A\left(-4\;\left|\;-2\;\left|\;1\right.\right.\right)$ , $\mathrm B\left(-1\;\left|\;2\;\left|\;6\right.\right.\right)$ , $\mathrm C\left(-6\;\left|\;-3\;\left|\;3\right.\right.\right)$ , $\mathrm D\left(-3\;\left|\;1\;\left|\;8\right.\right.\right)$ gegeben ist.
FE

Für diese Aufgabe benötigst Du folgendes Grundwissen: Flächenberechnung in der analytischen Geometrie
Berechne zuerst die Vektoren $\overrightarrow{\mathrm{AB}}$ und $\overrightarrow{\mathrm{AC}}$ .
Der Punkt $\mathrm D$ wird zur Berechnung des Flächeninhalts nicht benötigt und ist nur der Vollständigkeit halber angegeben.
$\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm B}-\overrightarrow{\mathrm A}=\begin{pmatrix}-1\\2\\6\end{pmatrix}-\begin{pmatrix}-4\\-2\\1\end{pmatrix}=\begin{pmatrix}3\\4\\5\end{pmatrix}$
$\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm C}-\overrightarrow{\mathrm A}=\begin{pmatrix}-6\\-3\\3\end{pmatrix}-\begin{pmatrix}-4\\-2\\1\end{pmatrix}=\begin{pmatrix}-2\\-1\\2\end{pmatrix}$
Setze in die Formel für die Fläche eines Parallelogramms im Zweidimensionalen ein: ${\mathrm A}_\mathrm{Parallelogramm}=\left|\overrightarrow{\mathrm{AB}}\times\overrightarrow{\mathrm{AC}}\right|$ .
$\mathrm A=\left|\begin{pmatrix}3\\4\\5\end{pmatrix}\times\begin{pmatrix}-2\\-1\\2\end{pmatrix}\right|$
Berechne nun das Kreuzprodukt und danach den Betrag des Vektors.
$\mathrm A=\left|\begin{pmatrix}3\\4\\5\end{pmatrix}\times\begin{pmatrix}-2\\-1\\2\end{pmatrix}\right|\\\phantom{A}=\left|\begin{pmatrix}13\\-16\\5\end{pmatrix}\right|\\\phantom{A}=\sqrt{13^2+\left(-16\right)^2+5^2}\\\phantom{A}=\sqrt{169+256+25}\\\phantom{A}=\sqrt{450}\\\phantom{A}=15\sqrt{2}\\\phantom{A}=21{,}21$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

7

Berechne den Flächeninhalt des Dreiecks $\triangle ABC$ mit $A (1 ∣ 2)$ , $B (- 1 ∣ 2)$ und $C(x|\sin(x))$ in Abhängigkeit von $x$ .

8

Die Punkte sind gegeben durch $A (- 3 ∣ 4)$ und $B (0 ∣ 1)$ , $C$ bewegt sich dabei auf der Funktion $\ln(x-3)$ in Abhängigkeit von $x$ .

Bestimme zuerst den Definitionsbereich von $x$ und dann den Flächeninhalt des Dreiecks $\Delta ABC$ .

Für diese Aufgabe benötigst Du folgendes Grundwissen: Flächenberechnung mit Determinante

Bestimmen des Definitionsbereiches

Zunächst bestimmst du den Definitionsbereich von $x$ .

Betrachte hierfür $\ln(x-3)$ . Der natürliche Logarithmus ist für negative Zahlen sowie für null nicht definiert. Für das Argument des Logarithmus muss also gelten:

$x - 3 > 0 \,\, \Longleftrightarrow\,\, x>3$

Damit ist der Definitionsbereich $D =\, ]\,3, \infty\,[$ .

Berechnen des Flächeninhaltes

Stelle zuerst zwei Seiten des Dreiecks als Vektoren auf.

\displaystyle \vec v = \overrightarrow{AB} = B - A = \begin{pmatrix} 3\\-3 \end{pmatrix}

\displaystyle \vec w = \overrightarrow{AC} = C - A = \begin{pmatrix}x+3\\\ln(x-3)-4 \end{pmatrix}

Benutze nun die Formel zur Flächenberechnung eines Dreiecks mithilfe der Determinante. Gegen den Uhrzeigersinn betrachtet kommt $\vec v$ vor $\vec w$ , also schreibst du zuerst $\vec v$ , dann $\vec w$ in die Formel.

\displaystyle \def\arraystretch{1.25} \begin{array}{rcl} F(x) = \frac12 |\vec v \vec w| &=& \frac12 \begin{vmatrix}3&x+3\\-3&\ln(x-3)-4\end{vmatrix} \\\\ &=& \frac12 \left( 3\cdot(\ln(x-3)-4)-(x+3)\cdot (-3) \right) \\\\ &=& 1{,}5\ln(x-3)+1{,}5x - 1{,}5 \end{array}

Nach Ausrechnen der Determinante bekommst du für den Flächeninhalt also:

\displaystyle F(x) = 1{,}5\ln(x-3) + 1{,}5x - 1{,}5

Unten kannst du, in dem du den Punkt $C$ entlang der Funktion $\,\ln(x-3)\,$ bewegst, den Flächeninhalt des Dreiecks $\Delta ABC$ verändern.

Mit einem Klick auf Bild oder Button oben stimmst du zu, dass externe Inhalte von GeoGebra geladen werden. Dabei können persönliche Daten zu diesem Service übertragen werden – entsprechend unserer Datenschutzerklärung.

[https://www.geogebra.org/material/iframe/id/1624393]

Hast du eine Frage oder Feedback?

Bitte melde dich an, um diese Funktion zu benutzen.

9

Berechne den Flächeninhalt des Dreiecks $\triangle ABC$ , wobei $A (- 4 ∣ - 1,5)$ und $B (- 1 ∣ 6)$ Punkte sind und der Punkt $C$ sich auf der Funktion $\,y(x) = x^3 - x +1$ in Abhängigkeit von $x$ bewegt.

10

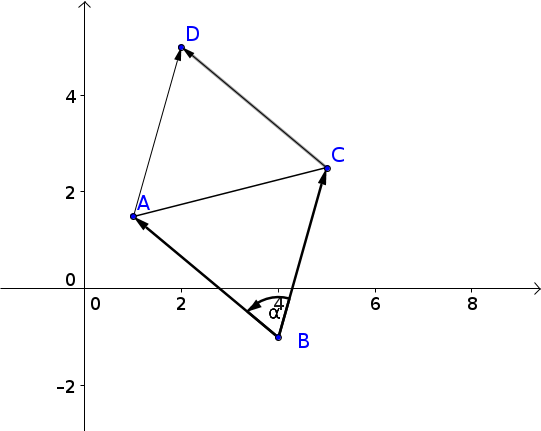
Berechne die Fläche des Parallelogramms, das von den angegebenen Punkten aufgespannt wird.

$A (1 ∣ 1,5); B (4 ∣ - 1); C (5 ∣ 2,5)$
FE

Für diese Aufgabe benötigst du - je nach Lösungsweg - Grundwissen über die Determinante oder über das Kreuzprodukt.
$A (1 ∣ 1,5); B (4 ∣ - 1); C (5 ∣ 2,5)$
Zeichne die Punkte in ein Koordinatensystem ein, um einen Überblick zu erhalten. Es ist nicht nötig, das Parallelogramm aus den angegeben Punkt zu konstruieren, da es nur zwei Vektoren benötigt, um die Fläche zu berechnen. Wähle eine Ecke, von der die Vektoren das Parallelogramm aufspannen, und berechne diese Vektoren.
Auch hier ist es egal, welche Ecke man wählt, da die Parallelogrammfläche stets das Doppelte der gewählten Dreiecksfläche ist.
$\overrightarrow{BA}=\begin{pmatrix}1-4\\1{,}5-(-1)\end{pmatrix}=\begin{pmatrix}-3\\2{,}5\end{pmatrix}$
$\overrightarrow{BC}=\begin{pmatrix}5-4\\2{,}5-(-1)\end{pmatrix}=\begin{pmatrix}1\\3{,}5\end{pmatrix}$
1. Lösungsweg: Flächenberechnung mit Determinante
Bestimme die Reihenfolge der Vektoren in der Determinante gegen den Uhrzeigersinn ( $\mathrm\alpha$ ) oder setze um die Determinante einen Betrag.
Wichtig: KEIN $\frac12$ !
Berechne die Determinante und erhalte dann das Ergebnis. Flächeneinheit dabei nicht vergessen, wenn gefordert.
$\mathrm A=\left|\begin{vmatrix}\overrightarrow{\mathrm{BA}}&\overrightarrow{\mathrm{BC}}\end{vmatrix}\right|=\begin{vmatrix}\overrightarrow{\mathrm{BC}}&\overrightarrow{\mathrm{BA}}\end{vmatrix}=\begin{vmatrix}1&-3\\3{,}5&2{,}5\end{vmatrix}=2{,}5+10{,}5=13\;FE$
2. Lösungsweg: Flächenberechnung mit Kreuzprodukt
Bette die Zeichenebene in den $\mathbb{R}^3$ ein. Dies geschieht, indem jedem Vektor als dritte Komponente der Eintrag $0$ hinzugefügt wird.
Berechne nun das Kreuzprodukt $\overrightarrow{BA}\times\overrightarrow{BC}$ . Das Ergebnis ist ein zu $\overrightarrow{BA}$ und $\overrightarrow{BC}$ orthogonaler Vektor, dessen Betrag dem Flächeninhalt des von $\overrightarrow{BA}$ und $\overrightarrow{BC}$ aufgespannten Parallelogramms entspricht.
$\displaystyle A=\left|\overrightarrow{BA}\times\overrightarrow{BC}\right|=\left|\begin{pmatrix}-3\\2{,}5\\0\end{pmatrix}\times\begin{pmatrix}1\\3{,}5\\0\end{pmatrix}\right|=\left|\begin{pmatrix}0\\0\\-13\end{pmatrix}\right|=13\;FE$
Hast du eine Frage oder Feedback?
Kommentiere hier 👉

$\displaystyle F(x)$	$=$	$\displaystyle \frac{1}{2}\|\vec{v}\vec{w}\|$
	↓	$\vec{v}$ , $\vec{w}$ einsetzen
	$=$	$\displaystyle \frac12 \begin{vmatrix}-2&x-1\\0&\sin(x)-2\end{vmatrix}$
	↓	Determinante berechnen
	$=$	$\displaystyle \frac{1}{2}\left(-2\cdot(\sin(x)-2)-(x-1)\cdot0\right)$
	$=$	$\displaystyle 2-\sin(x)$

Aufgaben zur Flächenberechnung

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Hast du eine Frage oder Feedback?

Berechnung des Flächeninhalts mit Determinante

Bestimmen des Definitionsbereiches

Berechnen des Flächeninhaltes

1. Lösungsweg: Flächenberechnung mit Determinante

2. Lösungsweg: Flächenberechnung mit Kreuzprodukt

Hast du eine Frage oder Feedback?