Aufgaben zur Diskussion von Polynomfunktionen
- 1
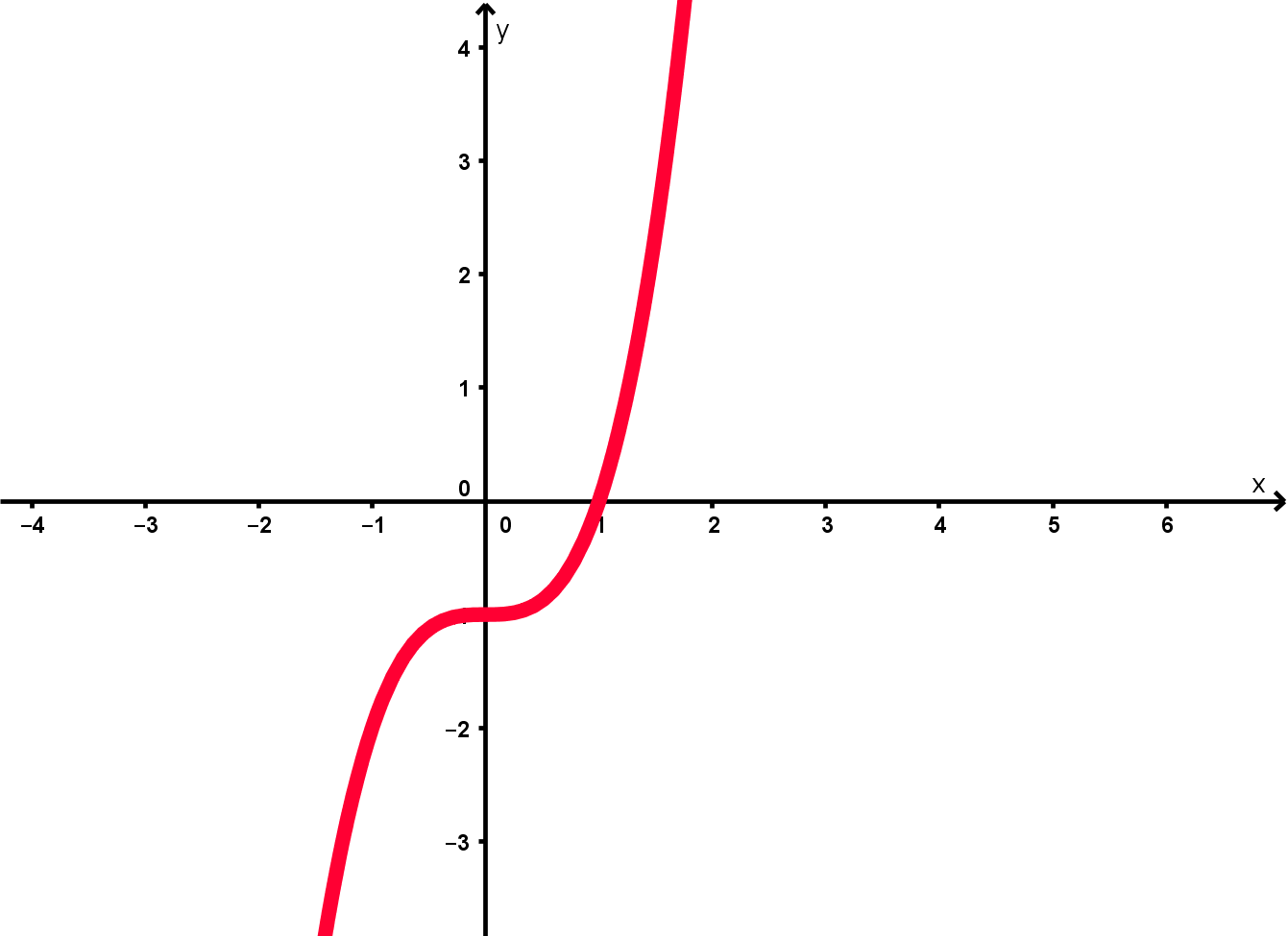
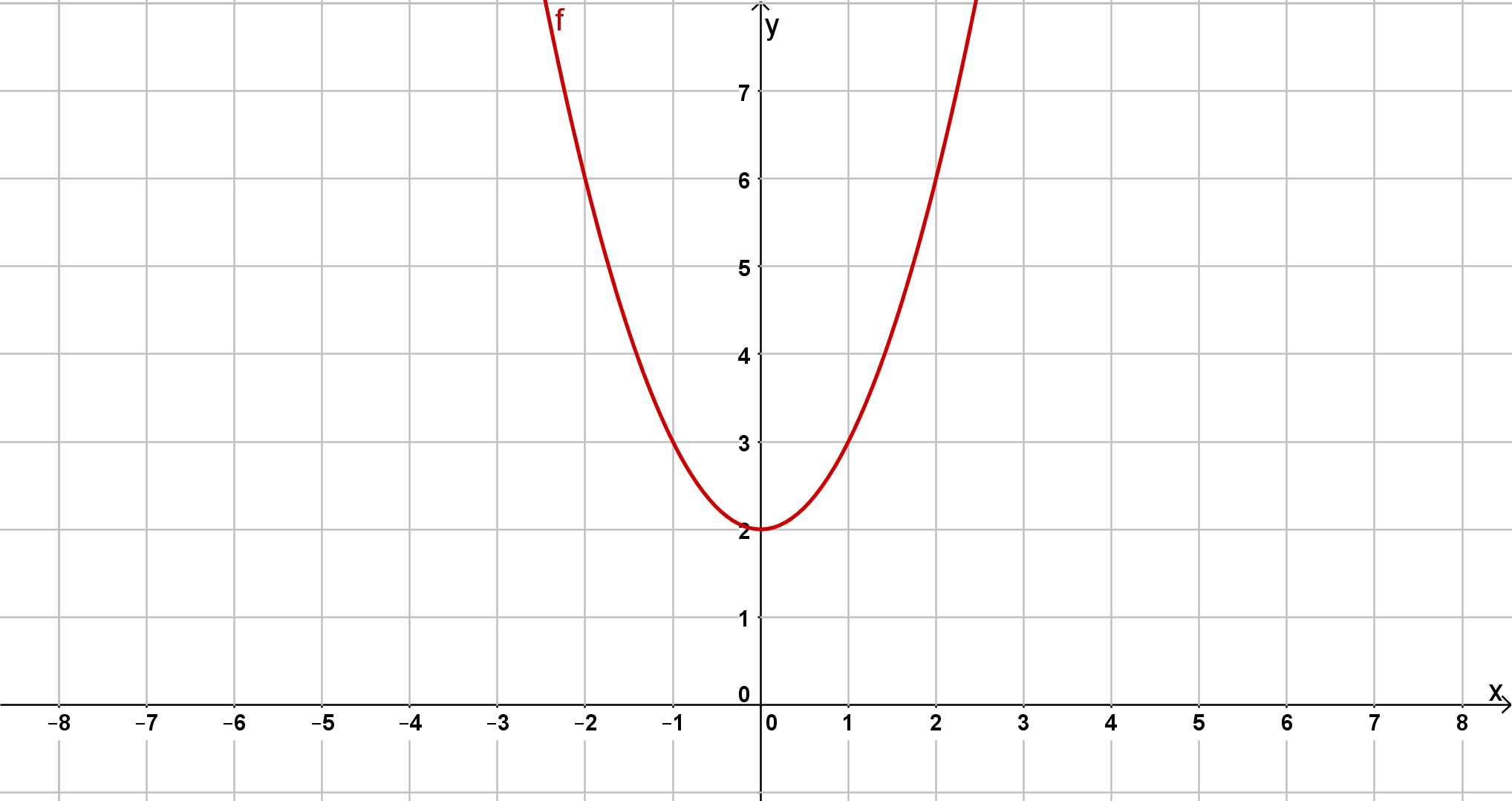
Welcher Funktionsterm gehört zum Graph?
- 2
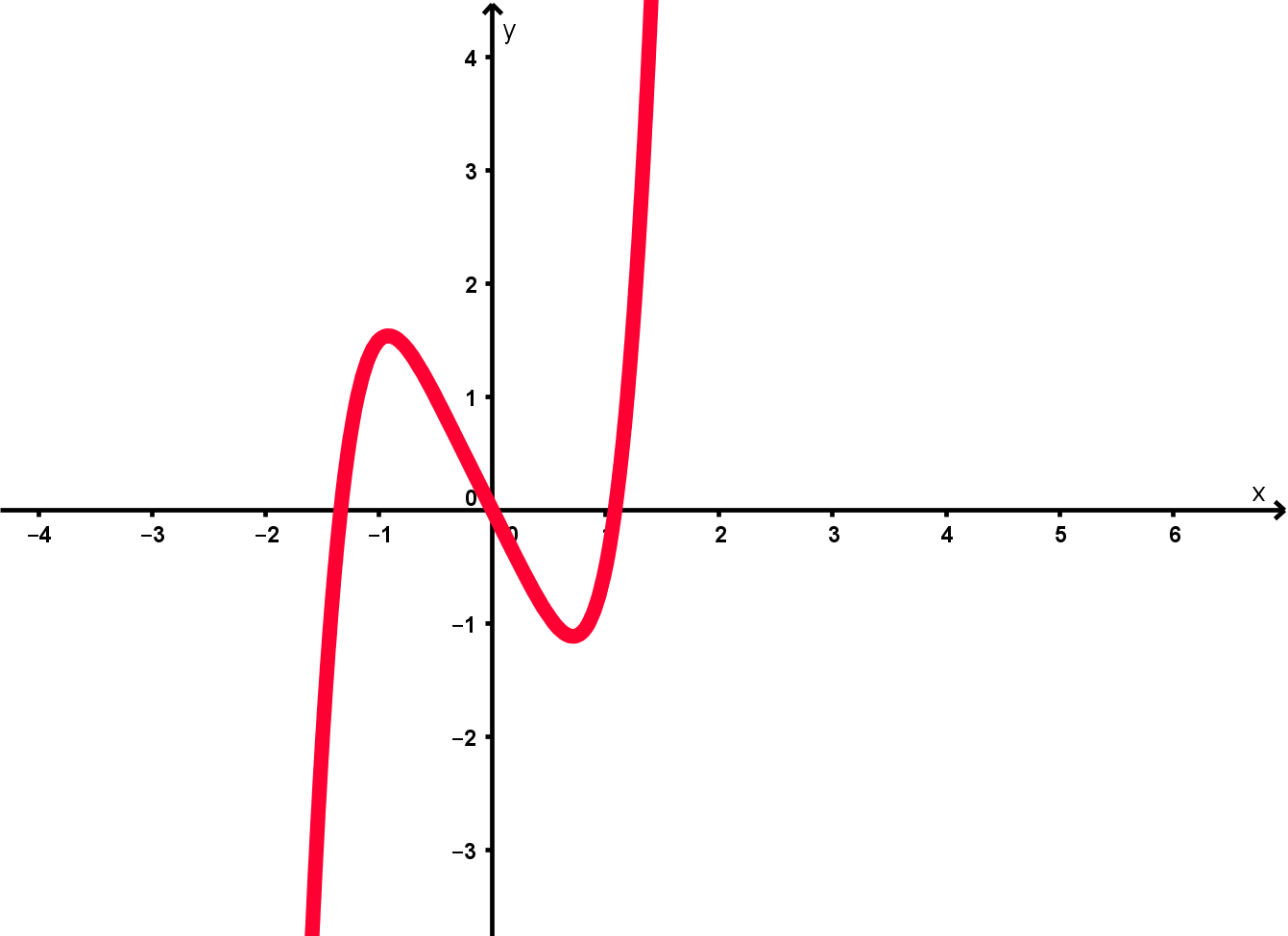
Untersuche den Graphen der Funktion mit soweit, sodass du ihn zeichnen kannst.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Kurvendiskussion
Ohne Wertetabelle ist es immer geschickt, sich über den Verlauf des Graphens Gedanken zu machen. Hilfreich hierbei sind vor allem erst einmal Nullstellen. Danach schaust du dir das Verhalten der Funktion im Unendlichen an.Um die Nullstellen herauszufinden gibt es zwei Möglichkeiten. Einmal kann man sie bei Polynomen mit einem Grad größer als zwei mit der Polynomdivision herausfinden oder bei dem Grad vier bietet sich auch die Substitution an.
Lösung 1: mit Polynomdivision
1. Schritt: Nullstellen raten
Schaue dir beim raten von Nullstellen die letzte Ziffer ohne ein an, wie kannst du die in ein Produkt aufteilen? Zum Beispiel in und . Probiere es mit : Super, eine Nullstelle gefunden!
2. Schritt: Polynomdivision um weitere Nullstellen zu finden
Jetzt sollte man die Wurzel ziehen um auf das zu kommen. Dann würde aber etwas negatives unter der Wurzel stehen, dies ist nicht erlaubt. Also gibt es keine weitere Nullstelle.
3. Schritt: Verhalten im Unendlichen
Setze jetzt überall da wo in der Funktion ein steht ein bzw. ein und schaue was raus kommt. Allerdings darfst du das nur in Anführungszeichen schreiben, da dies eigentlich keine mathematische Ausdrucksweise ist und somit nur eine inoffizielle Lösung aber eine gute Hilfe um sich das besser vorstellen zu können.
Schaue dir jetzt das Vorzeichen vor dem höchsten Exponenten/Grad an: hier steht ein Minus. Also kommt insgesamt Minus Unendlich raus.
Bei geraden Exponenten wird das Minus in der Klammer wieder zu einem Plus und du kommst auf das selbe Ergebnis.
Der Verlauf ist also "von unten nach unten".
4. Schritt: Symmetrie
Es können drei Fälle eintreten: Achsensymmetrie, Punktsymmetrie, keine Symmetrie. Ersetze dafür jedes mit einem .
Jetzt musst du dir die Exponenten/Potenzen anschauen, hier sind das nur gerade, also fallen unsere Minuszeichen vor den weg. Damit bist du wieder bei der Funktion gelandet.
Welche Symmetrie war das? Richtig, die Achsensymmetrie.
5. Schritt: y-Achsen Abschnitt
Um den Schnittpunkt einer Funktion mit der y-Achse zu ermitteln, muss für den -Wert eingesetzt werden.
6. Schritt: Graphen zeichnen
Lösung 2: durch Substitution
Bei der Substitution wird in einem Term ein Teil (z.B. ) durch einen neuen Term (z.B. ) ersetzt.
Ersetze nun jedes mit .
Aus dieser quadratischen Funktion kannst du jetzt die Nullstellen mit Hilfe der Mitternachtsformel ausrechnen.
und
Nun musst du rücksubstituieren.
Um auf und zu kommen musst du also die Wurzel aus und ziehen. Das funktioniert allerdings nur bei , da negativ ist. Aber Achtung: Nur die Wurzel ziehen ist keine Äquivalenzumformung, deshalb musst du die ziehen.
So bist du wieder bei den Nullstellen von oben angekommen und kannst bei Schritt 3: Verhalten im Unendlichen weiter machen.
Überlege dir, was du alles benötigst, um den Graphen zeichnen zu können:
Nullstellen
Verhalten im Unendlichen
Symmetrie
y-Achsen Abschnitt
- 3
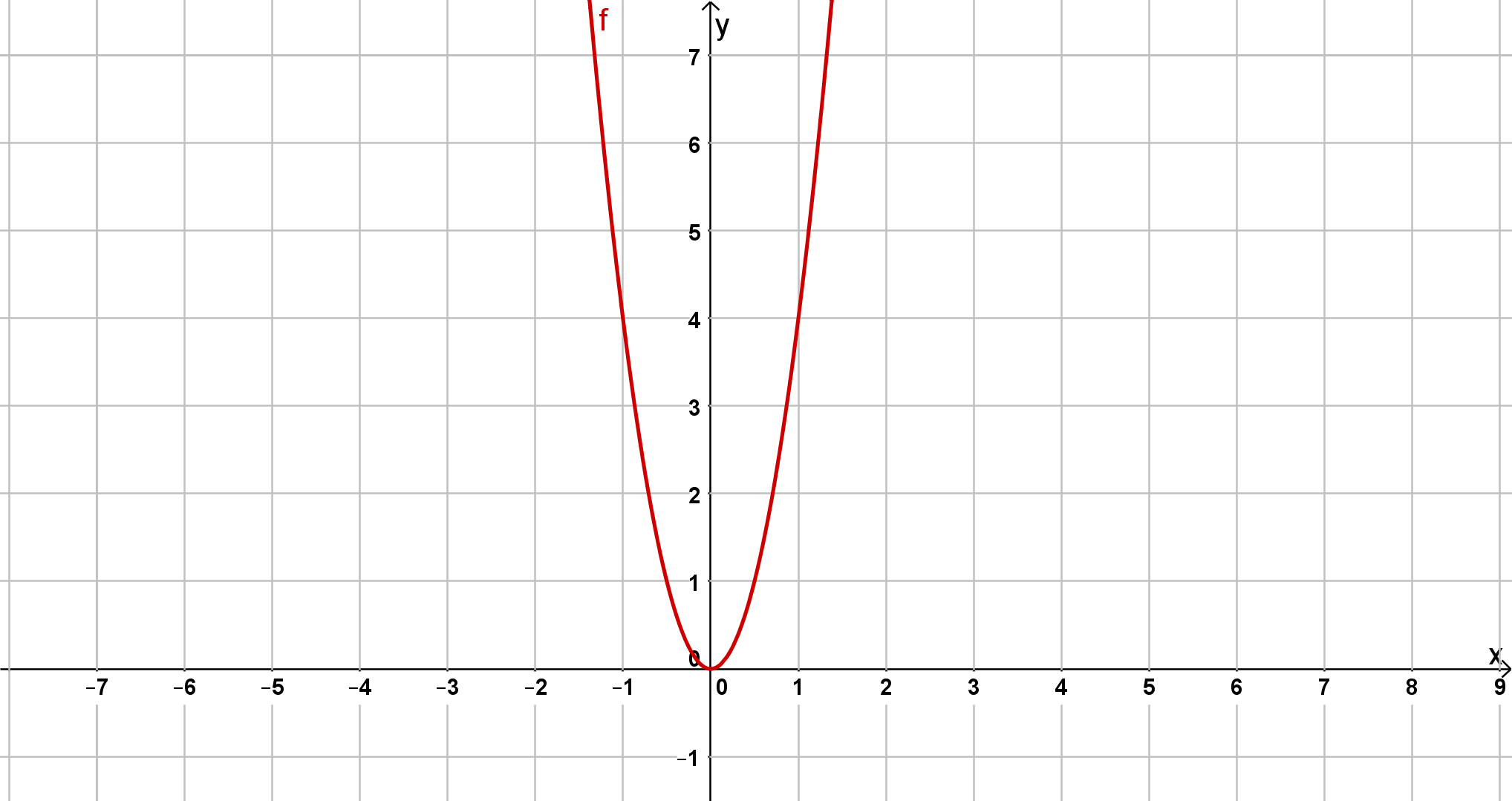
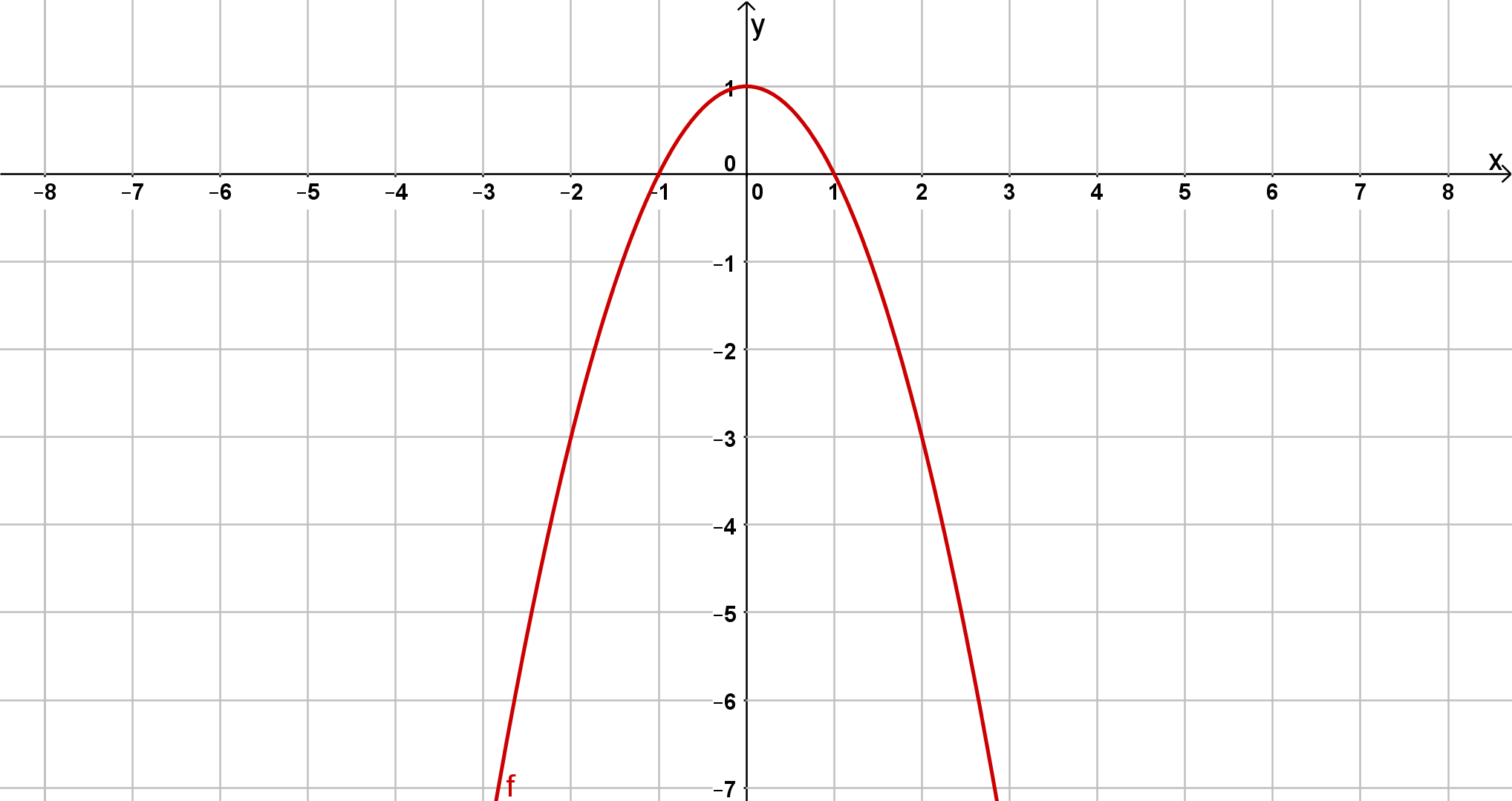
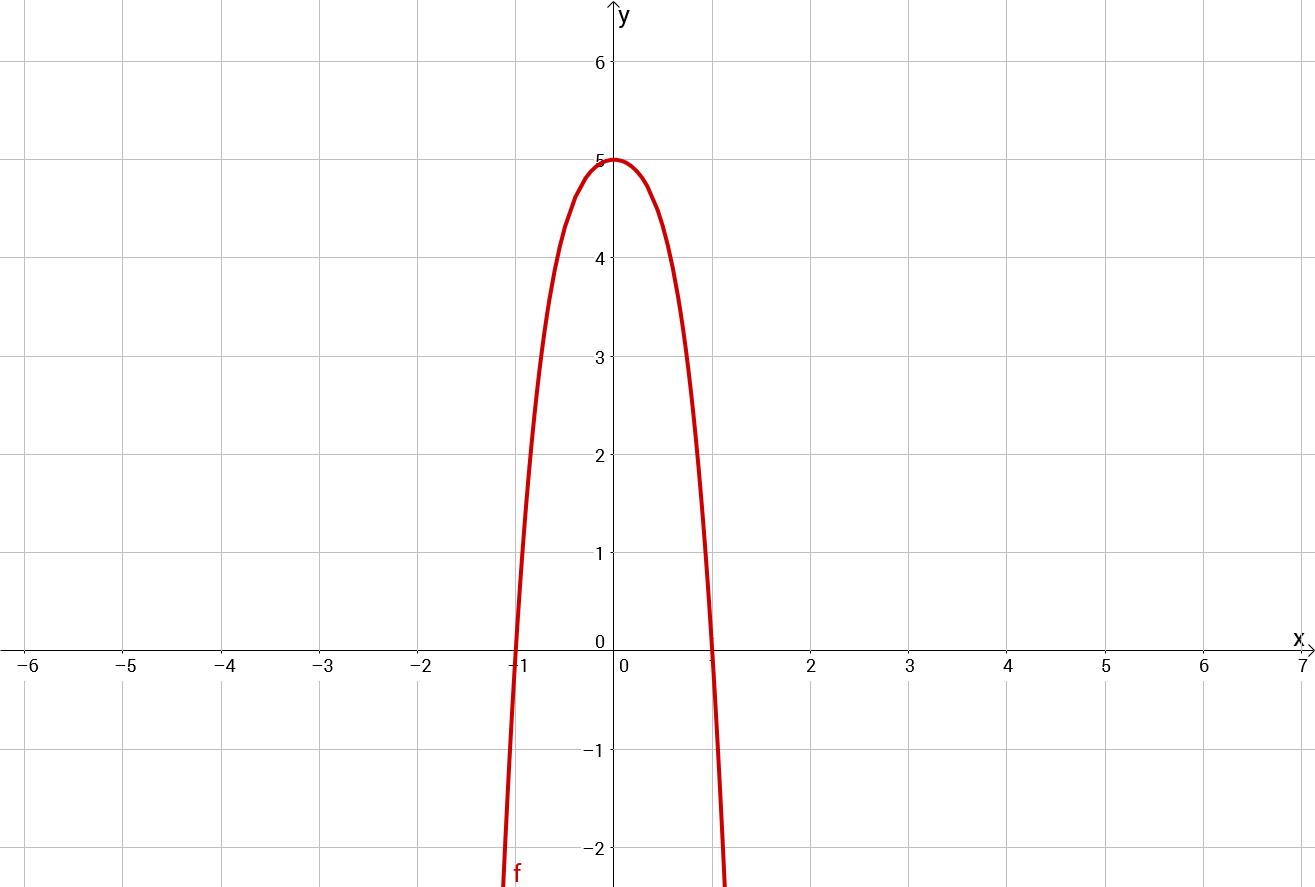
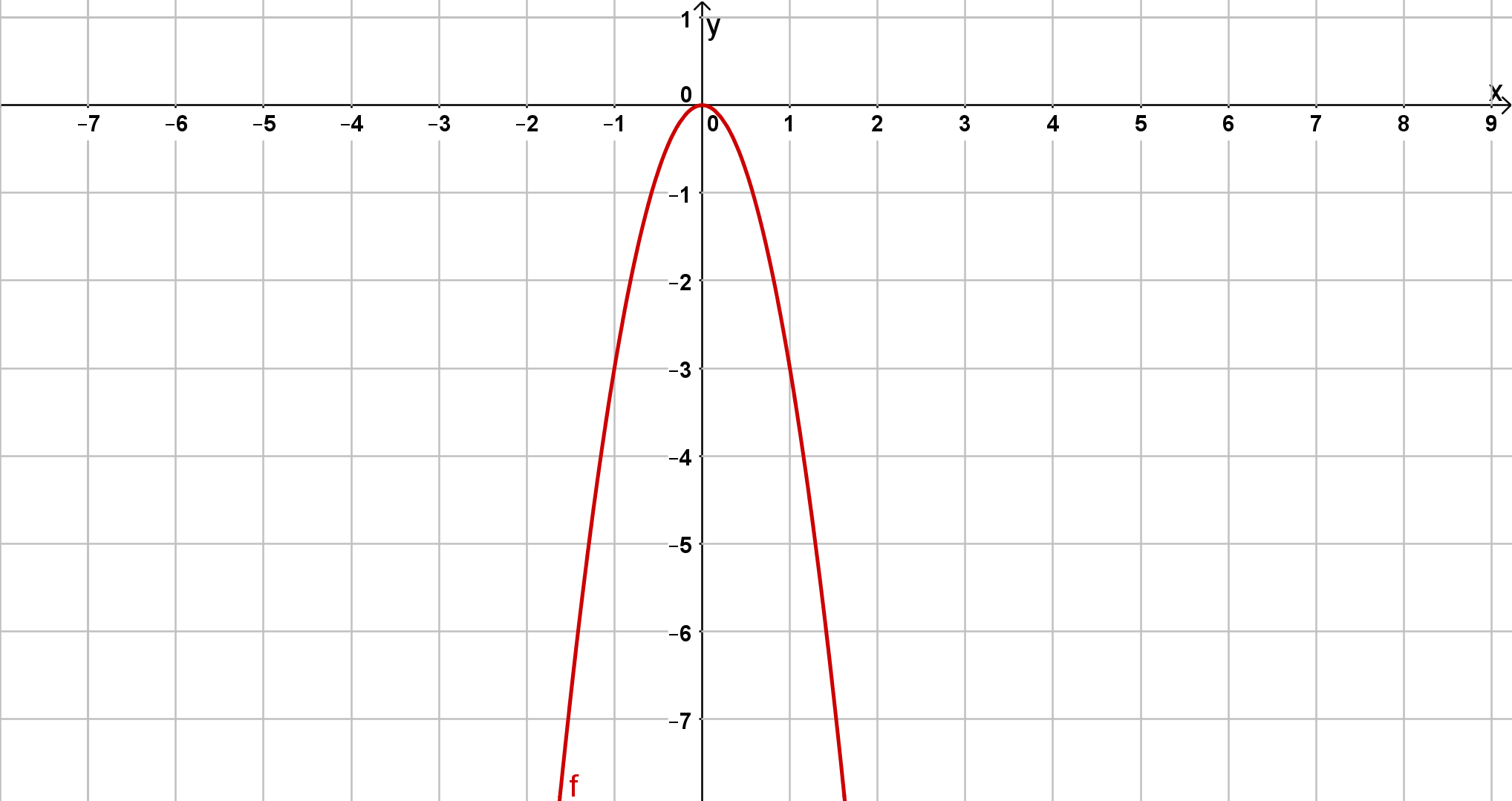
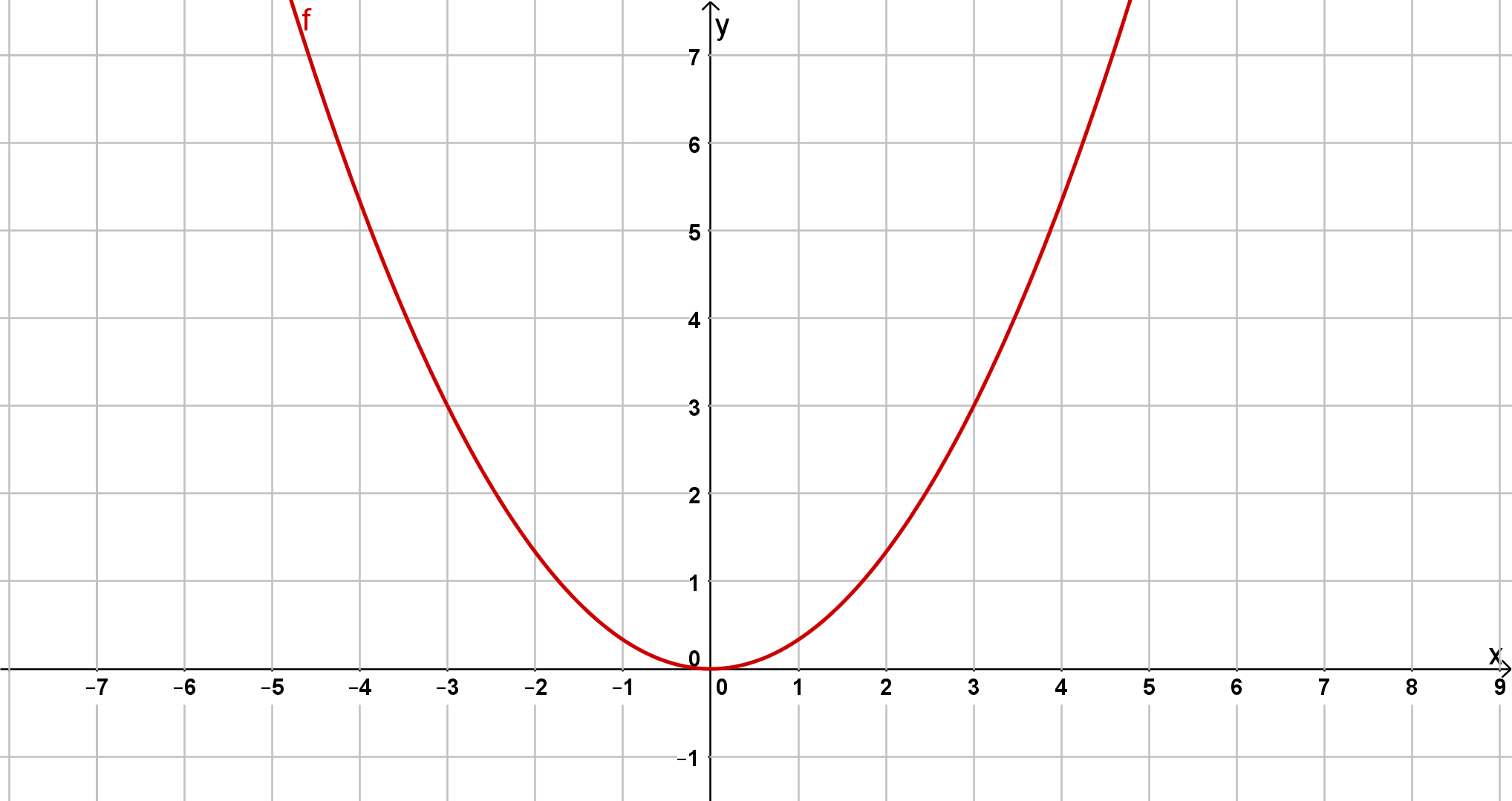
Zeichne die Graphen der folgenden Funktionen:
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Überlege dir, was macht das Minus vor dem x und was macht die 3 da. Das Minus öffnet die Parabel nach unten und die 3 macht die Parabel schmaler. Wenn du jetzt noch zwei x-Werte einsetzt kannst du die Funktion gut zeichnen.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Der Faktor ist kleiner als 1 und macht die Parabel damit breiter.
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Überlege dir, was die 2 für Auswirkungen auf die Funktion hat. Richtig, in diesem Fall steht das in für die Verschiebung entlang der y-Achse, hier in die negative Richtung wegen dem Minus. Ansonsten ist die Normalparabel.
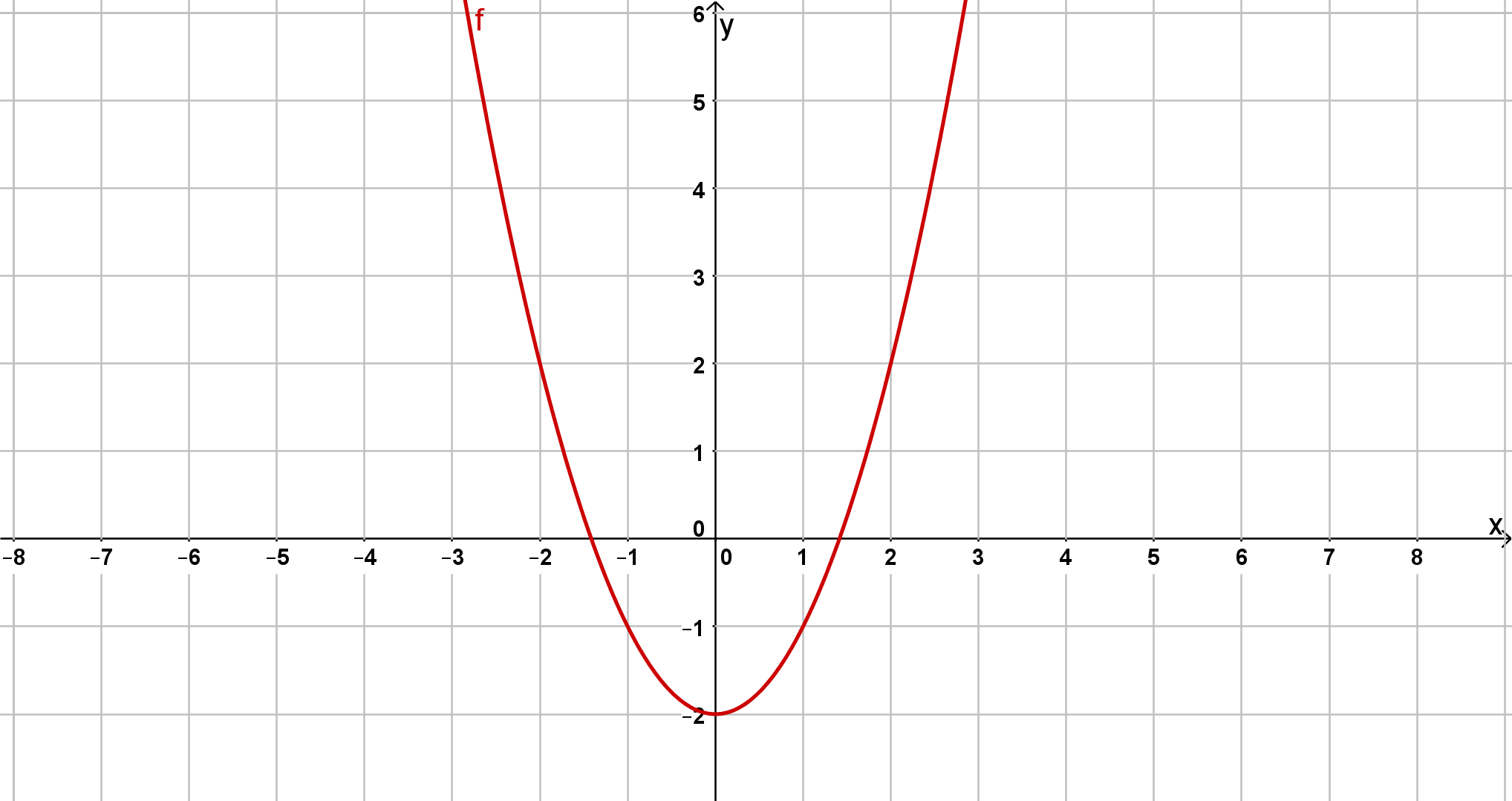
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Hier steht nur vor dem noch der Faktor . Dieser öffnet die Parabel weiter.
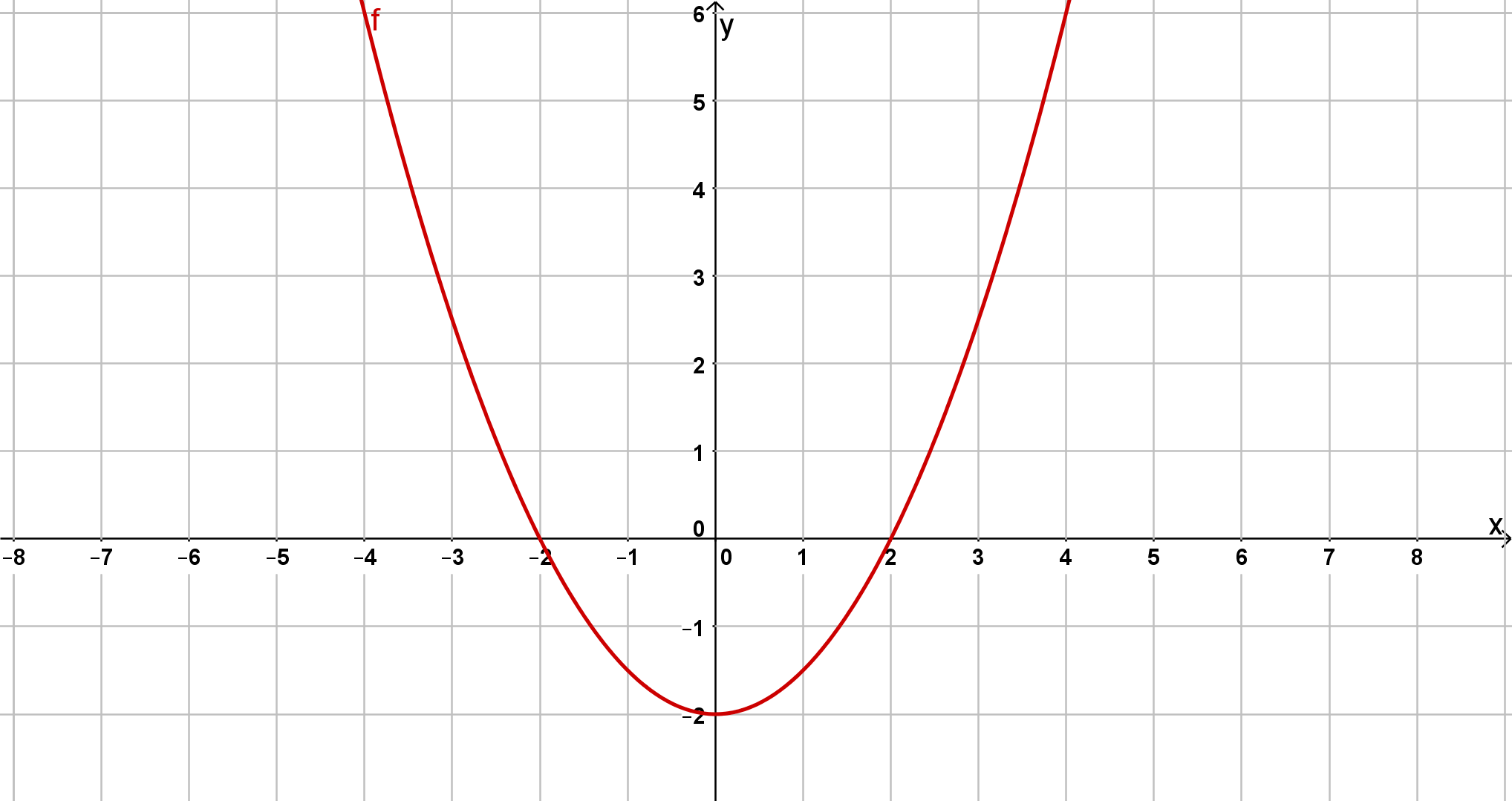
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Überlege dir, was das Minus vor dem macht. Außerdem haben wir eine Verschiebung um plus in die y-Richtung.
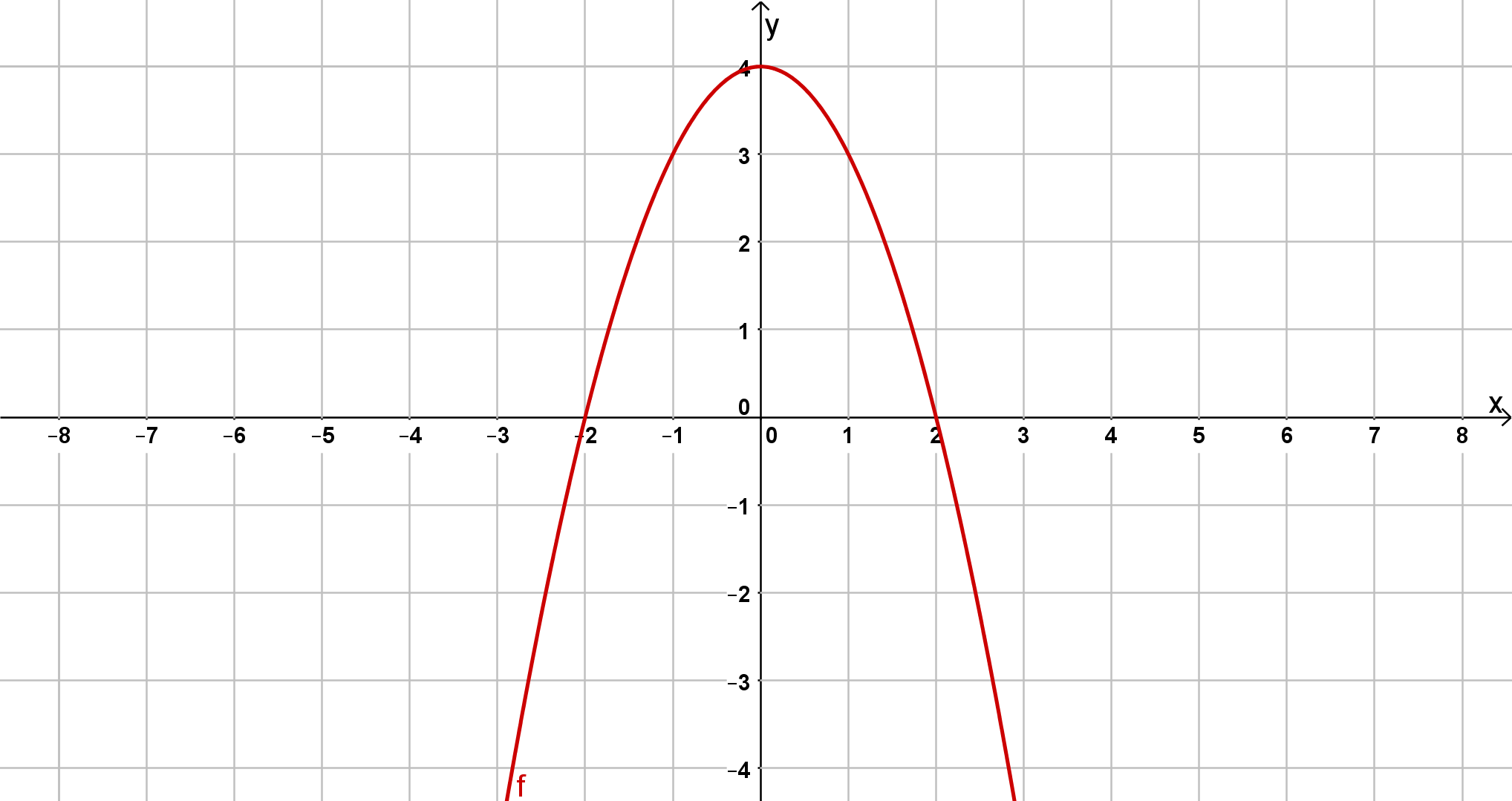
Hast du eine Frage oder Feedback?
Für diese Aufgabe benötigst Du folgendes Grundwissen: Parabel
Überlege dir, was die für Auswirkungen auf die Funktion hat.
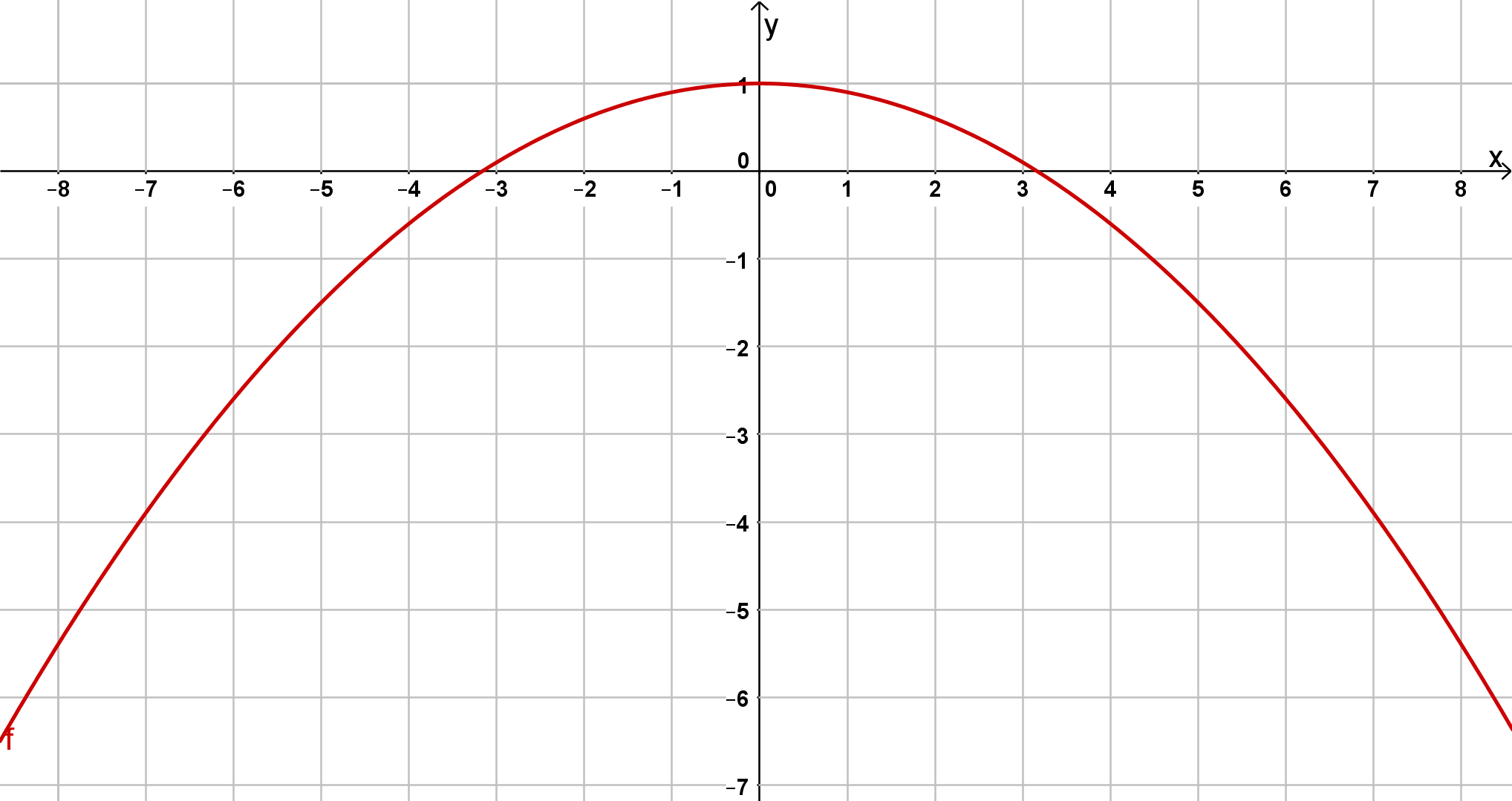
Hast du eine Frage oder Feedback?
- 4
Skizziere den Graphen der Funktion mit nur durch Überlegung und ohne Wertetabelle.
Für diese Aufgabe benötigst Du folgendes Grundwissen: Polynomfunktion
Zuerst wird die Funktion in die einzelnen Terme aufgeteilt.
Betrachte . Das Vorzeichen sagt dir, dass eine nach unten geöffnete Polynomfunktion vierten Grades vorliegt. Diese ist durch den Faktor 3 relativ schmal. Da hier der höchste Exponent der Funktion vorliegt, sieht die Funktion nach außen betrachtet aus, wie eine Funktion vierten Grades.
Betrachte . Das Vorzeichen sagt dir, dass eine nach oben geöffnete Parabel vorliegt, die durch den Faktor 2 ebenfalls etwas schmaler wird. Da hier der kleinste Exponent vorliegt, sieht die Funktion bei kleinen x-Werten, also in der Umgebung von Null, so aus wie eine Parabel.
Betrachte .
Hier liegt keine Verknüpfung mit einem vor, deswegen ist die 5 die Verschiebung auf der y-Achse, und zwar in die positive Richtung.
Es liegen also nur gerade Exponenten vor. Dies sagt dir, dass der Graph symmetrisch ist.
Die Terme wieder zusammen in der Funktion ergibt dann das:

Überlege dir zuerst, was die Vorzeichen für Auswirkungen auf die Funktion haben. Wo hast du Potenzen, welchen Grad haben diese? Welcher y-Achsen-Abschnitt liegt vor?
Dieses Werk steht unter der freien Lizenz
CC BY-SA 4.0 → Was bedeutet das?