1 Übersicht
Nullstellen und deren Berechnung sind in vielen Bereichen der Mathematik und anderen Wissenschaften von Bedeutung. In diesem Kurs lernst du, welche Strategien du anwenden kannst, um Nullstellen ausgewählter Funktionen bestimmen zu können.
Vorkenntnisse
Umformen von Gleichungen
Graphen zeichnen und auswerten
Grundkenntnisse über die verschiedenen Funktionstypen
Kursdauer:
ca. 3 Stunden
2 Definition von Nullstellen
Eine Nullstelle einer Funktion ist der -Wert eines Schnittpunktes vom Graphen von mit der x-Achse.
Das sind also gerade die -Werte, an denen ist.
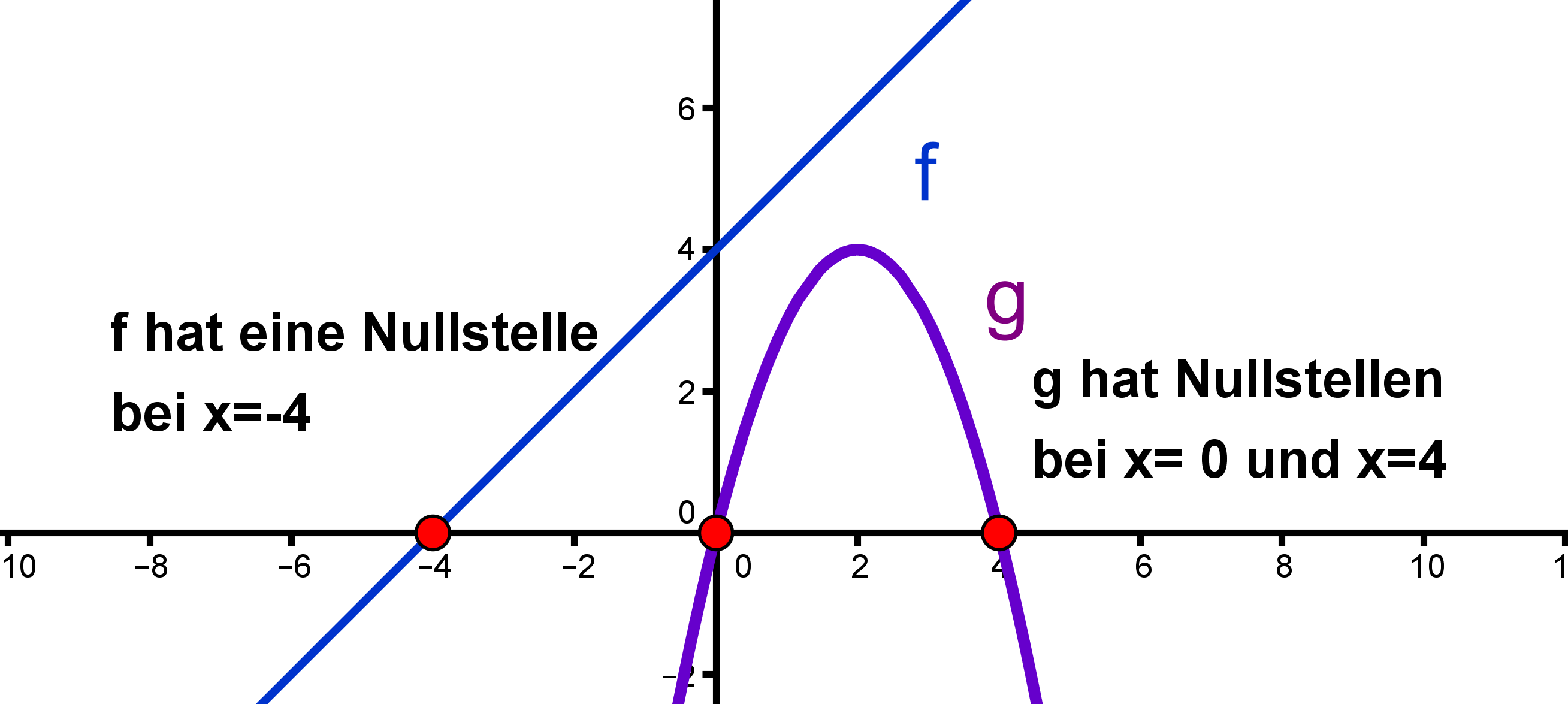
Hier sind die Nullstelle(n) der linearen Funktion f mit und der quadratischen Funktion g mit eingezeichnet.
Zur Berechnung der Nullstellen einer Funktion, brauchst du also Folgendes immer zu beachten:
3 Vorgehen bei der Nullstellenbestimmung
Wie du bei der Nullstellenberechnung vorgehst, hängt davon ab, welcher Funktionstyp vorliegt.
Handelt es sich um …
eine ganzrationale Funktion?
eine Wurzelfunktion?
eine Exponentialfunktion?
eine Logarithmusfunktion?
4 Nullstellen von ganzrationalen Funktionen
Eine ganzrationale Funktion ist eine Funktion der Form
Beispiele sind die Funktionen oder .
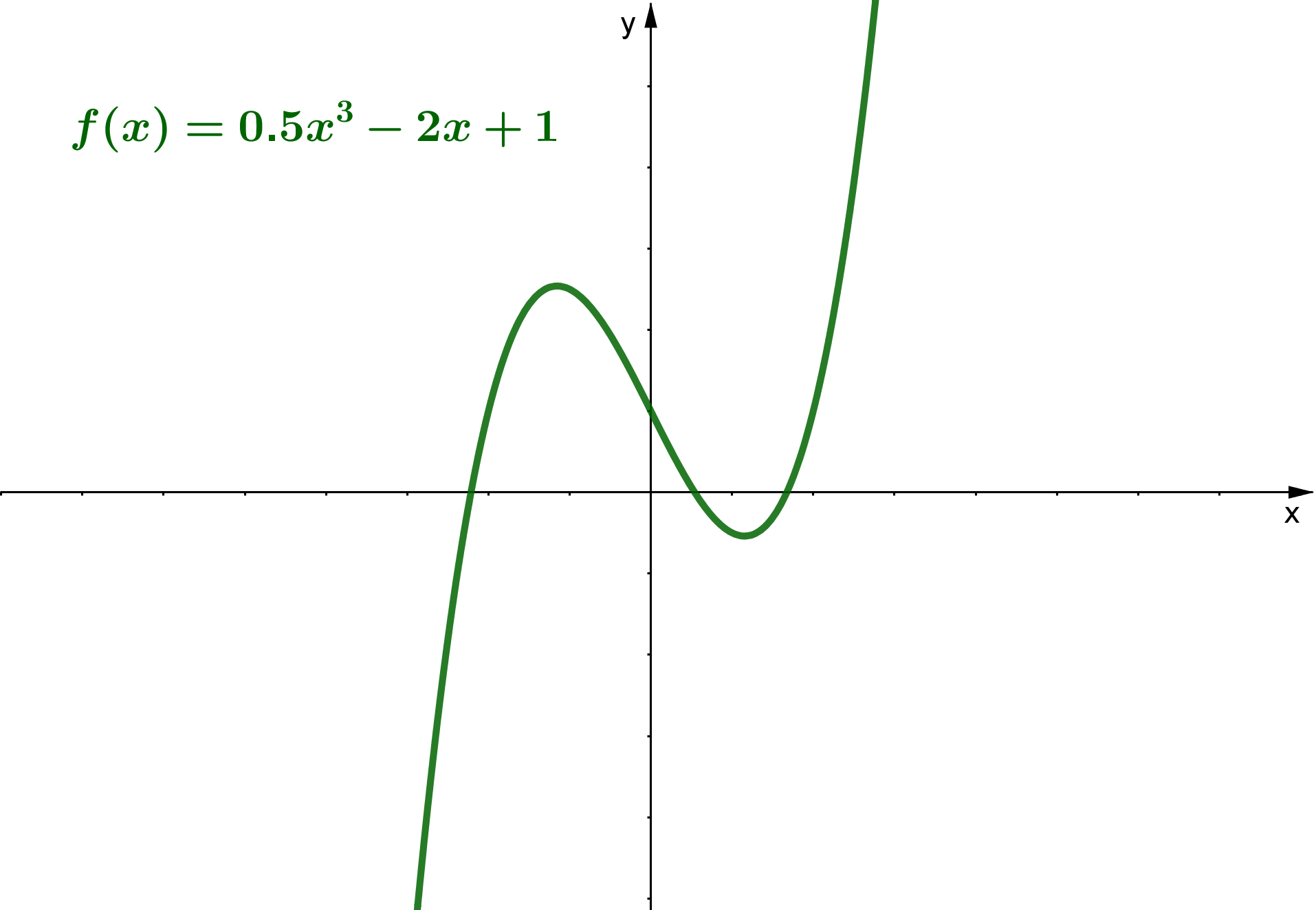
Wie du die Nullstellen einer Polynomfunktion berechnen kannst, hängt von der Form und vom Grad der Funktion ab.
Ist die Funktion in Linearfaktordarstellung, kannst du die Nullstellen sofort ablesen. Du musst nur betrachten, für welche Zahlen die einzelnen Faktoren Null werden.
Ist nur ein Teil der Funktion in Linearfaktoren zerlegt, musst du die Nullstellen der einzelnen Faktoren teilweise mit anderen Mitteln bestimmen wie z.B. der quadratischen Lösungsformel.
Handelt es sich um eine Summe aus einer Potenzfunktion und einer Konstanten, dann bringe die Konstante auf die andere Seite des Gleichheitszeichens und ziehe die Wurzel.
Bei einer quadratische Funktion kannst du die Nullstellen mithilfe der quadratischen Lösungsformel oder mit dem Satz von Vieta bestimmen.
Handelt es sich um eine Polynomfunktion vom Grad , gibt es unterschiedliche Vorgehensweisen bei der Nullstellenbestimmung:
kleinste Potenz von ausklammern
Eine ausführliche Erklärung zur Nullstellenberechnung bei ganzrationalen Funktionen findest du in dem Kurs: Berechnungsmethoden - Nullstellen von Polynomfunktionen.
5 Nullstellen von gebrochenrationalen Funktionen
Eine gebrochenrationale Funktion ist eine Funktion, die sich als Bruch von Polynomen darstellen lässt. Gebrochenrationale Funktionen sind also von der Form , wobei sowohl als auch Polynome sind.
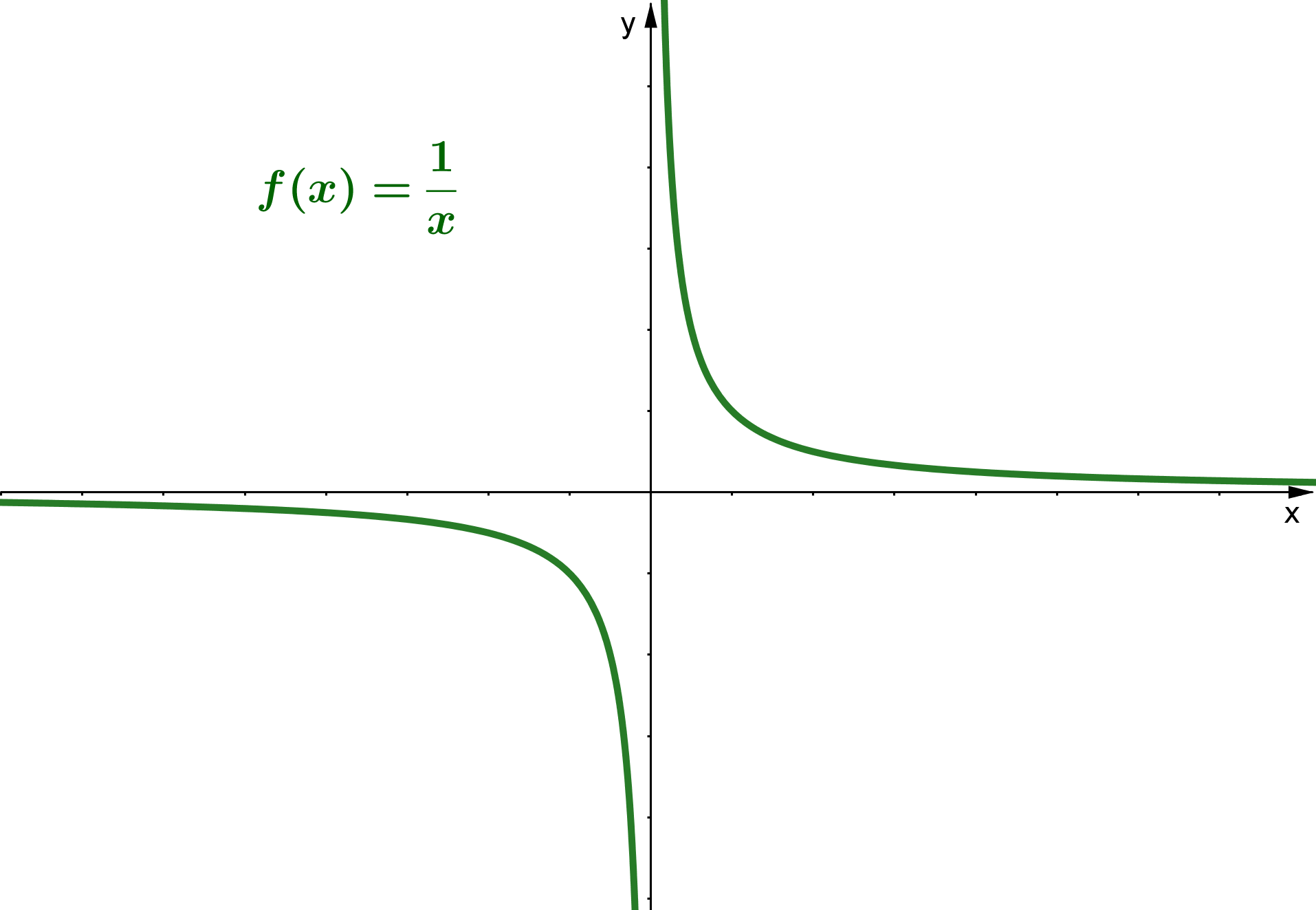
Eine gebrochenrationale Funktion wird genau dann Null, wenn das Zählerpolynom ist und das Nennerpolynom an der ermittelten Nullstelle ist.
Beispiel
Sowohl das Zählerpolynom als auch das Nennerpolynom hat eine Nullstelle unter anderem bei . An dieser Stelle nimmt die gebrochenrationale Funktion den Wert an.
Berechnung der Nullstellen
Um die Nullstellen von zu berechnen, brauchst du also nur das Polynom zu setzen. Du musst aber danach prüfen, dass für das Nennerpolynom an der ermittelten Nullstelle gilt. Die Nullstellen von kannst du auf die gleiche Weise bestimmen, wie es auf der Kursseite Nullstellen von ganzrationalen Funktionen beschrieben wird.
Dabei muss eine beliebige Nullstellen auch im Definitionsbereich der Funktion liegen, also .
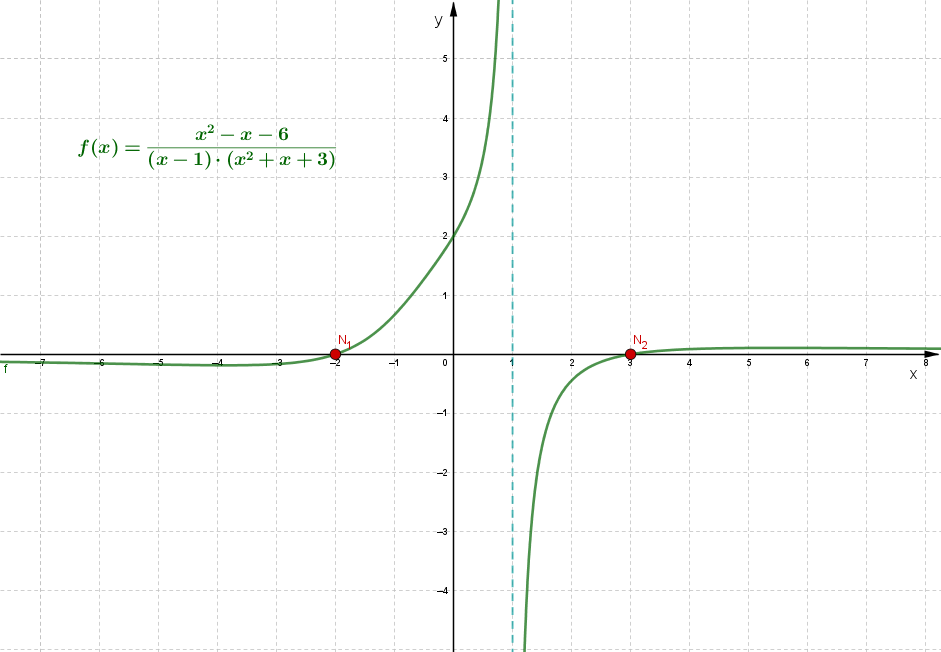
Beispiel
| ↓ | Berechne die möglichen Nullstellen von . Setze dazu . | ||
| ↓ | Mitternachtsformel anwenden. | ||
Überprüfe nun, ob das Nennerpolynom an den ermittelten Nullstellen nicht auch Null wird.
, und .
Überprüfe nun, ob die Nullstellen im Definitionsbereich der Funktion liegen, indem du die Definitionsmenge bestimmst. Setze dazu das Nennerpolynom gleich Null und berechne die Nullstellen von .
Aus dem Linearfaktor kannst du die Nullstelle von ablesen. Überprüfe auf weitere Nullstellen. Setze dazu die zweite Klammer gleich Null.
| ↓ | Mitternachtsformel anwenden. | ||
Da die Diskriminante , besitzt keine weiteren Nullstellen. Bestimme die Definitionsmenge .
Da und , hat zwei Nullstellen bei , .
6 Nullstellen von Wurzelfunktionen
Wurzelfunktionen sind Potenzfunktionen in der Form, dass die Variable unter einer Wurzel steht, also . Sie bilden damit die Umkehrfunktionen zu Potenzfuktionen der Form mit .
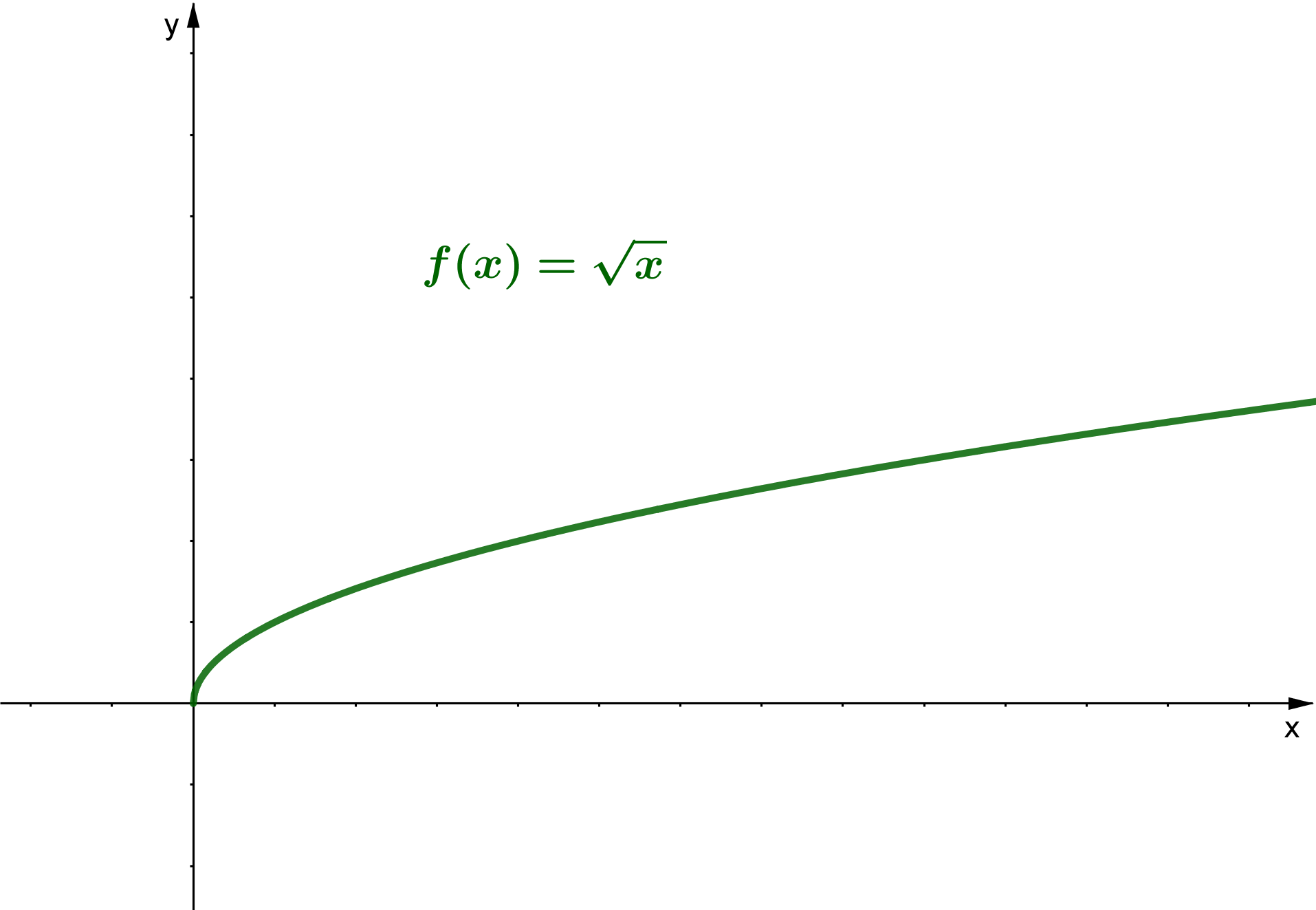
Unter der Wurzel muss nicht nur eine Potenz stehen, sondern die Wurzelfunktion kann auch von der Form sein, bei der der Radikand eine beliebige Funktion ist.
Eine Wurzelfunktion nimmt den Wert Null genau dann an, wenn der Radikand Null ist.
Beispiele
a)
Setze den Radikanden gleich Null.
Die Funktion hat drei Nullstellen bei , , .
b)
Setze den Radikanden gleich Null.
hat somit dieselben Nullstellen wie eine Sinusfunktion der Form .
Auf den folgenden Kursseiten erfährst du, wie du diese bestimmen kannst.
7 Nullstellen von trigonometrischen Funktionen (1|5)
Die Winkelfunktionen Sinus, Kosinus und Tangens sind die wichtigsten trigonometrischen Funktionen. Sie tauchen sowohl am rechtwinkligen Dreieck, als auch in der Kreisgeometrie (Trigonometrie am Einheitskreis) auf.
Durch die Form ihrer Graphen spielen sie auch eine wichtige Rolle bei der mathematischen Beschreibung von Wellen und Schwingungen.
Trigonometrische Funktionen sind periodisch, d. h. es gibt nicht nur eine oder zwei Nullstellen, sondern entweder unendlich viele oder gar keine.
Trigonometrische Funktionen haben unendlich viele oder gar keine Nullstellen.
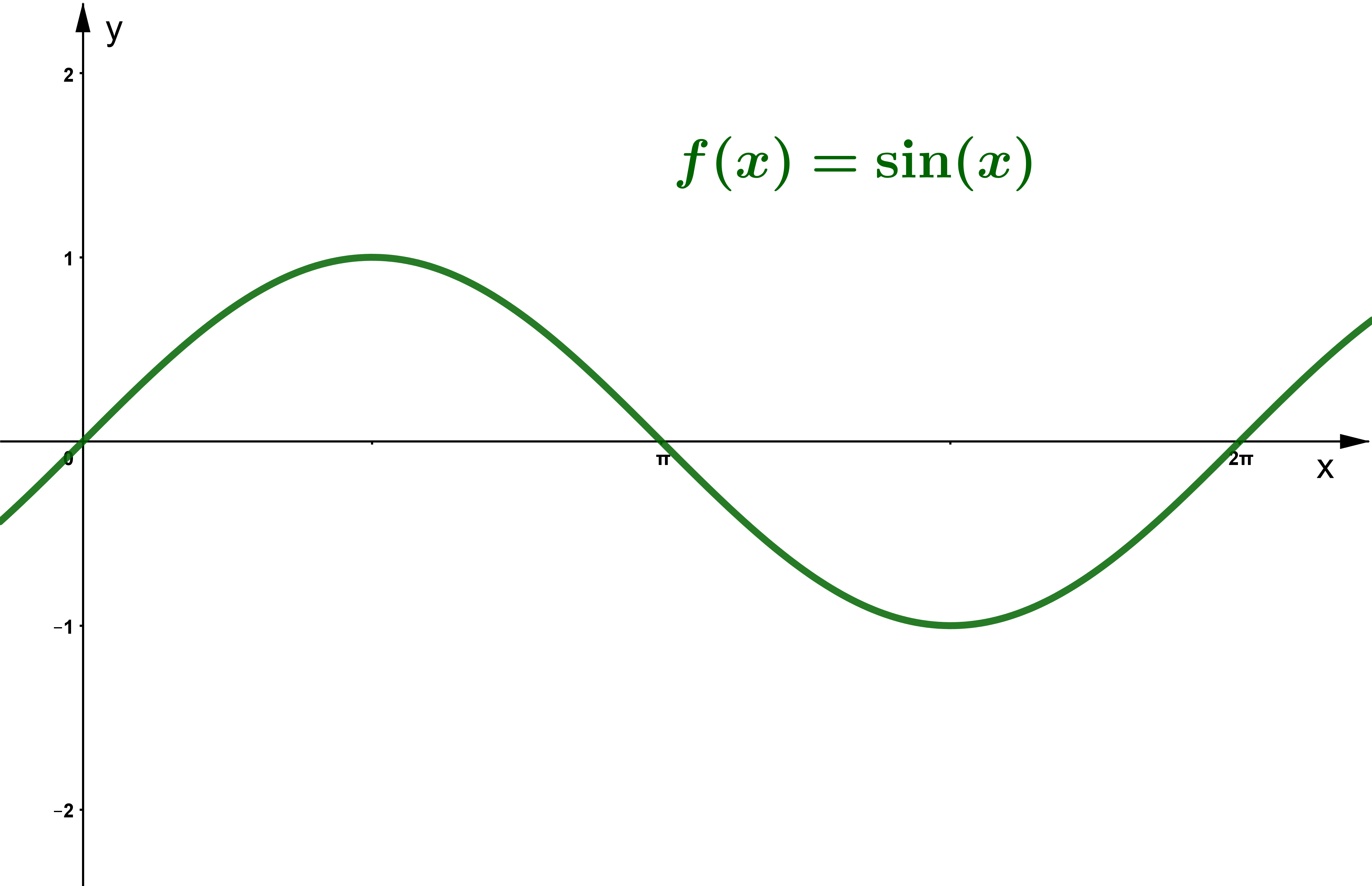
Sinus
Für welche wird Null?
Am Einheitskreis kannst du erkennen, für welche Null wird.
Es gilt: , also für jedes beliebige .
Aus dem Einheitskreis kann man ablesen, dass bzw. und bzw. Nullstellen im Intervall bzw. sind.
Da die Sinusfunktion aber periodisch ist, hat sie unendlich viele Nullstellen.
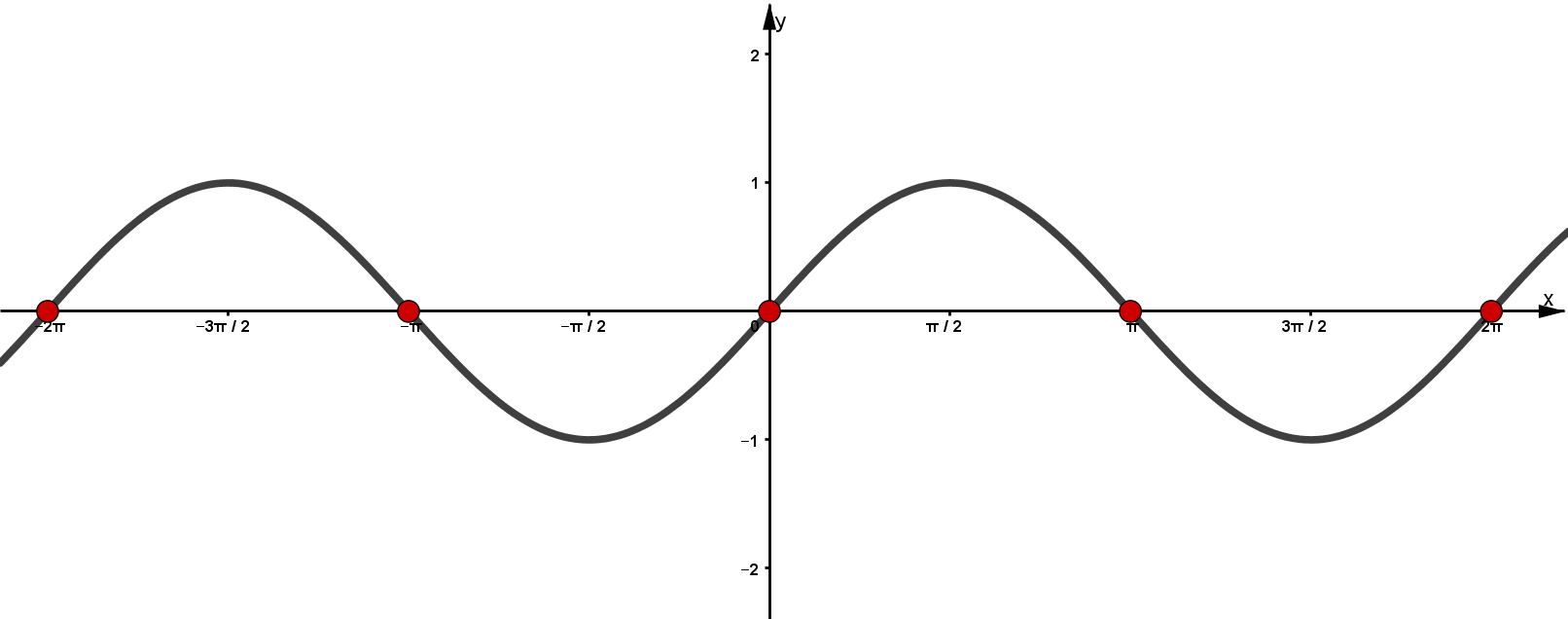
Wir wissen, dass der Sinus an ganzzahligen Vielfachen von Null wird. Es gilt also: mit .
Die Nullstellenmenge für lautet somit: .
8 Nullstellen von trigonometrischen Funktionen (2|5)
Betrachten wir nun eine Sinusfunktion der Form mit , bei der das Argument eine beliebige Funktion ist.
Da , brauchst du bei der Nullstellenbestimmung also nur zu setzen. Wir wissen, dass beim Sinus gilt: mit . Somit gilt zur Nullstellenbestimmung:
Löse diese Gleichung nach auf, um die Nullstellen von zu erhalten.
Fazit
Um Nullstellen der Sinusfunktion zu bestimmen, muss man prüfen, wann ihr Argument ein Vielfaches von wird.
Beispiel
Man weiß, dass mit . Setze das Argument der Sinusfunktion also gleich und löse nach auf.
Stelle das Ergebnis mit einer Nullstellenmenge dar.
9 Nullstellen von trigonometrischen Funktionen (3|5)
Als Nächstes schauen wir uns den Cosinus an.
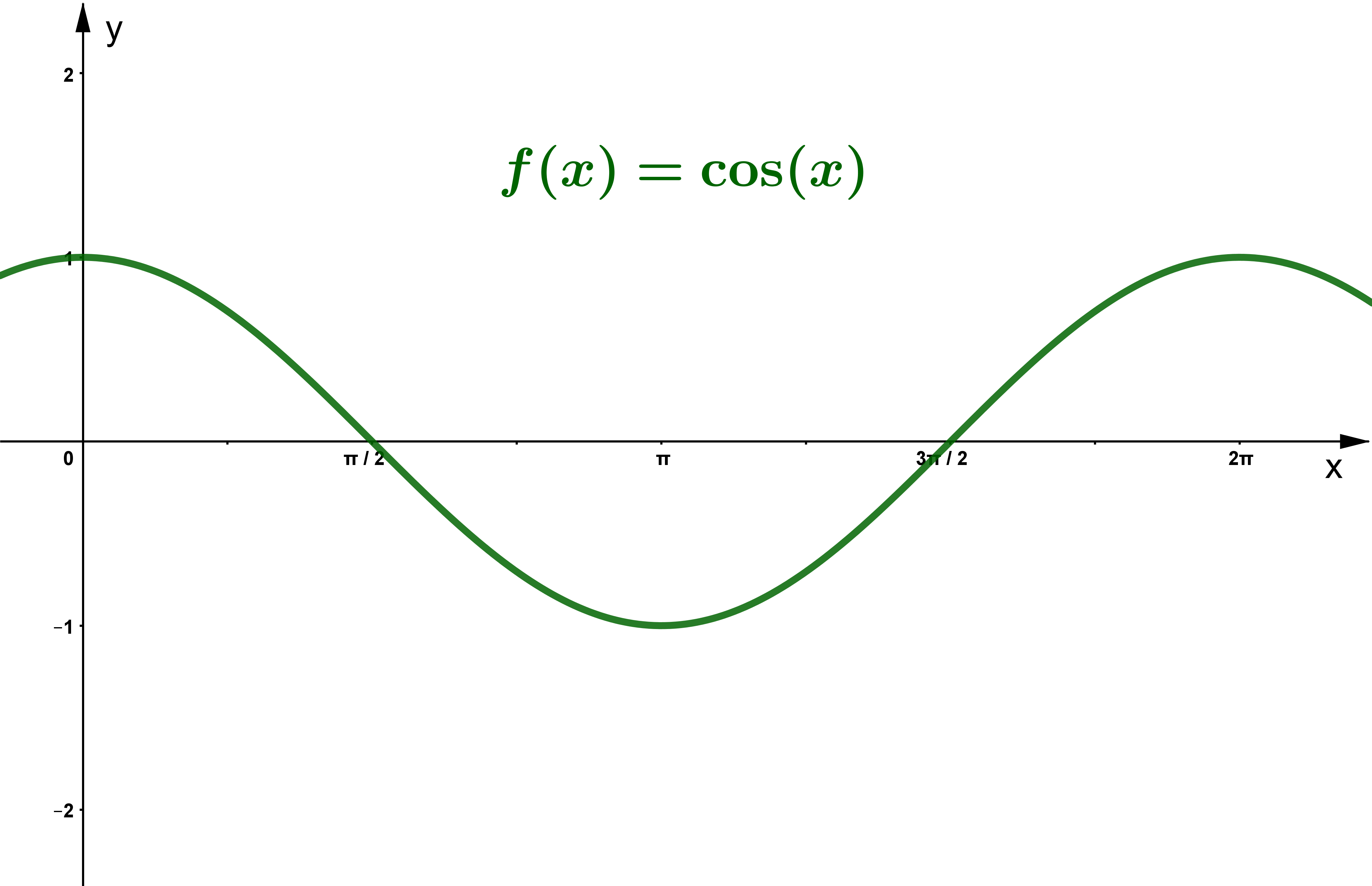
Cosinus
Für welche wird Null?
Am Einheitskreis kannst du erkennen, für welche Null wird.
Es gilt: , also für jedes beliebige .
Aus dem Einheitskreis kann man ablesen, dass bzw. und bzw. Nullstellen im Intervall bzw. sind.
Da die Cosinusfunktion aber periodisch ist, hat sie unendlich viele Nullstellen.
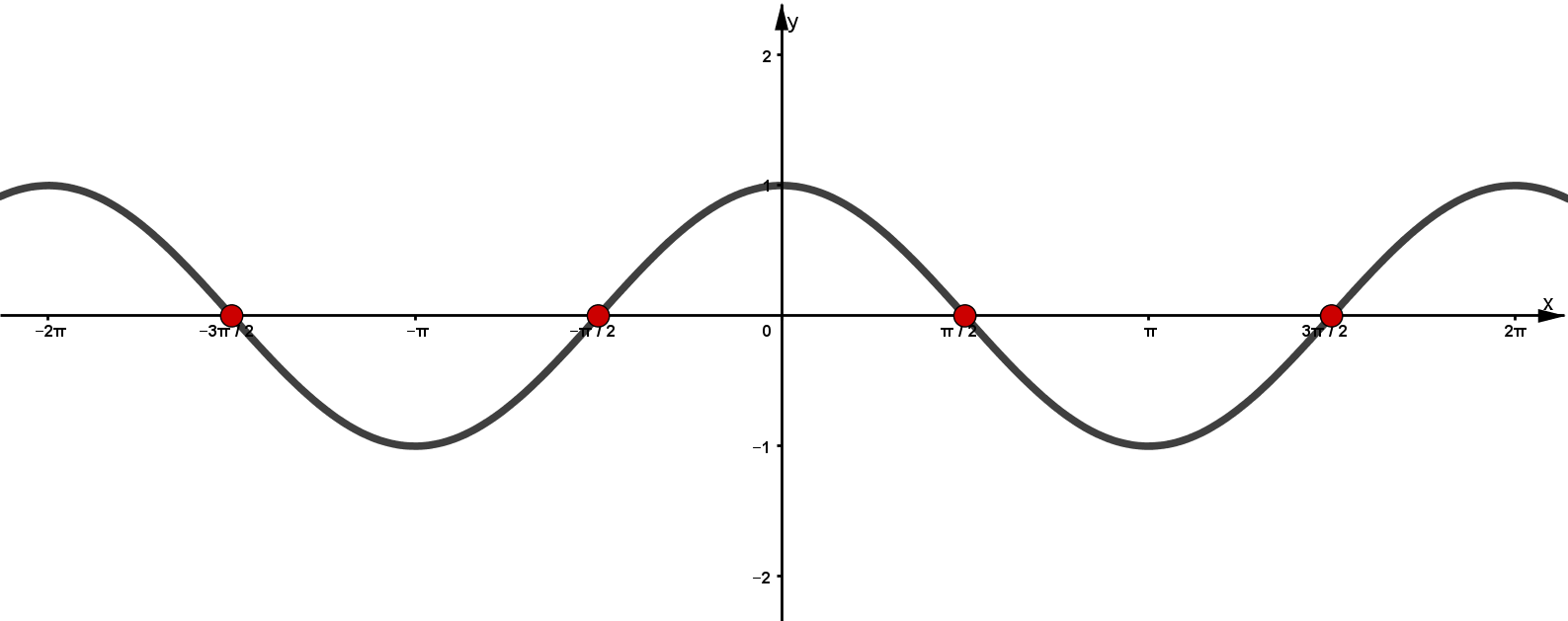
Wir wissen, dass der Cosinus an ungeradzahligen Vielfachen von Null wird. Es gilt also mit . Gerade Zahlen sind ein Vielfaches von . Ungerade Zahlen kann man beschreiben, indem man von einer geraden Zahl abzieht. Es gilt also mit .
Die Nullstellenmenge für lautet somit: .
10 Nullstellen von trigonometrischen Funktionen (4|5)
Betrachten wir nun eine Cosinusfunktion der Form mit , bei der das Argument eine beliebige Funktion ist.
Da , brauchst du bei der Nullstellenbestimmung also nur zu setzen. Wir wissen, dass beim Cosinus gilt: mit . Somit gilt zur Nullstellenbestimmung:
Löse diese Gleichung nach auf, um die Nullstellen von zu erhalten.
Fazit
Um die Nullstellen der Cosinusfunktion zu bestimmen, muss man prüfen, wann ihr Argument ein ungerades Vielfaches von wird.
Beispiel
Man weiß, dass mit .Setze das Argument der Cosinusfunktion also gleich und löse nach auf.
Stelle das Ergebnis mit einer Nullstellenmenge dar.
Da eine beliebige ganze Zahl ist, kann der Radikand sowohl positive, als auch negative Werte annehmen. Für einen negativen Radikanden gibt es allerdings keine reelle Lösung. Dass das Ergebnis dennoch stimmt, hat damit zu tun, dass die Funktion reelle und komplexe Nullstellen hat.
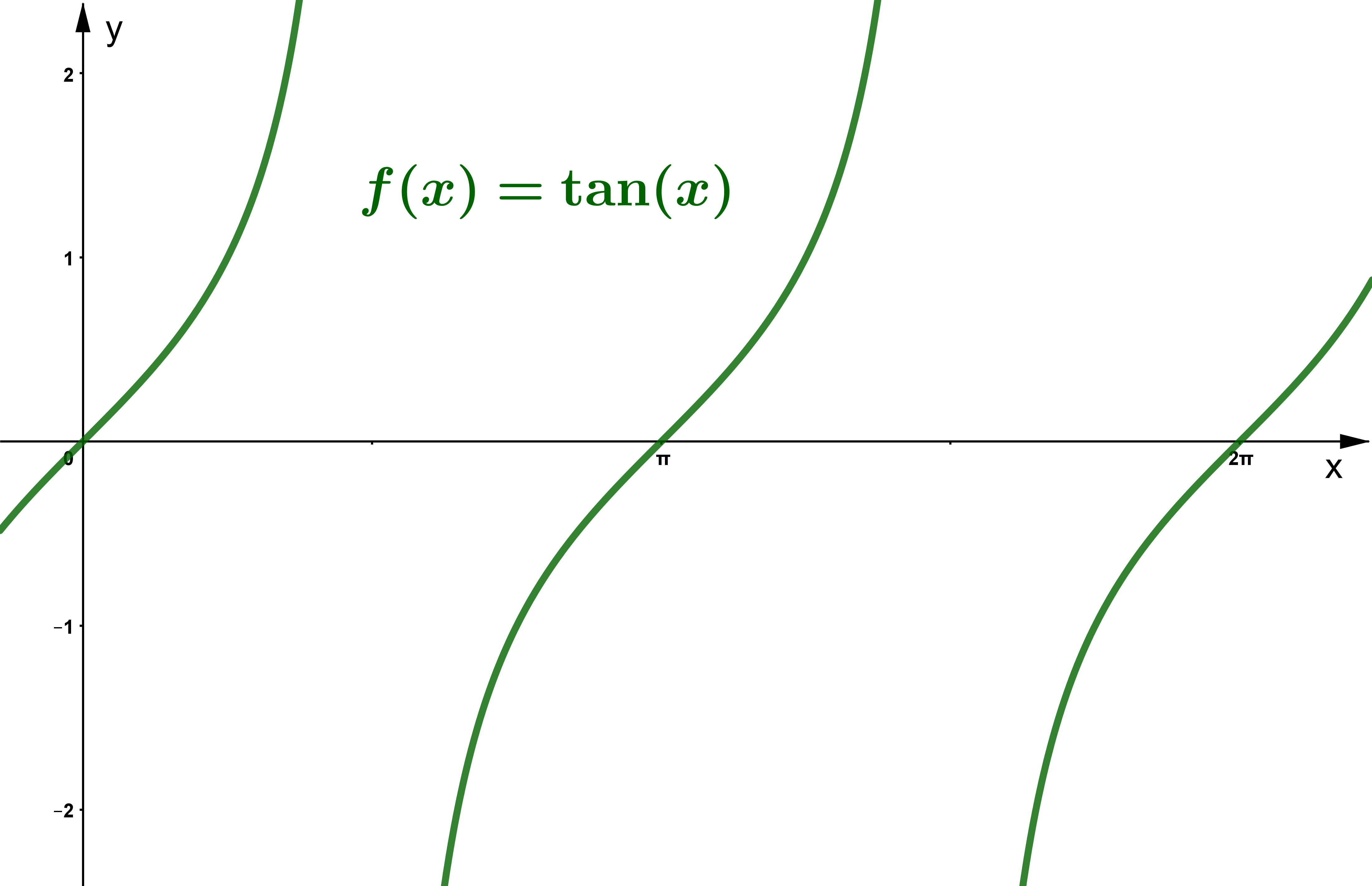
11 Nullstellen von trigonometrischen Funktionen (5|5)
Als Nächstes schauen wir uns noch den Tangens an.
Tangens
Für welche wird Null?
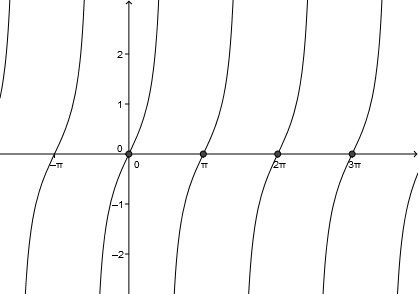
Die Tangensfunktion ist definiert als .
Der Tangens wird genau dann Null, wenn der Zähler gleich Null ist. Also .
Somit hat die Tangensfunktion, ebenso wie die Sinusfunktion, Nullstellen bei allen ganzzahligen Vielfachen von .
mit
Fazit
Um Nullstellen der Tangensfunktion zu bestimmen, muss man prüfen, wann ihr Argument ein Vielfaches von wird.
12 Nullstellen von Exponentialfunktionen
Eine Exponentialfunktion hat den Funktionsterm . Dabei ist und .
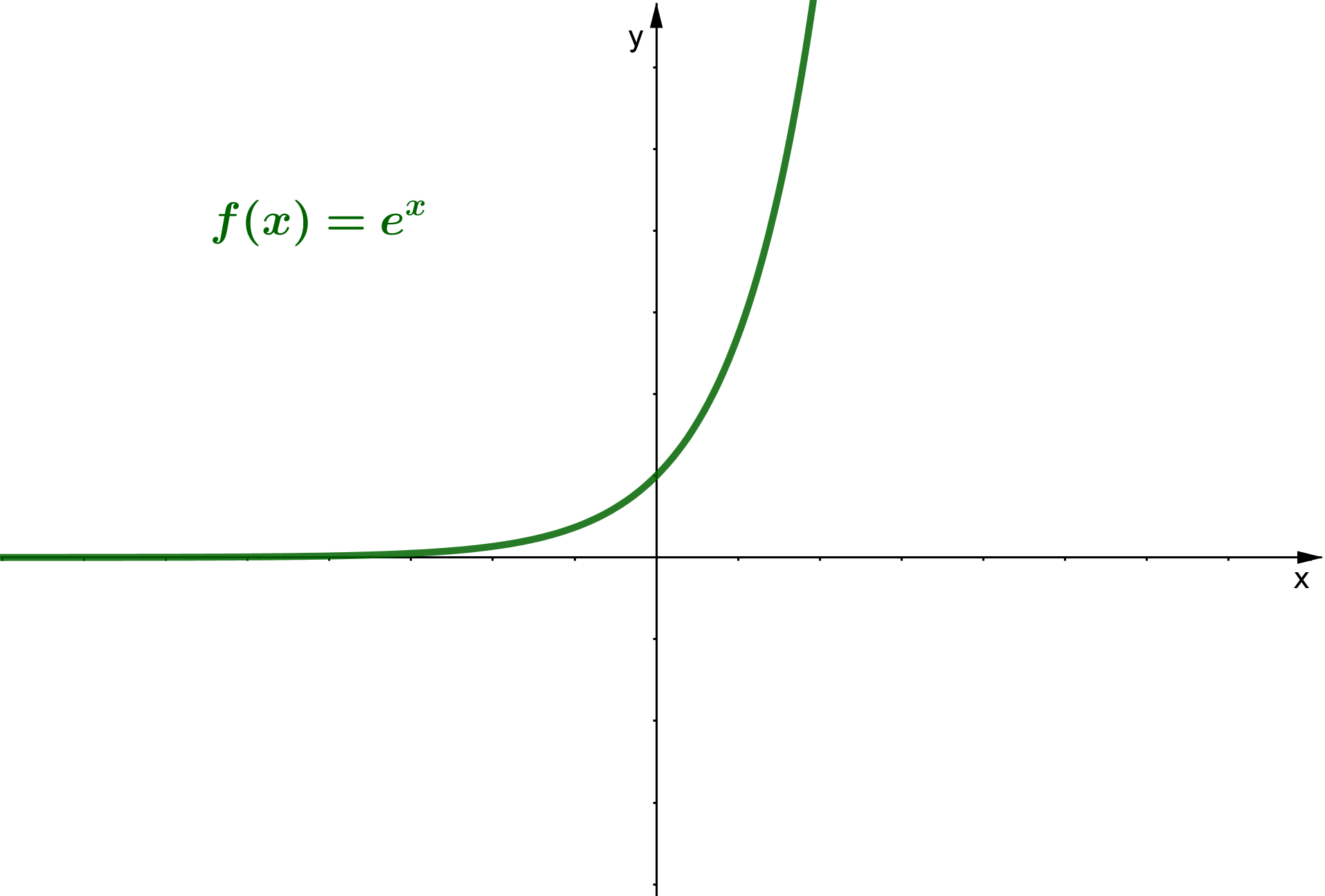
Da , muss zur Bestimmung der Nullstelle gelten: . Jedoch gilt: . Daraus folgt:
Ausnahme
Wird eine Exponentialfunktion durch eine Konstante in -Richtung verschoben, kann es eine Nullstelle geben.
Da ist, muss für das Vorhandensein einer Nullstelle gelten:
wenn , dann .
wenn , dann .
Du kannst dir das noch nicht so gut vorstellen? Im folgenden Applet kannst du , und mit den Schiebereglern verändern und schauen, wie sich der Graph der Exponentialfunktion verhält.
13 Nullstellen von Logarithmusfunktionen
Eine Logarithmusfunktion ist eine Abbildung mit der Funktionsvorschrift
, wobei und gilt.
heißt Basis des Logarithmus.
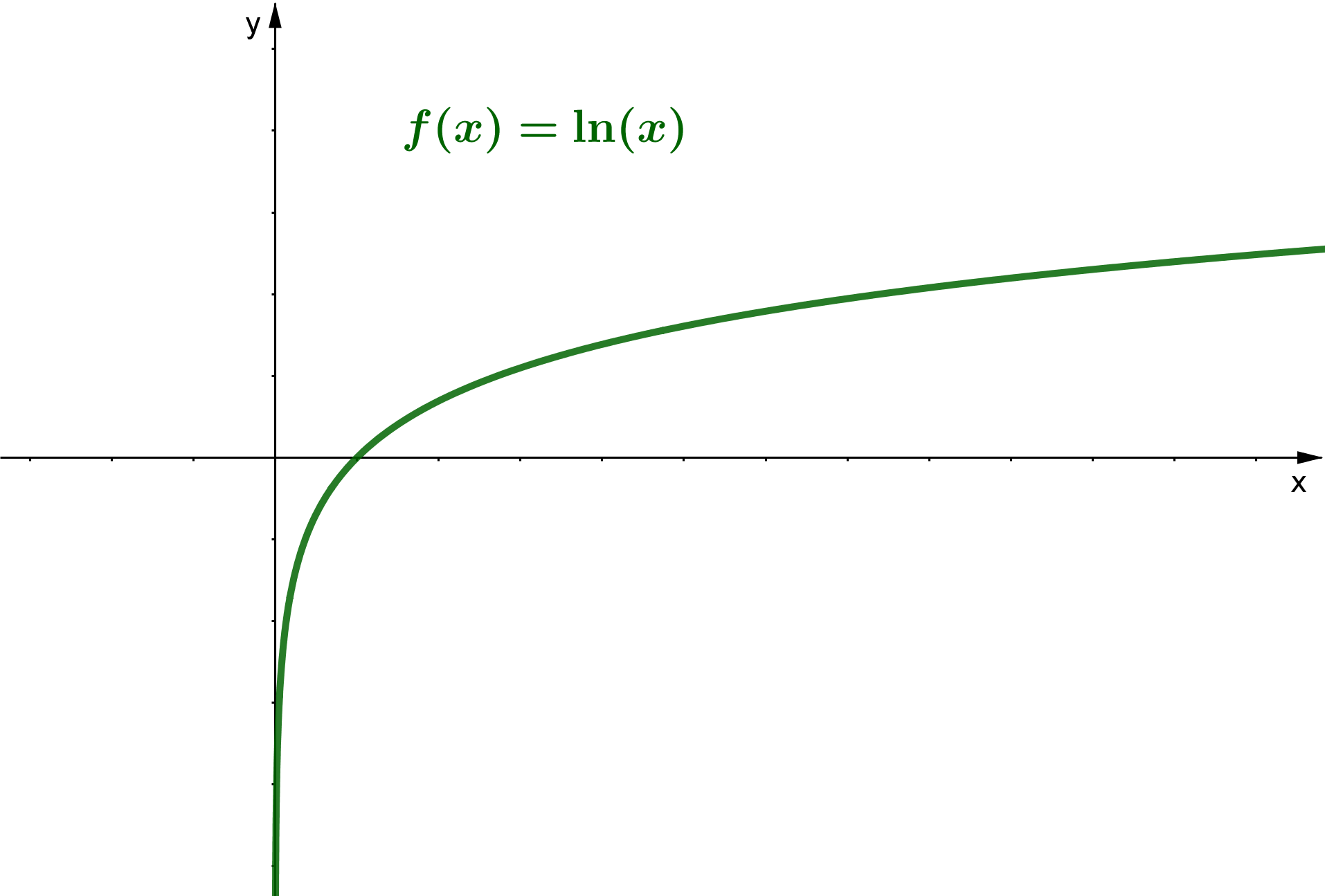
Betrachte eine beliebige Logarithmusfunktion . Setze zur Bestimmung der Nullstellen die Funktion gleich Null:
Da die Logarithmusfunktion die Umkehrfunktion einer Exponentialfunktion der Form ist, gilt: .
Daraus folgt für die Nullstelle:
Eine Logarithmusfunktion der Form hat die Nullstelle bei .
Die Logarithmusfunktion kann auch von der Form sein, bei der das Argument eine beliebige Funktion ist.
Allgemein gilt also:
Eine Logarithmusfunktion nimmt genau dann den Wert Null an, wenn ihr Argument 1 ist.
Beispiele
Setze das Argument gleich Eins und löse die Gleichung.
| ↓ | Mitternachtsformel anwenden. | ||
Die Funktion hat zwei Nullstellen bei , .
Setze das Argument gleich Eins und löse die Gleichung.
14 Aufgaben zur Nullstellenberechnung
Berechne die Nullstelle(n) der folgenden Funktionen.
a)
Laden
b)
Laden
c)
Laden
d)
Laden
e)
Laden
f)
Laden
g)
Laden
h)
Laden
i)
Laden
Feedback
Damit wir unseren Kurs für die Zukunft verbessern können, würden wir uns sehr über ein Feedback von dir freuen. Es dauert auch nur 3 Minuten!
Klicke dazu auf diesen Link.
